Display Title
Math Example--Measures of Central Tendency--Range: Example 26
Display Title
Math Example--Measures of Central Tendency--Range: Example 26
Topic
Measures of Central Tendency
Description
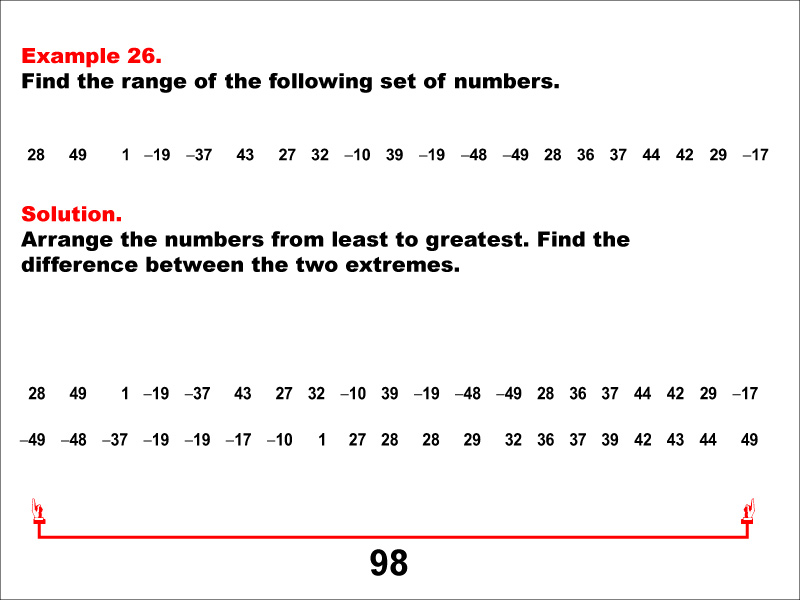
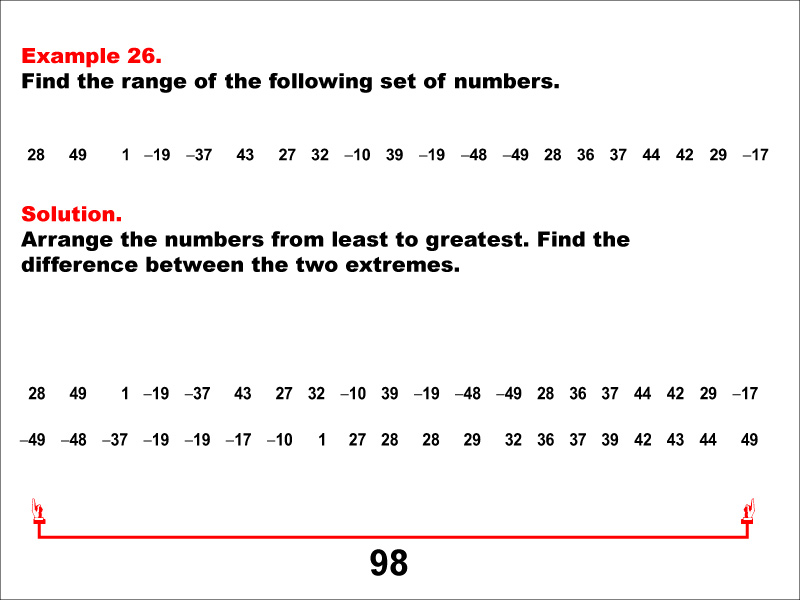
This example illustrates how to find the range of the following set of numbers: 28, 49, 1, -19, -37, 43, 27, 32, -10, 39, -19, -48, -49, 28, 36, 37, 44, 42, 29, -17. The solution involves arranging the numbers from least to greatest and finding the difference between the two extremes. The range is calculated to be 98. This example is particularly valuable as it includes a mix of positive and negative values, as well as repeated numbers, helping students understand how to handle diverse and complex datasets when calculating the range.
In the broader context of measures of central tendency, examples like this are crucial for developing a comprehensive understanding of data analysis. The range provides insight into the spread of data, complementing other measures such as mean, median, and mode. By working through multiple examples with varying types of numbers and repetitions, students can better grasp how these measures work together to provide a complete picture of a dataset's distribution.
Teacher's Script: Now, let's take a closer look at this example. We'll start by organizing our numbers from smallest to largest, paying special attention to the negative values and repeated numbers. Then, we'll identify the minimum and maximum values to calculate the range. Remember, when working with negative numbers, we use the absolute value of the difference. This process will help us understand how the range represents the spread of our data, especially when dealing with a mix of positive and negative numbers. Can you think of any limitations of using the range as a measure of spread in this case? How might other measures of central tendency or spread provide additional insights into this dataset?
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Range Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.7.SP.B.4, CCSS.MATH.CONTENT.6.SP.B.5.C |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2014 |
| Keywords | data analysis, tutorials, measures of central tendency, range |