Display Title
Math Example--Measures of Central Tendency--Sample Mean--Example 5
Display Title
Math Example--Measures of Central Tendency--Sample Mean--Example 5

Topic
Measures of Central Tendency
Description
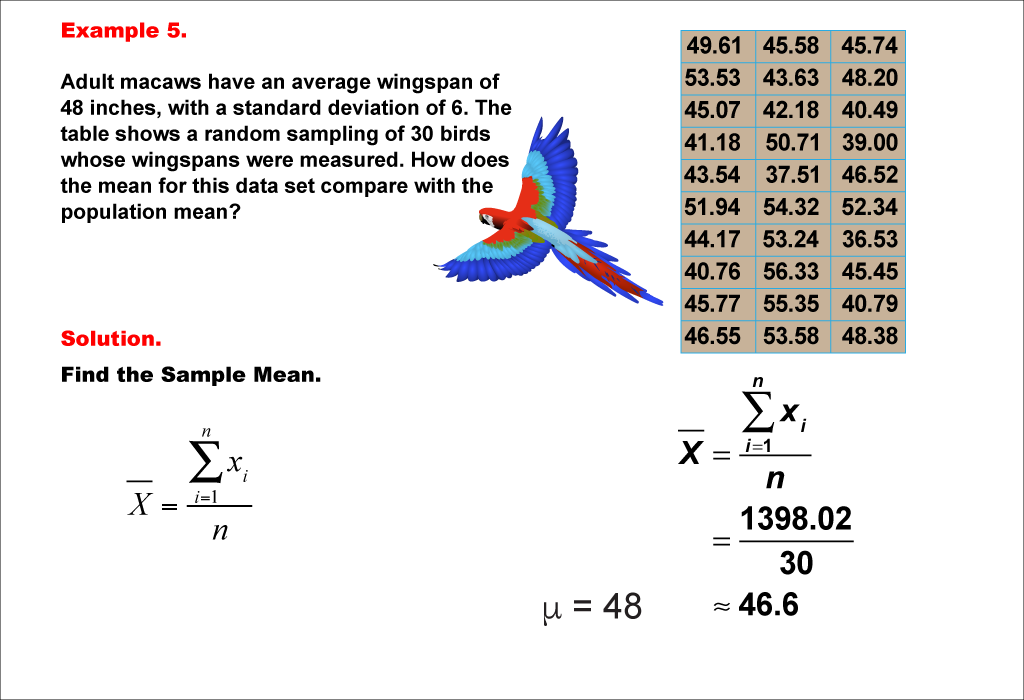
This example illustrates the calculation of the sample mean for another group of 30 macaws. The image displays a table showing the wingspans of these macaws, along with the formula for calculating the sample mean. The population of adult macaws has an average wingspan of 48 inches with a standard deviation of 6. The sample mean is calculated by summing all the wingspans (1398.02 inches) and dividing by the number of macaws (30), resulting in a sample mean of 46.6 inches.
Measures of central tendency are essential in statistics, offering a way to describe the typical or central value in a dataset. This collection of examples supports teaching this topic by presenting various scenarios where the sample mean is calculated and compared to the known population mean. By using different samples from the same population (macaws) and other populations (trout, elephants, sequoia trees), students can observe how sample means may fluctuate but generally approximate the population mean.
Providing multiple worked-out examples is vital for students to fully grasp the concept of sample mean. Each example reinforces the calculation process while showcasing how sample means can differ from the population mean and from other samples of the same population. This repetition helps students develop confidence in their ability to calculate and interpret sample means across diverse situations, and understand the natural variability in sampling.
Teacher's Script: Now, let's look at our fifth example of calculating a sample mean. We have another set of data on the wingspans of 30 macaws. How does this example compare to our previous macaw example? Let's calculate this new sample mean together. How does it compare to our previous sample mean and the population mean for macaws? What might explain these differences? What does this tell us about the importance of not relying on a single sample?
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Sample Mean Collection.
| Common Core Standards | CCSS.MATH.CONTENT.7.SP.A.2, CCSS.MATH.CONTENT.HSS.IC.B.4, CCSS.MATH.CONTENT.HSS.IC.B.5 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2021 |
| Keywords | mean, measures of central tendency, sample mean |