Display Title
Math Example--Measures of Central Tendency--Weighted Mean--Example 2
Display Title
Math Example--Measures of Central Tendency--Weighted Mean--Example 2
Topic
Measures of Central Tendency
Description
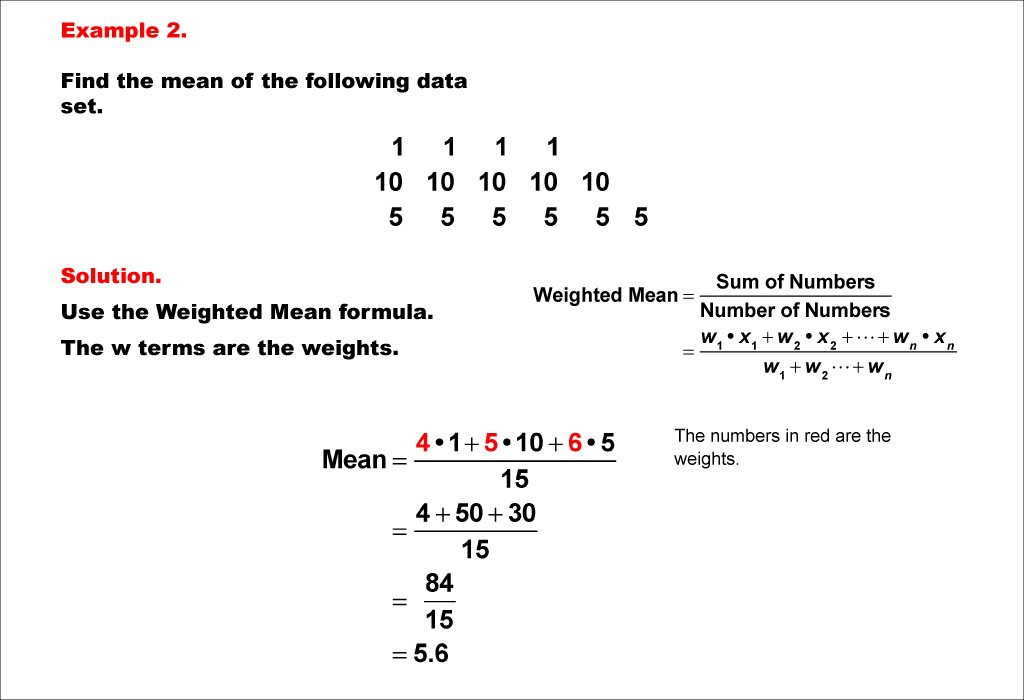
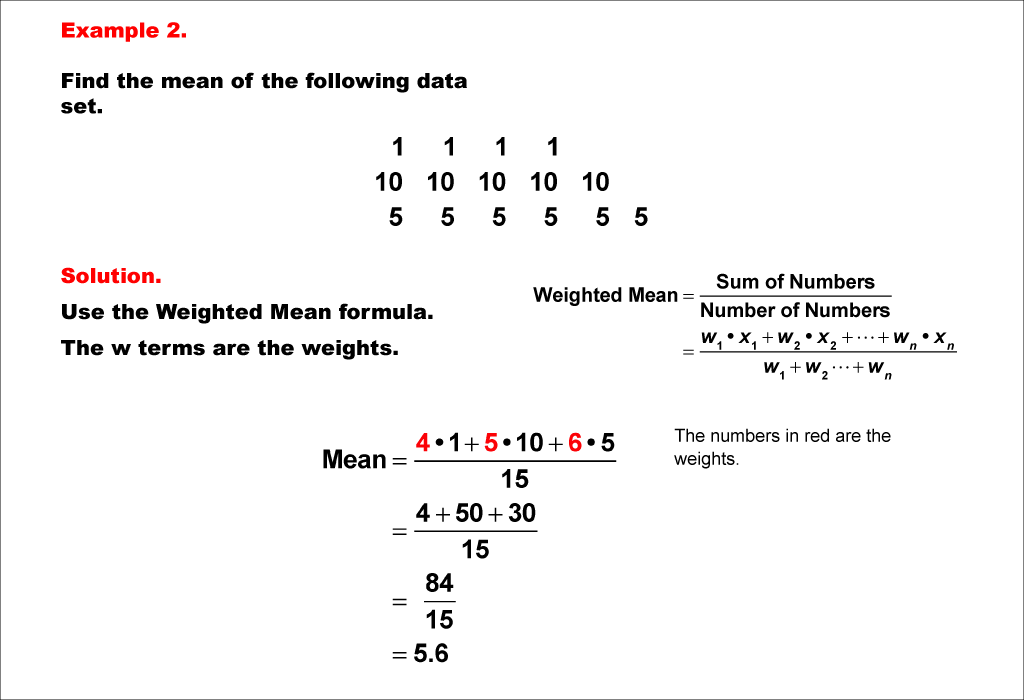
This example illustrates the calculation of a weighted mean for a data set containing the values 1, 10, and 5, with weights of 4, 5, and 6 respectively. The weighted mean is computed using the formula: (4 * 1 + 5 * 10 + 6 * 5) / (4 + 5 + 6), resulting in a final answer of 5.6.
Weighted mean is an important concept in measures of central tendency, allowing for the consideration of the relative importance or frequency of each data point. This collection of examples helps teach this topic by providing diverse scenarios and data sets, enabling students to practice and understand the application of the weighted mean formula in various contexts.
Presenting multiple worked-out examples is crucial for students to fully comprehend the concept of weighted mean. By observing the formula applied to different situations, students can develop a deeper understanding of how weights influence the final average and how to interpret the results in real-world scenarios.
Teacher's Script: In this example, we're calculating another weighted mean. Notice how the weights affect the final result. What do you think would happen if we changed the weights? How would that impact our average?
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Weighted Mean Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.5.C |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2021 |
| Keywords | mean, measures of central tendency |