Display Title
Math Example--Percents--Equations with Percents: Example 12
Display Title
Math Example--Percents--Equations with Percents: Example 12
Topic
Solving Equations
Description
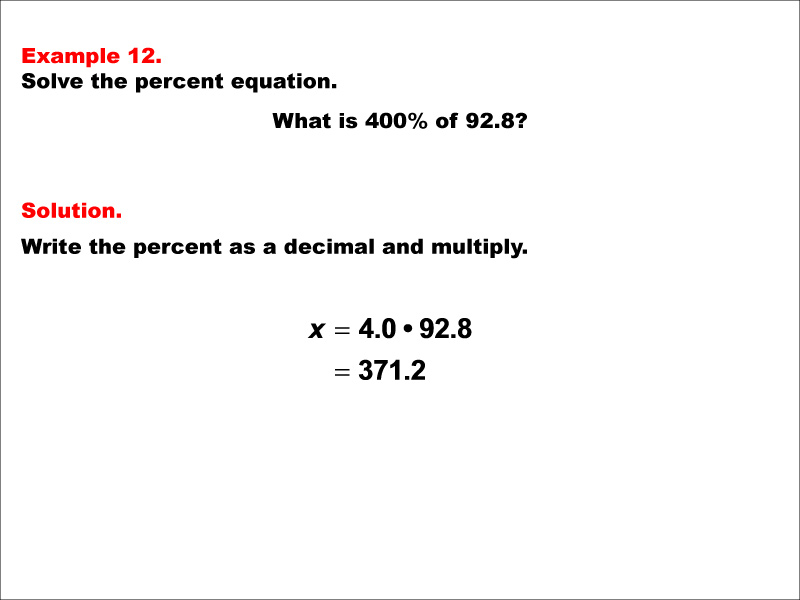
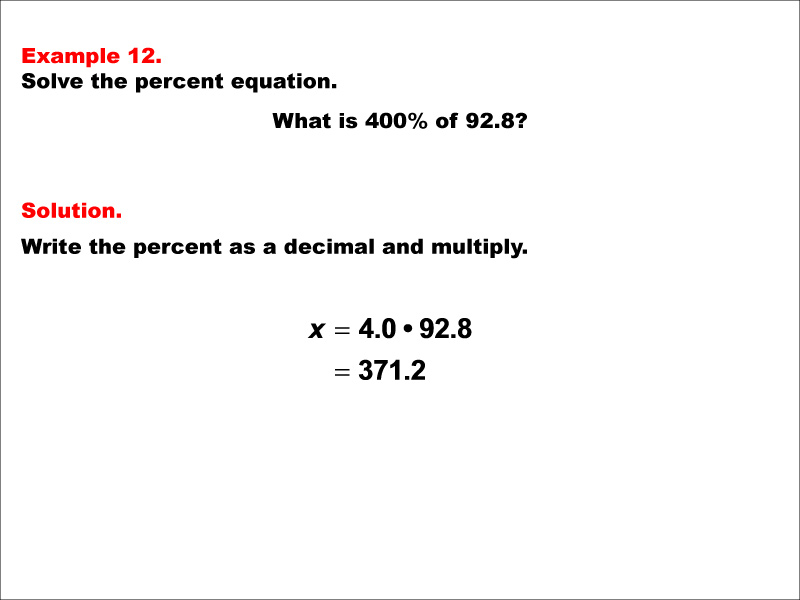
This math example demonstrates solving percent equations by asking "What is 400% of 92.8?" The solution involves converting 400% to its decimal equivalent, 4.0, and then multiplying it by 92.8 to obtain the result of 371.2. This example showcases how to handle percentages greater than 100% and their application to decimal numbers, illustrating the versatility of the percent-to-decimal conversion method in complex scenarios.
Solving equations with percents is a fundamental skill in mathematics that has wide-ranging applications in finance, statistics, and scientific analysis. These examples help students understand the process of converting percentages to decimals and applying them to various numbers, including cases where the percentage represents a multiple of the original value. This skill is crucial for more advanced mathematical concepts and real-world problem-solving scenarios, such as calculating compound growth rates, analyzing market trends, or understanding rapid increases in scientific phenomena.
The importance of presenting multiple worked-out examples cannot be overstated. Each new example reinforces the concept while introducing different percentage values and base numbers. This approach allows students to recognize patterns, adapt their problem-solving strategies, and build confidence in handling diverse percentage-based calculations. By practicing with percentages above 100% and decimal base numbers, students develop a more robust understanding of how percentages relate to real-world quantities and prepare for more complex mathematical challenges they may encounter in higher education or professional settings.
Teacher Script: "Let's tackle this interesting example together. We need to find 400% of 92.8. First, what does 400% mean in terms of the original value? That's right, it's four times the original. So, how do we convert 400% to a decimal? Correct, it becomes 4.0. Now, we multiply this by our base number, 92.8. Can anyone calculate 4.0 × 92.8 for us? Excellent, it's 371.2. Notice how we're using the same method, but with a percentage that represents multiplying the original value by 4. This shows that our approach works consistently, even when dealing with percentages above 100%. In real-world situations, you might encounter scenarios where something quadruples or even increases more, so being comfortable with these calculations is crucial for analyzing growth or increase scenarios in various fields."
For a complete collection of math examples related to Solving Equations click on this link: Math Examples: Equations with Percents Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.EE.B.5, CCSS.MATH.CONTENT.7.RP.A.3, CCSS.MATH.CONTENT.6.RP.A.3.C |
|---|---|
| Grade Range | 5 - 8 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Percent Equations |
| Copyright Year | 2013 |
| Keywords | Percent, equation, solution, solving equation, percentage |