
Display Title
Math Example--Percents--Equations with Percents: Example 14
Display Title
Math Example--Percents--Equations with Percents: Example 14
Topic
Solving Equations
Description
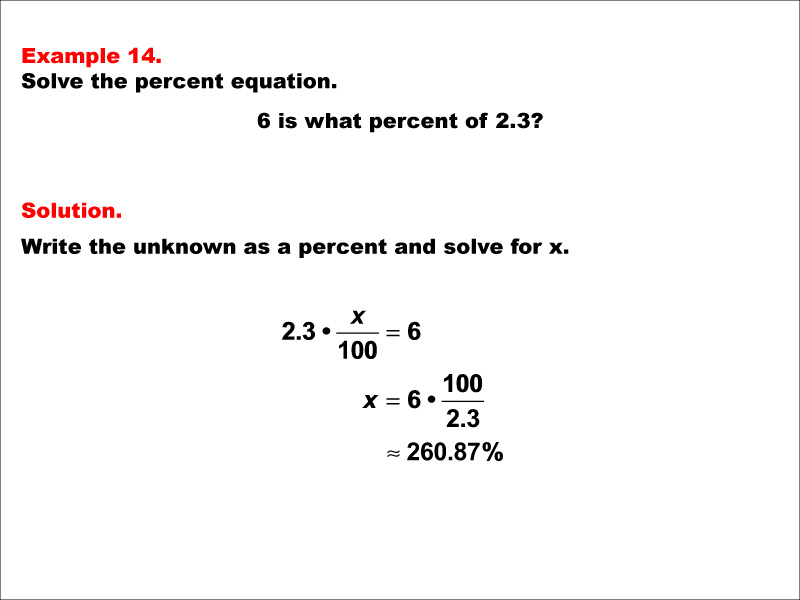
This math example demonstrates solving percent equations by asking "6 is what percent of 2.3?" The solution involves setting up the equation 2.3 * (x / 100) = 6, then solving for x to get x = 6 * (100 / 2.3), which is approximately 260.87%. This example introduces a scenario where the resulting percentage is greater than 100% and involves a decimal base number.
Solving equations with percents is a fundamental skill in mathematics that has wide-ranging applications in finance, statistics, and data analysis. These examples help students understand how to set up and solve equations involving percentages, especially in cases where the result exceeds 100%. This skill is crucial for more advanced mathematical concepts and real-world problem-solving scenarios, such as calculating percentage increases, analyzing growth rates, or understanding relative values in various contexts.
The importance of presenting multiple worked-out examples cannot be overstated. Each new example reinforces the concept while introducing different scenarios, including those with decimal numbers and percentages above 100%. This approach helps students recognize patterns, adapt their problem-solving strategies, and gain confidence in their ability to handle diverse percentage-based calculations. By practicing with various value pairs, including those that result in percentages greater than 100%, students develop a more comprehensive understanding of how percentages relate different quantities and prepare for more complex mathematical challenges they may encounter in higher education or professional settings.
Teacher Script: "Let's tackle this interesting percent problem. We're asked, '6 is what percent of 2.3?' To solve this, we set up the equation 2.3 * (x / 100) = 6. Now, how do we solve for x? We multiply both sides by 100/2.3. This gives us x = 6 * (100 / 2.3), which is approximately 260.87%. Notice that our result is greater than 100%. This means that 6 is more than twice 2.3. In real-world scenarios, you might encounter situations where one value is significantly larger than another, resulting in percentages above 100%. For example, if a company's profits more than doubled, we might say they increased by over 200%. Understanding these concepts is crucial for analyzing growth, comparing values, and interpreting data in various fields."
For a complete collection of math examples related to Solving Equations click on this link: Math Examples: Equations with Percents Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.EE.B.5, CCSS.MATH.CONTENT.7.RP.A.3, CCSS.MATH.CONTENT.6.RP.A.3.C |
|---|---|
| Grade Range | 5 - 8 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Percent Equations |
| Copyright Year | 2013 |
| Keywords | Percent, equation, solution, solving equation, percentage |