
Display Title
Math Example--Percents--Equations with Percents: Example 21
Display Title
Math Example--Percents--Equations with Percents: Example 21
Topic
Solving Equations
Description
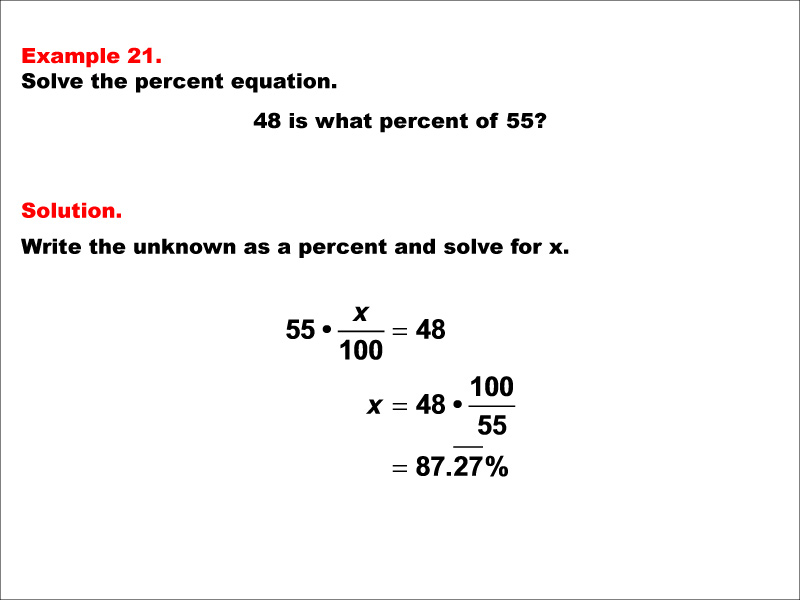
This math example focuses on solving percent equations by asking "48 is what percent of 55?" The solution involves setting up the equation 55 * (x / 100) = 48, then solving for x to get x = 48 * (100 / 55), which equals 87.27%. This example demonstrates how to calculate a percentage when the two numbers are relatively close in value, resulting in a percentage close to but less than 100%.
Solving equations with percents is a critical skill in mathematics that finds applications in various fields such as finance, statistics, and data analysis. These examples help students grasp the fundamental concept of relating two values through percentages and how to set up equations to solve for unknown percentages, particularly when dealing with values that are close to each other. This understanding forms the basis for more complex mathematical operations and real-world problem-solving scenarios, such as calculating percentage differences, analyzing proportions, or understanding relative values in different contexts.
Providing multiple worked-out examples is essential for students to fully comprehend this concept. Each new example reinforces the process while introducing different scenarios and number relationships. This approach allows students to recognize patterns, adapt their problem-solving strategies, and build confidence in handling diverse percentage-based calculations. By practicing with various value pairs, including those that result in percentages close to 100%, students develop a more nuanced understanding of how percentages relate different quantities and prepare for more advanced mathematical challenges they may encounter in higher education or professional settings.
Teacher Script: "Let's examine this percent problem. We're asked, '48 is what percent of 55?' To solve this, we set up the equation 55 * (x / 100) = 48. Now, how do we solve for x? We multiply both sides by 100/55. This gives us x = 48 * (100 / 55), which equals approximately 87.27%. Notice that our result is close to, but less than, 100%. This means that 48 is slightly less than 55. In real-world scenarios, you might encounter situations where you need to express one value as a percentage of another similar value. For example, if a company's current year sales were $48 million compared to last year's $55 million, you could say they're operating at about 87.27% of last year's level. Understanding these concepts is crucial for analyzing year-over-year performance, comparing values across similar scales, and interpreting proportions in various fields like business, economics, and data analysis."
For a complete collection of math examples related to Solving Equations click on this link: Math Examples: Equations with Percents Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.EE.B.5, CCSS.MATH.CONTENT.7.RP.A.3, CCSS.MATH.CONTENT.6.RP.A.3.C |
|---|---|
| Grade Range | 5 - 8 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Percent Equations |
| Copyright Year | 2013 |
| Keywords | Percent, equation, solution, solving equation, percentage |