Display Title
Math Example--Polynomial Concepts--Degree of a Polynomial: Example 4
Display Title
Math Example--Polynomial Concepts--Degree of a Polynomial: Example 4
Topic
Polynomials
Description
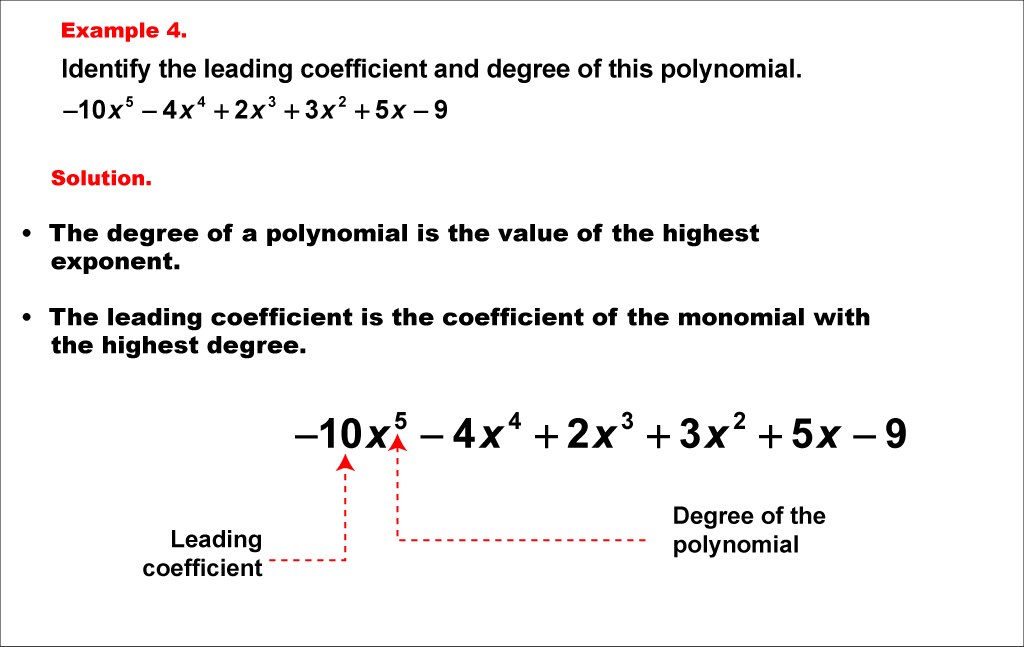
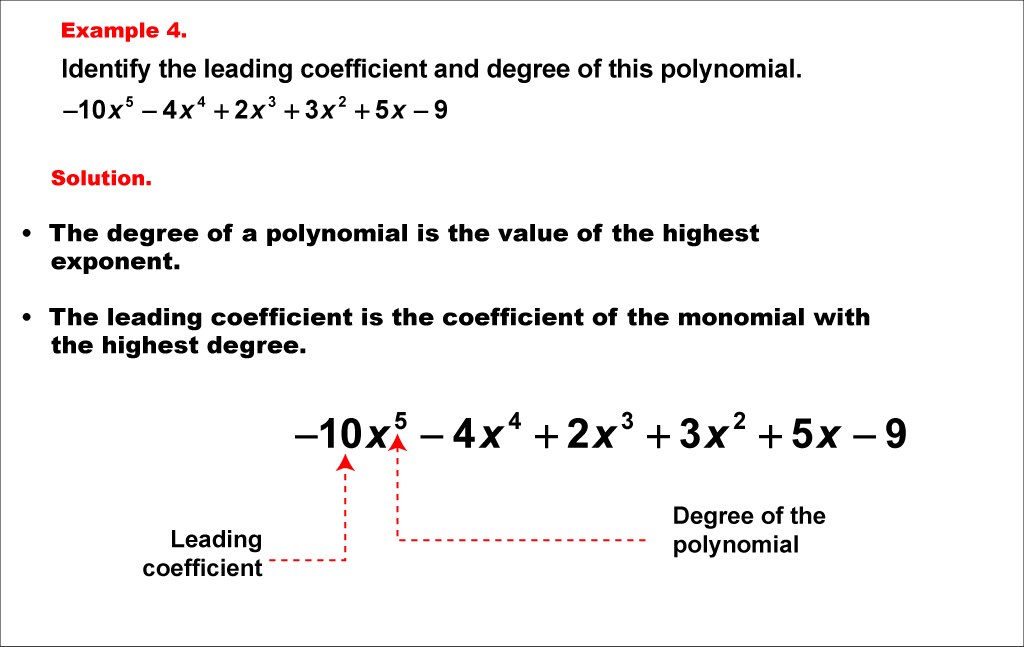
The example demonstrates how to identify and understand the degree of a polynomial. The degree is determined by the highest power of the variable. The degree of the polynomial is given by the highest exponent, which is 5. The leading coefficient is the coefficient of the term with the highest degree, which is -10 for -10x5. Thus, the degree of the polynomial is 5, and the leading coefficient is -10.
The degree of a polynomial is a fundamental concept in algebra that describes the highest exponent of the variable in the polynomial expression. Understanding this helps students recognize the structure and behavior of polynomials, which is crucial for solving equations and modeling real-world scenarios. The examples in this collection systematically guide students through identifying and analyzing polynomial degrees in various contexts.
Seeing multiple worked-out examples allows students to observe patterns and reinforces their understanding. This repetition is especially important for concepts like polynomials, where visualizing different forms and configurations aids comprehension.
Teacher's Script: Let's look at this example together. The polynomial shown here has terms with different powers of the variable. To determine the degree, identify the term with the highest exponent. In this case, the degree is highlighted. Remember, the degree tells us important information about the polynomial's behavior and structure.
For a complete collection of math examples related to Polynomials click on this link: Math Examples: Degree of a Polynomial Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.A.1 |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Polynomials • Polynomial Expressions |
| Copyright Year | 2021 |
| Keywords | Degree of a Polynomial, coefficient |