Display Title
Math Example--Polynomial Concepts--The Fundamental Theorem of Algebra--Example 02
Display Title
Math Example--Polynomial Concepts--The Fundamental Theorem of Algebra--Example 02
Topic
Polynomials
Description
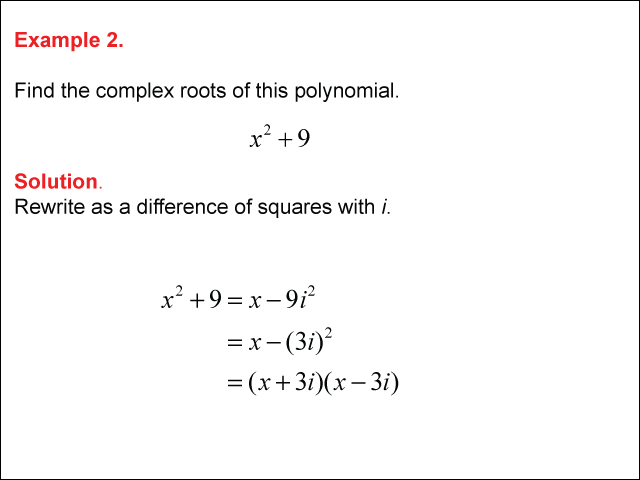
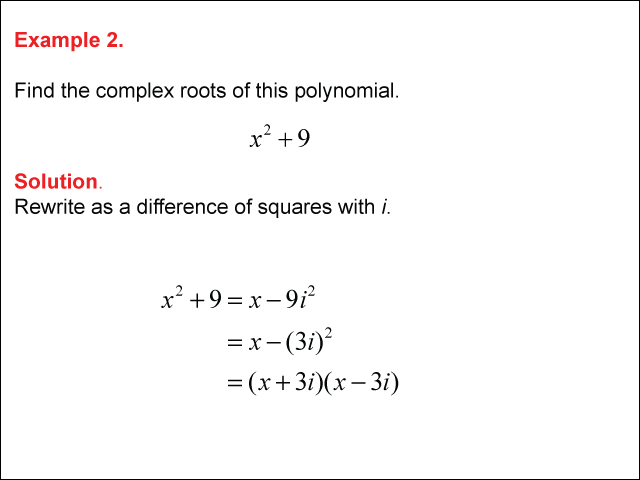
The image shows Example 2, where the task is to find the complex roots of x2 + 9. The solution is presented by rewriting the equation as a difference of squares with i. Example 2: The equation is x2 + 9 = (x + 3i)(x - 3i). This example factors the quadratic expression and finds its complex roots.
In this topic, students explore the Fundamental Theorem of Algebra by finding the complex roots of various polynomials. Each example provides a structured approach to breaking down a polynomial and examining its complex roots by using the difference of squares and imaginary units. This technique helps students visualize how complex roots are derived and connects the concept of imaginary numbers to polynomial roots.
Seeing multiple worked-out examples is essential for students to understand fully the methods involved. By examining similar problems with slight variations, students can build confidence in using complex numbers, identifying patterns, and applying these techniques independently. Each example reinforces understanding by guiding students through step-by-step problem-solving.
Teacher’s Script: Let's look at this example together. We’re finding the complex roots for the equation The equation is x2 + 9 = (x + 3i)(x - 3i). Notice how we rewrite the polynomial using imaginary numbers. This lets us use the difference of squares method to factor it and find our complex roots. Pay close attention to how we handle the imaginary units—it’s a crucial part of finding complex roots accurately.
For a complete collection of math examples related to Fundamental Theorem of Algebra click on this link: Math Examples: Fundamental Theorem of Algebra Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSN.CN.C.7, CCSS.MATH.CONTENT.HSN.CN.C.8, CCSS.MATH.CONTENT.HSN.CN.C.9 |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Numerical and Algebraic Expressions |
| Copyright Year | 2020 |
| Keywords | complex numbers, Fundamental Theorem of Algebra |