Display Title
Math Example--Polynomial Concepts-- Perfect Squares and Cubes--Example 7
Display Title
Math Example--Polynomial Concepts-- Perfect Squares and Cubes--Example 7
Topic
Polynomials
Description
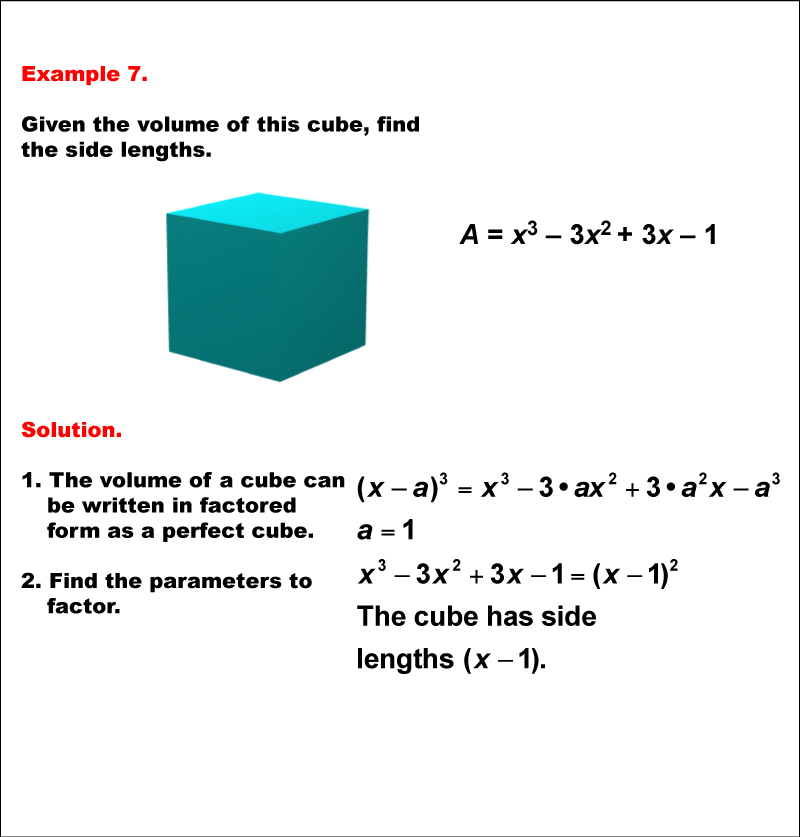
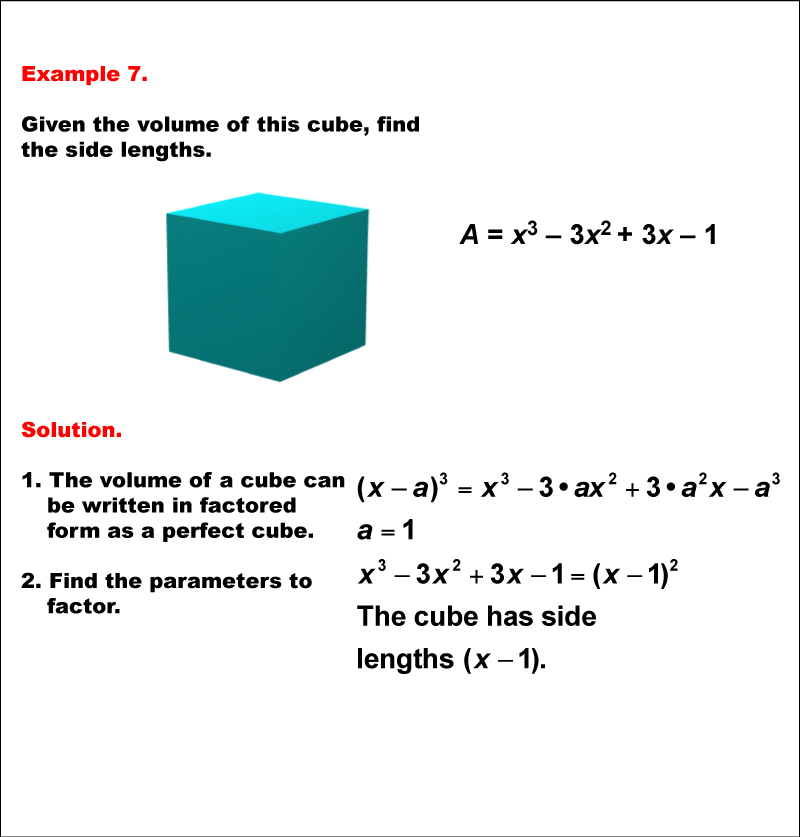
Another cubic example solving for side length with volume A = x3 - 3x2 + 3x - 1.
Example 7: For the volume A = x3 - 3x2 + 3x - 1, express as (x - 1)3 to determine side lengths of x - 1.
Polynomials involve expressions with variables raised to powers, and these examples specifically address perfect squares and cubes. Each example in this collection explores how to derive side lengths or volumes using factorization, demonstrating the practical applications of polynomial expressions.
Seeing multiple worked-out examples is essential for students to grasp the variations and nuances of solving polynomial equations. Repeated exposure to examples with subtle differences helps students build confidence and reinforce their understanding of core concepts.
Teacher's Script: Let's look at this example where we find the side length of a shape based on a given polynomial expression. For instance, when we have A = x3 - 3x2 + 3x - 1, express as (x - 1)3 to determine side lengths of x - 1, our goal is to factor this expression to determine the side length. This process helps us see the underlying structure of polynomials and understand how to break down complex expressions.
For a complete collection of math examples related to Polynomials click on this link: Math Examples: Perfect Squares and Cubes Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.EE.B.6, CCSS.MATH.CONTENT.HSA.SSE.A.2 |
|---|---|
| Grade Range | 6 - 10 |
| Curriculum Nodes |
Algebra • The Language of Math • Variable Expressions |
| Copyright Year | 2020 |
| Keywords | area, volume |