
Display Title
Math Example--Polynomial Concepts--Polynomial Long Division--Example 03
Display Title
Math Example--Polynomial Concepts--Polynomial Long Division--Example 03
Topic
Polynomials
Description
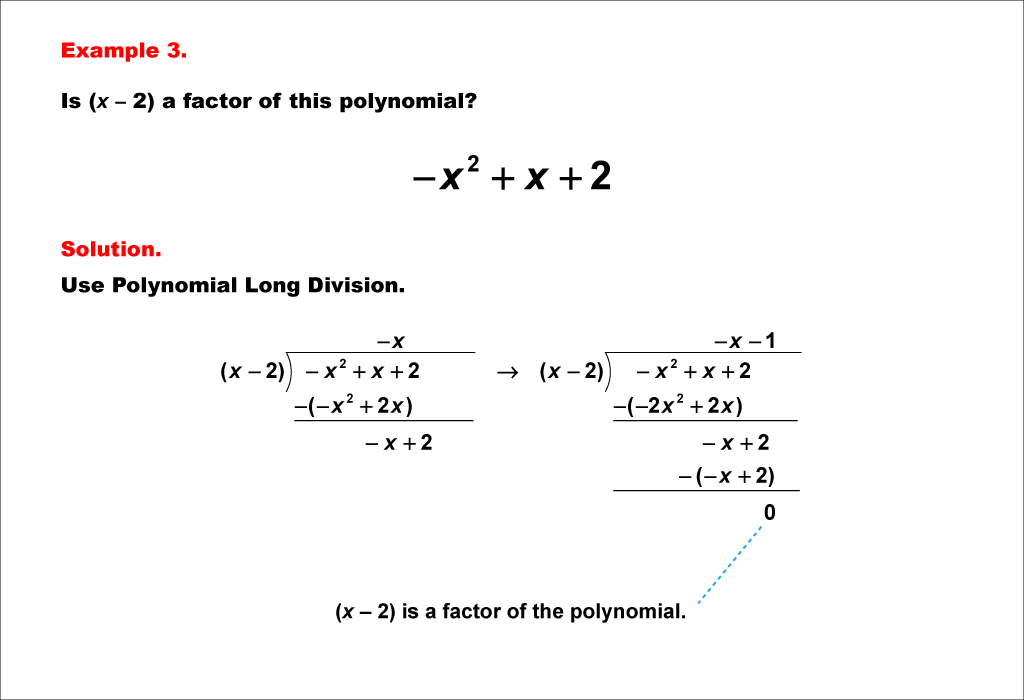
Determine if (x - 2) is a factor of the polynomial -x2 + x + 2. Perform polynomial long division by dividing -x2 + x + 2 by (x - 2). Divide the leading term -x2 by x to get -x, and proceed with the subtraction and simplification. After completing the division steps, the remainder is 0. Therefore, (x - 2) is a factor of -x2 + x + 2.
Polynomial division is a technique used to divide one polynomial by another, breaking down complex expressions into simpler forms. This collection focuses on examples that illustrate polynomial long division, a method crucial in algebraic manipulation and in understanding factorization and remainder determination.
Seeing multiple worked-out examples is essential for students, as it reinforces the steps involved in polynomial division and provides diverse scenarios that strengthen understanding and confidence.
Teacher’s Script: As we explore polynomial division, let's look at this example. In this case, we are dividing using the divisor provided to see if it’s a factor. Follow each step to observe how terms are divided and remainders calculated. Pay attention to how we align terms and subtract accurately.
For a complete collection of math examples related to Polynomials click on this link: Math Examples: Polynomial Long Division Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSA.APR.B.2 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Polynomials • Polynomial Expressions • Polynomial Functions and Equations |
| Copyright Year | 2021 |
| Keywords | polynomials |