Display Title
Math Example--Polynomial Concepts-- Rational Root Theorem: Example 2
Display Title
Math Example--Polynomial Concepts-- Rational Root Theorem: Example 2
Topic
Polynomials
Description
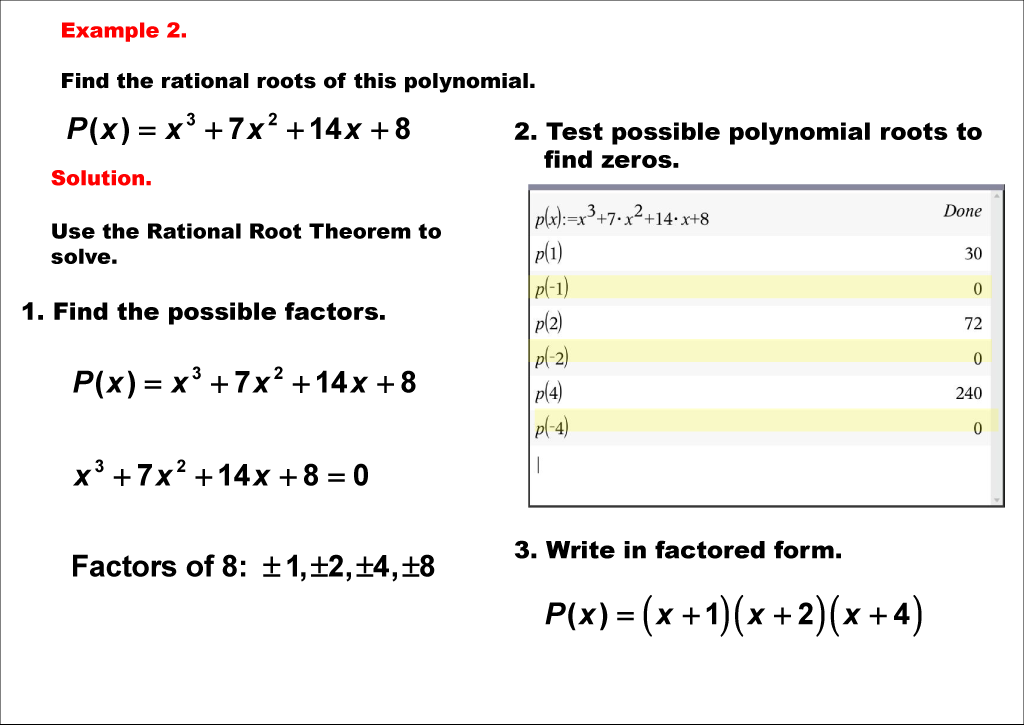
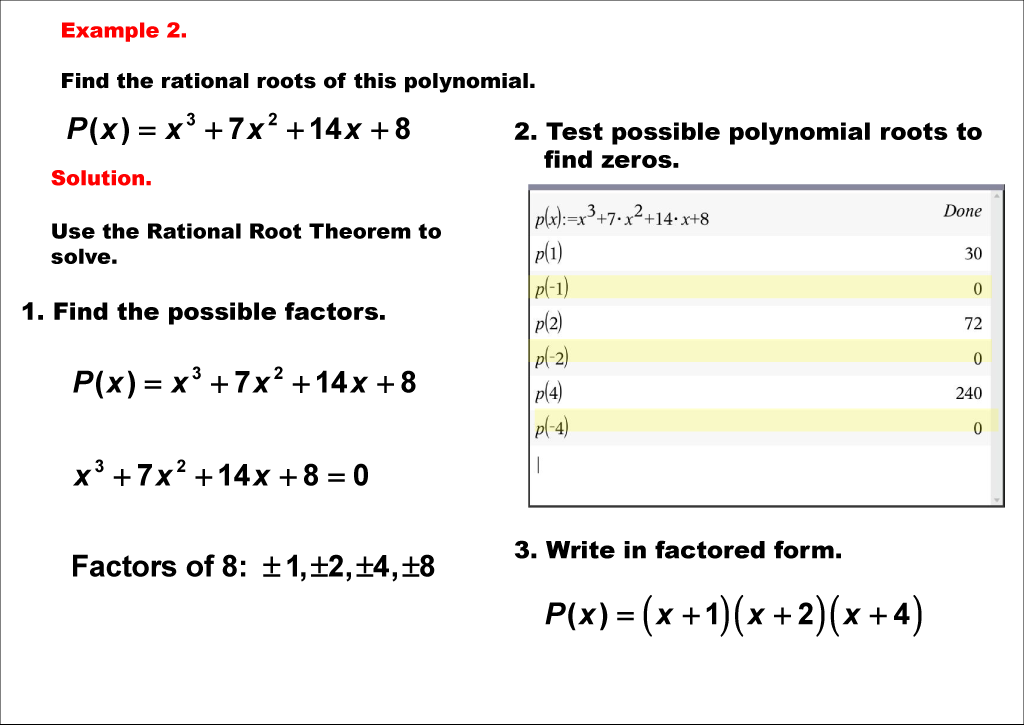
The example illustrates the process of finding rational roots for the polynomial. Find the rational roots of the polynomial P(x) = x3 + 7x2 + 14x + 8 using the Rational Root Theorem. 1. Identify possible factors of the constant term (8), which are ±1, ±2, ±4, and ±8. 2. Test each factor by substituting them into the polynomial to find the roots. Roots found are x = -1, -2, and -4. 3. The polynomial can be factored as P(x) = x3 + 7x2 + 14x + 8. The answer is P(x) = (x + 1)(x + 2)(x + 4).
The topic of rational roots in polynomials is central to understanding algebraic functions. By exploring different examples, students can grasp the methodical process required to test potential roots using substitution or synthetic division. The examples in this collection cover various cases, helping students see patterns and apply strategies effectively.
Seeing multiple worked-out examples is critical for students to develop a strong foundation in algebra. These examples reinforce the step-by-step approach to solving polynomial equations, enabling learners to build confidence and accuracy in their calculations.
Teacher’s Script: Today we’re working on finding rational roots for polynomials. Look at this example where we analyze the polynomial. Notice how we test possible roots based on the factors of the constant term and leading coefficient. Let’s explore each step together, ensuring you understand the logic behind testing each possible value.
For a complete collection of math examples related to Polynomials click on this link: Math Examples: Rational Root Theorem Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.B.3 |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Polynomials • Factoring Polynomials |
| Copyright Year | 2021 |
| Keywords | Rational Root Theorem |