Display Title
Math Example--Polynomial Concepts--Synthetic Division--Example 12
Display Title
Math Example--Polynomial Concepts--Synthetic Division--Example 12

Topic
Polynomials
Description
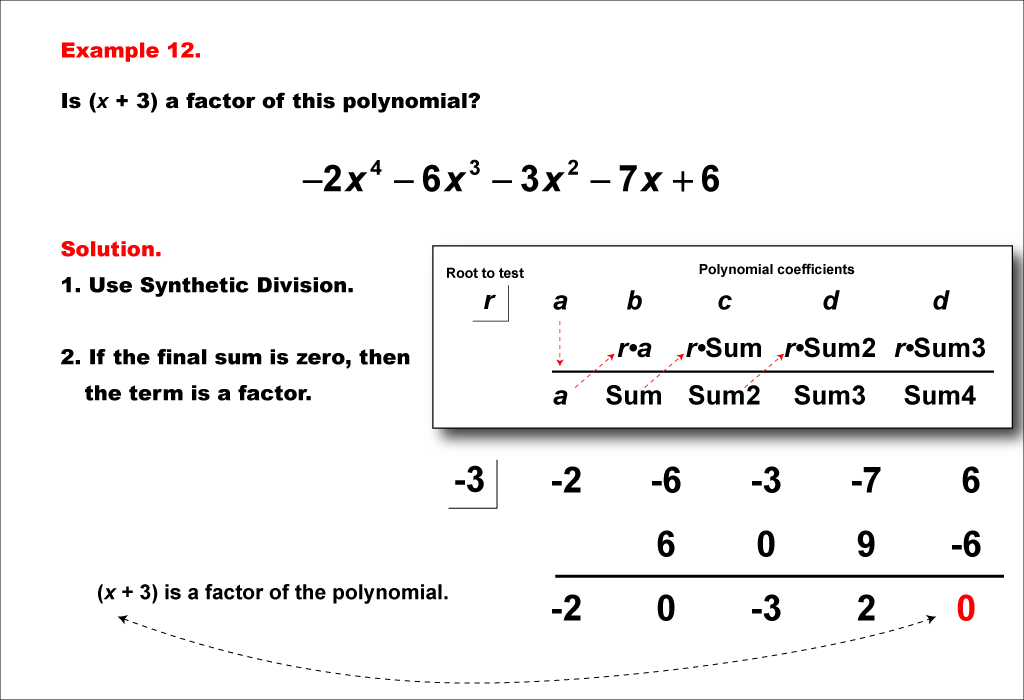
Determine if (x + 3) is a factor of the polynomial -2x4 - 6x3 - 3x2 - 7x + 6.. 1. Use synthetic division by setting the root to test as r = -3 (since x + 3 = 0 implies x = -3). 2. Apply synthetic division with coefficients -2, -6, -3, -7, 6. 3. Perform synthetic division steps, yielding a final sum of 0. 4. Since the remainder is 0, (x + 3) is confirmed as a factor of the polynomial..
The topic of synthetic division is explored here, which is a simplified way to divide polynomials, often used when the divisor is in the form (x - a). These examples showcase how synthetic division allows for faster computations compared to traditional polynomial division. By using synthetic division, students see a step-by-step approach to finding factors of polynomials, which reinforces their understanding of polynomial functions and factorization.
Seeing multiple worked-out examples is crucial for students to fully grasp synthetic division. It allows them to observe the pattern in computations and how each step builds toward simplifying a polynomial division problem. This reinforces their procedural skills and helps them recognize common errors.
Teacher’s Script: Let's examine how synthetic division works with this example. Look at the divisor and set it up to divide our polynomial. Follow each step to see how coefficients change as we progress through the division. This helps you identify the remainder and whether the divisor is a factor of the polynomial.
For a complete collection of math examples related to Polynomials click on this link: Math Examples: Synthetic Division Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSA.APR.B.2 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Polynomials • Polynomial Expressions • Polynomial Functions and Equations |
| Copyright Year | 2021 |
| Keywords | polynomials |