Display Title
Math Example--Probability Concepts--The Probability of Independent Events--Example 10
Display Title
Math Example--Probability Concepts--The Probability of Independent Events--Example 10
Topic
Probability and Statistics
Description
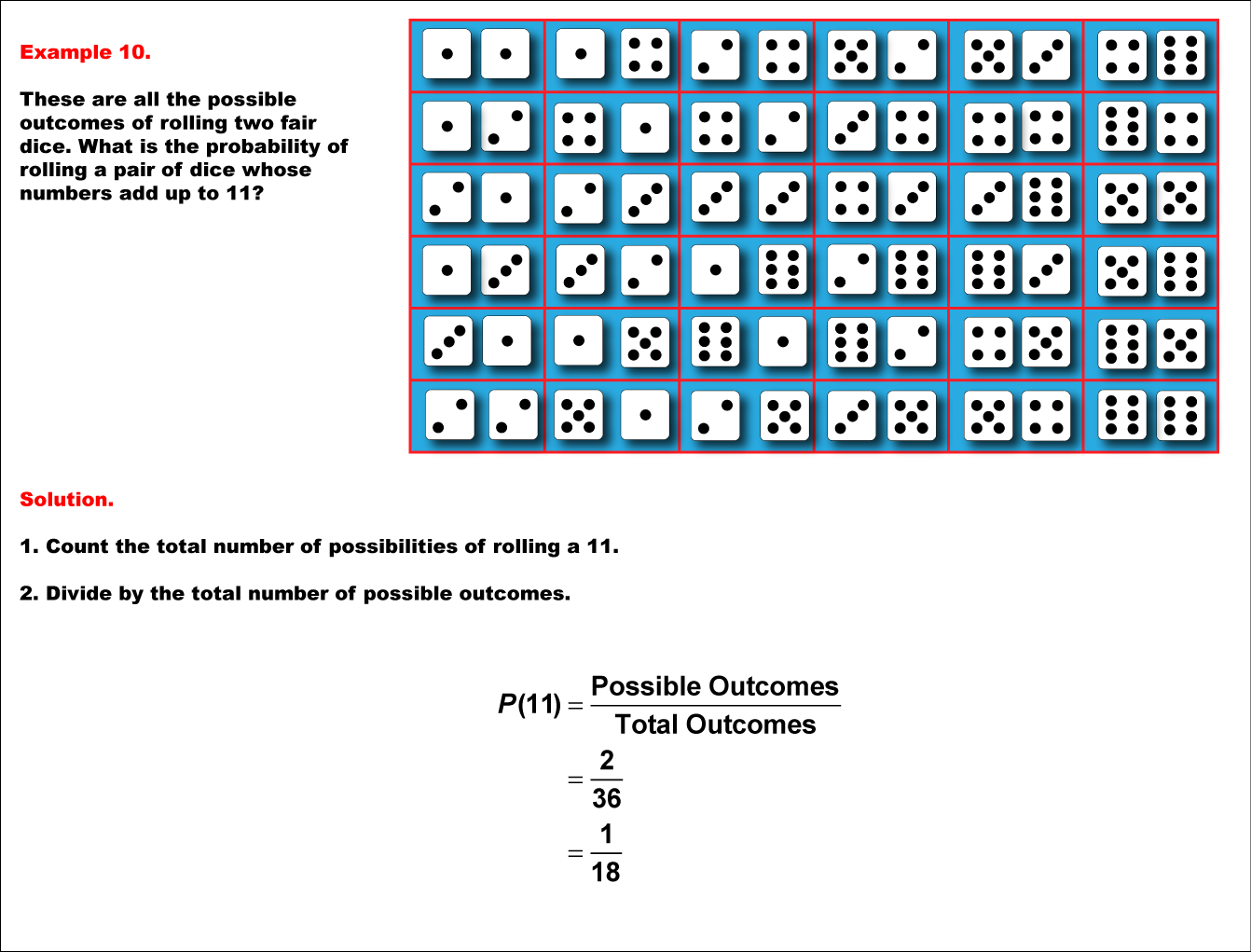
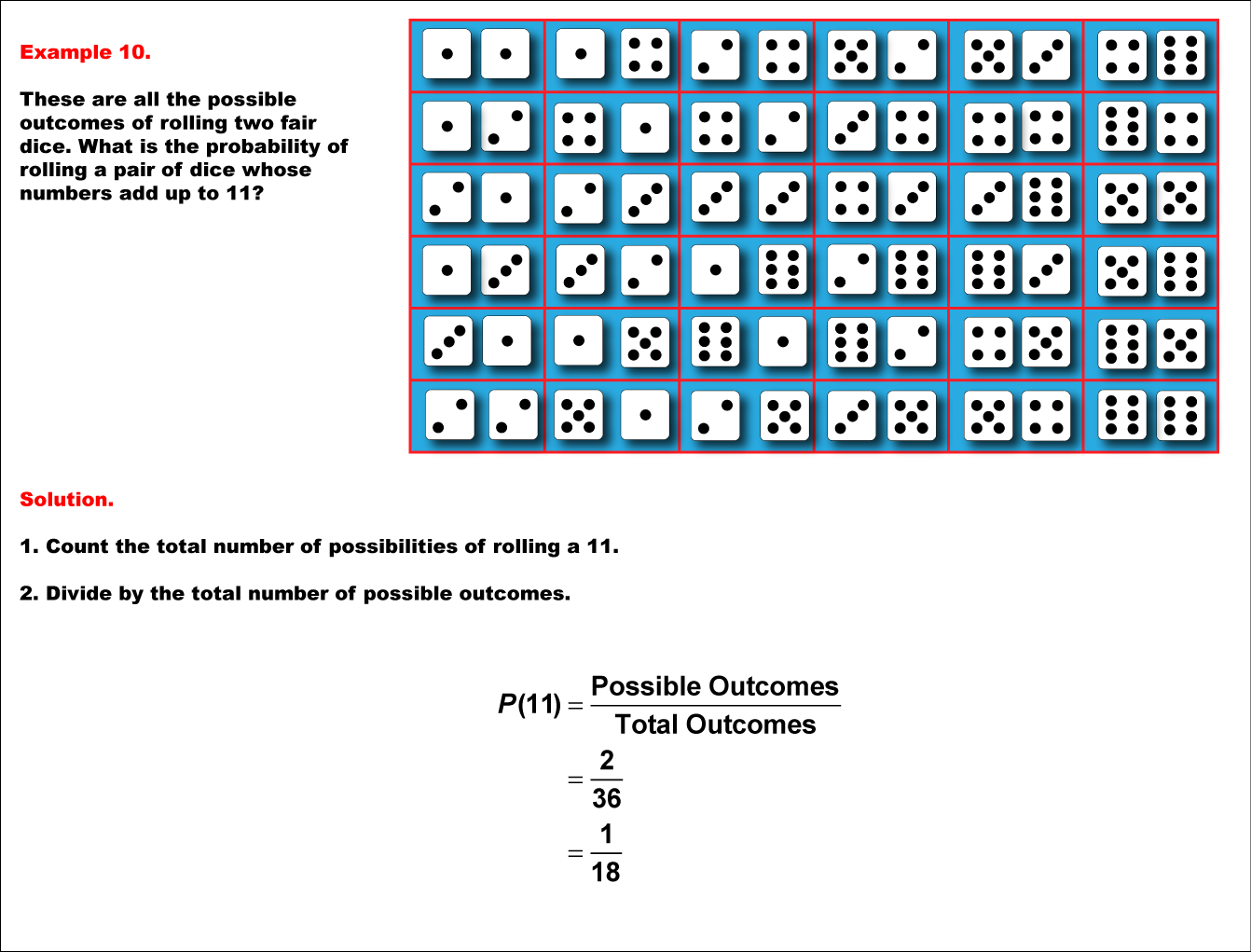
This example illustrates a situation where understanding probability through visual aids can greatly enhance learning. Example 10. These are all the possible outcomes of rolling two fair dice. What is the probability of rolling a pair of dice whose numbers add up to 11? Solution. Count the total number of possibilities of rolling an 11. Divide by the total number of possible outcomes By seeing multiple worked-out examples, students can deepen their comprehension and master essential concepts in probability. Visual representations help in connecting abstract ideas to practical applications. Having access to various examples enables students to approach problems from different angles, which is indispensable for effective understanding. Furthermore, it cultivates critical thinking and problem-solving skills, as students can compare and contrast different scenarios. Ultimately, consistent practice with a variety of examples is vital for internalizing mathematical principles.
For a complete collection of math examples related to Probability click on this link: Math Examples: Probability of Independent Events Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSS.CP.A.1, CCSS.MATH.CONTENT.HSS.CP.A.2, CCSS.MATH.CONTENT.HSS.CP.A.3, CCSS.MATH.CONTENT.HSS.CP.A.4, CCSS.MATH.CONTENT.HSS.CP.A.5, CCSS.MATH.CONTENT.HSS.CP.B.6, CCSS.MATH.CONTENT.HSS.CP.B.7, CCSS.MATH.CONTENT.HSS.CP.B.8, CCSS.MATH.CONTENT.HSS.MD.A.1, CCSS.MATH.CONTENT.HSS.MD.A.2, CCSS.MATH.CONTENT.HSS.MD.A.3, CCSS.MATH.CONTENT.HSS.MD.A.4, CCSS.MATH.CONTENT.7.SP.C.8.A, CCSS.MATH.CONTENT.7.SP.C.8.B, CCSS.MATH.CONTENT.7.SP.C.8.C |
|---|---|
| Grade Range | 7 - 12 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Probability |
| Copyright Year | 2020 |
| Keywords | probability, Conditional Probability |