Display Title
Math Example--Quadratics--Conic Sections: Example 10
Display Title
Conic Sections: Example 10
Topic
Quadratics
Description
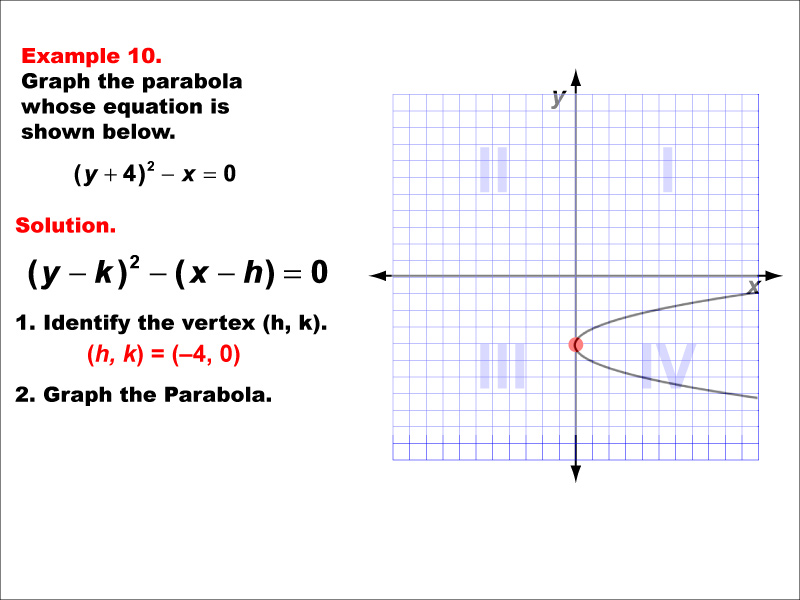
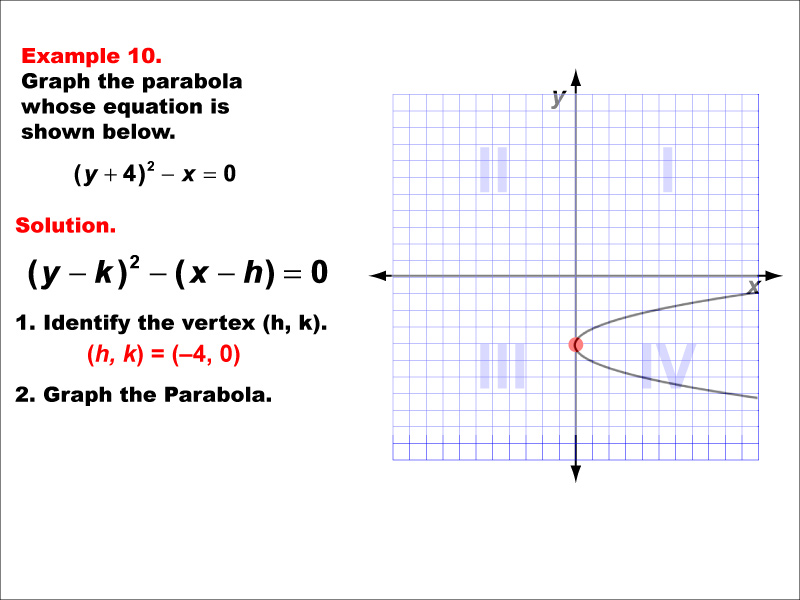
This example shows a horizontal parabola opening to the right. The characteristic equation for such a parabola is x = a(y - k)² + h, where (h, k) is the vertex and 'a' is positive. The vertex is on the y-axis at (-4, 0). Horizontal parabolas are not functions as they fail the vertical line test. This example demonstrates how parabolas can have different orientations, emphasizing the versatility of conic sections. Understanding these variations is crucial in fields like physics (projectile motion) and engineering (reflector design). Parabolas, as conic sections, are fundamental in mathematics and have numerous real-world applications, from satellite dishes to architectural design.
For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSG.GPE.A.1, CCSS.MATH.CONTENT.HSG.GPE.A.2, CCSS.MATH.CONTENT.HSG.GPE.A.3 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Functions and Relations • Conic Sections |
| Copyright Year | 2013 |
| Keywords | conic section, quadratic relations |