Display Title
Math Example--Quadratics--Conic Sections: Example 44
Display Title
Conic Sections: Example 44
Topic
Quadratics
Description
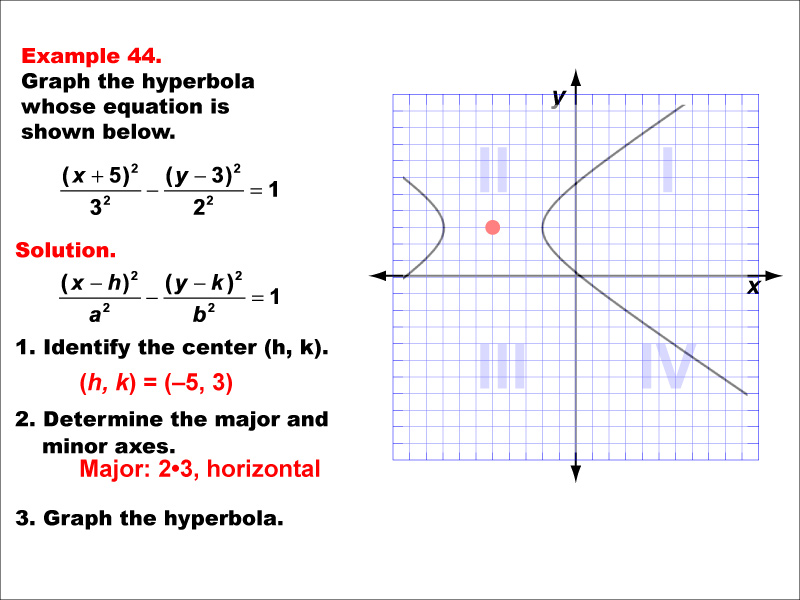
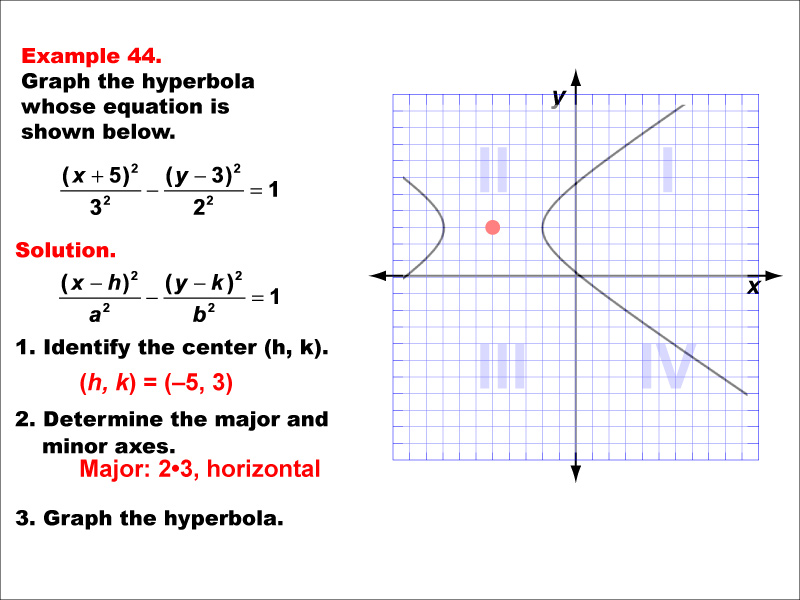
This example illustrates a hyperbola, a type of conic section characterized by two separate curves called branches. The general equation of a hyperbola is ((x - h)²/a²) - ((y - k)²/b²) = 1 for horizontal hyperbolas or ((y - k)²/a²) - ((x - h)²/b²) = 1 for vertical hyperbolas. This example demonstrates how the hyperbola is positioned on the coordinate plane and how its equation reflects this position. Understanding hyperbolas is crucial for applications in physics and engineering, such as analyzing the paths of celestial bodies or designing certain types of antennas. Skills involved include identifying the hyperbola's center, axes, and asymptotes, and graphing it accurately.
For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSG.GPE.A.1, CCSS.MATH.CONTENT.HSG.GPE.A.2, CCSS.MATH.CONTENT.HSG.GPE.A.3 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Functions and Relations • Conic Sections |
| Copyright Year | 2013 |
| Keywords | conic section, quadratic relations |