Display Title
Math Example--Quadratics--Factoring Quadratics: Example 8
Display Title
Factoring Quadratics: Example 8
Topic
Quadratics
Description
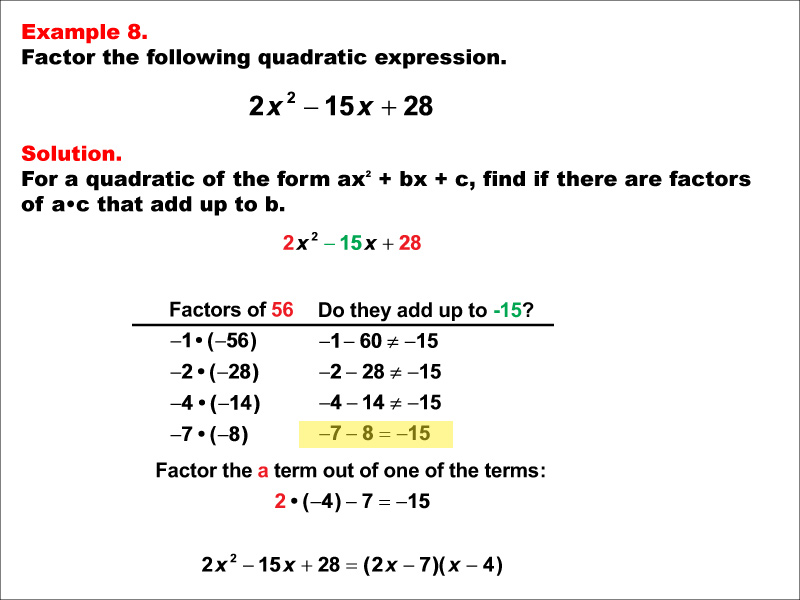
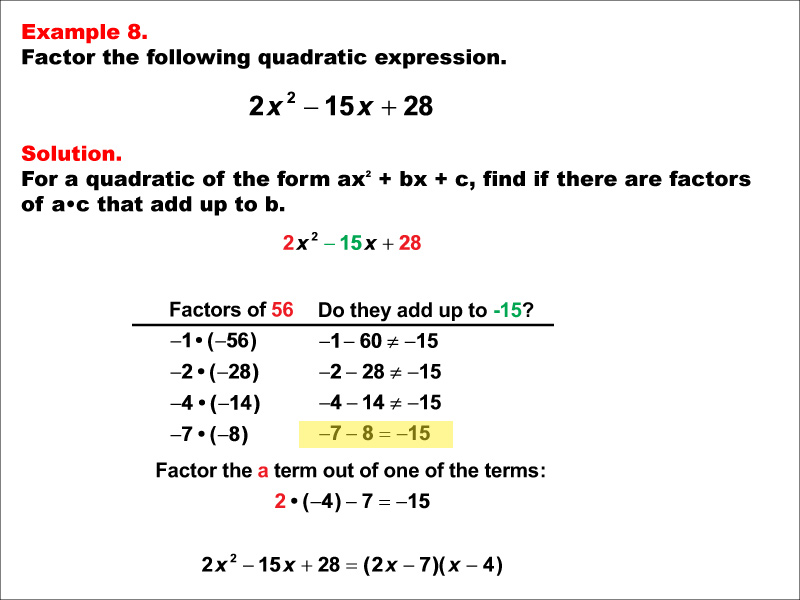
This example shows the factoring of a quadratic expression in standard form (ax² + bx + c), where a ≠ 1. The factoring process involves finding two numbers that multiply to give a•c and add up to b. For instance, if the expression is x² - 7x + 12, we would look for factors of 12 that add up to -7, resulting in (x - 3)(x - 4). This method is crucial for solving quadratic equations as it allows us to find the roots (or zeros) of the quadratic function. By setting each factor to zero, we can quickly determine the x-intercepts of the parabola. This type of factoring is often the first step in solving quadratic equations and is essential for understanding the structure of quadratic expressions.
For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSA.SSE.B.3.A, CCSS.MATH.CONTENT.HSF.IF.C.8.A |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Quadratic Functions and Equations • Factoring Quadratics |
| Copyright Year | 2013 |
| Keywords | quadratics, factoring |