Display Title
Math Example--Quadratics--Parabola Maximum and Minimum: Example 5
Display Title
Parabola Maximum and Minimum: Example 5
Topic
Quadratics
Description
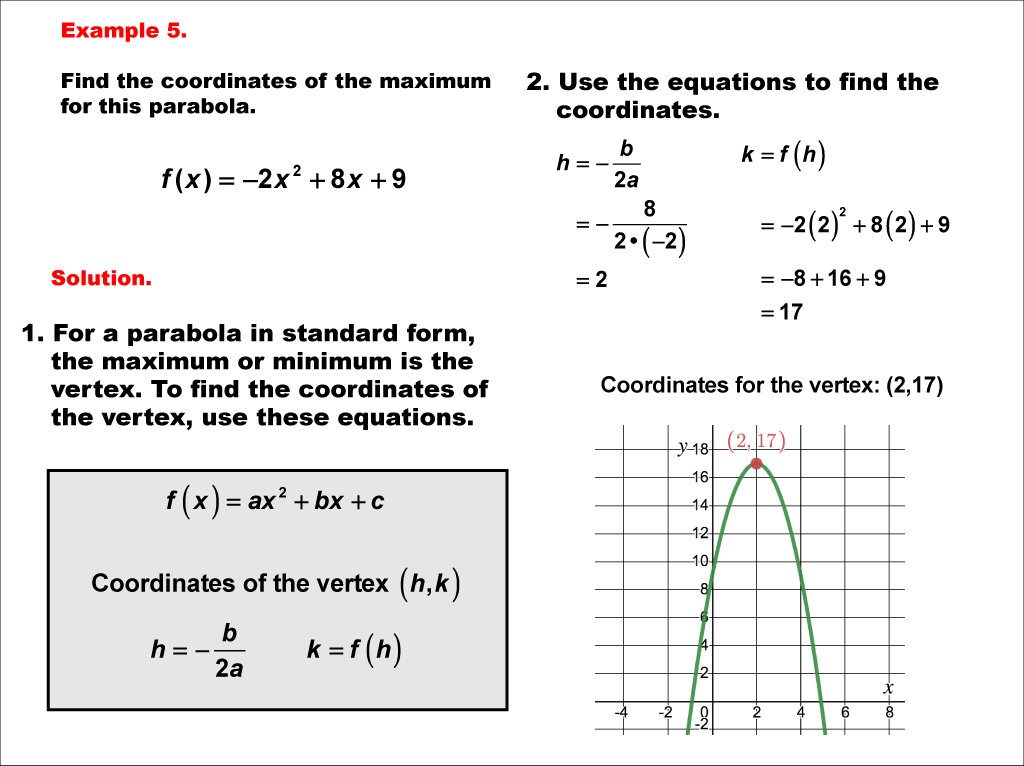
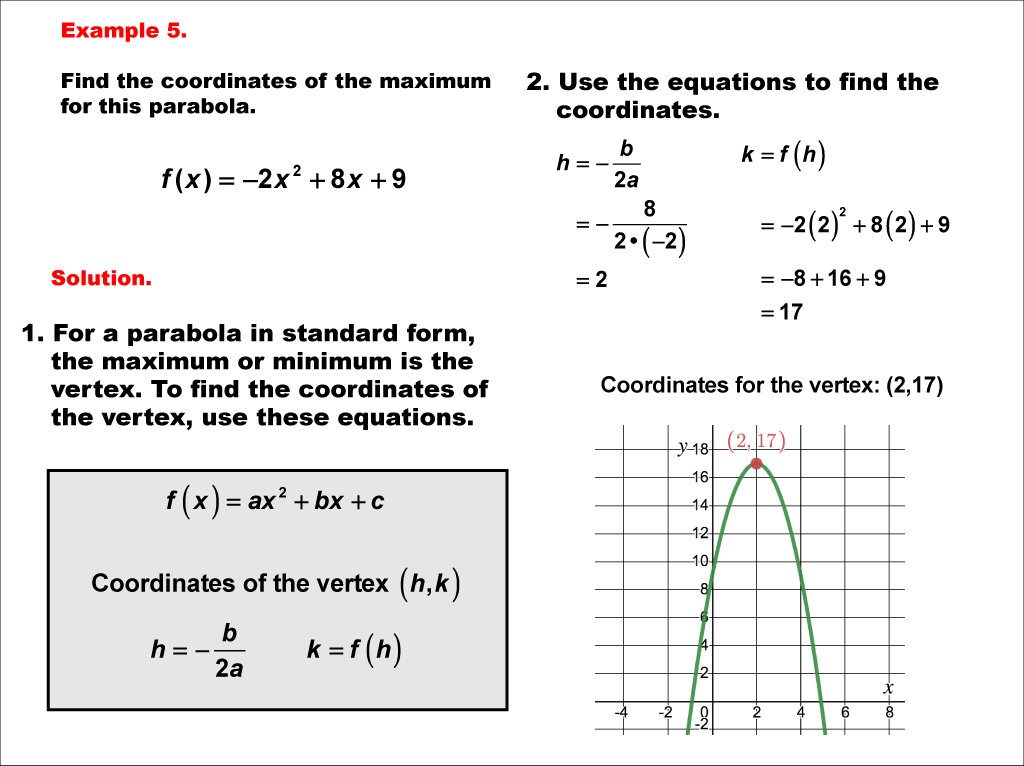
The maximum or minimum value of a parabola occurs at its vertex. For a parabola opening upward (a > 0), this point is a minimum. For a parabola opening downward (a < 0), it's a maximum. The vertex lies on the axis of symmetry.
For a quadratic function in standard form f(x) = ax² + bx + c, the x-coordinate of the vertex is given by x = -b/(2a), and the y-coordinate (maximum or minimum value) can be calculated by substituting this x-value into the original function.
This example shows f(x) = -2x² + 8x + 9. With a = -2 being negative, the parabola opens downward and has a maximum value. The x-coordinate of the vertex is x = -b/(2a) = 2. The maximum y-value is f(2) = 17. Therefore, the maximum value is 17, occurring at x = 2. The vertex (2, 17) is on the axis of symmetry x = 2.
For a complete collection of math examples related to Quadratics click on this link: Math Examples: Quadratics Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.8.A |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Quadratic Functions and Equations • Graphs of Quadratic Functions • Quadratic Equations and Functions |
| Copyright Year | 2021 |
| Keywords | parabola, maximum, minimum |