Display Title
Math Example--Rational Concepts--Graphs of Rational Functions: Example 7
Display Title
Math Example--Rational Concepts--Graphs of Rational Functions: Example 7
Topic
Rational Functions
Description
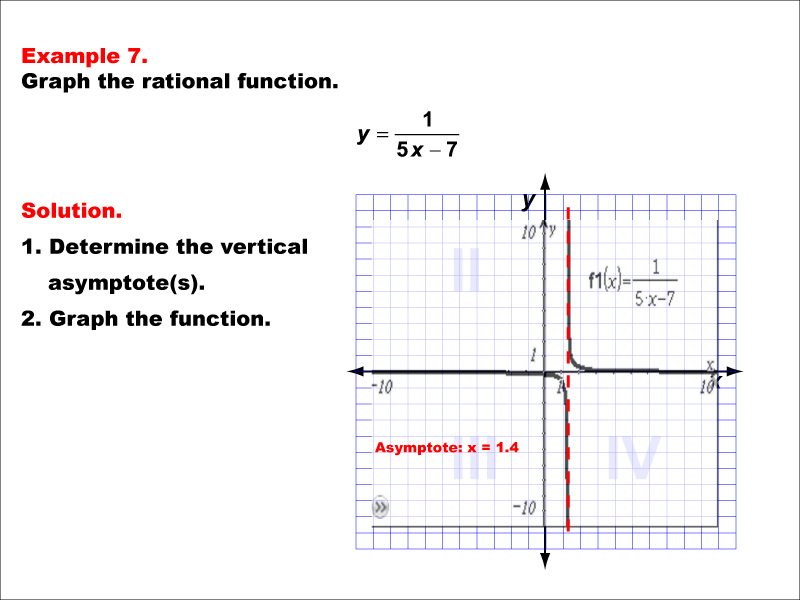
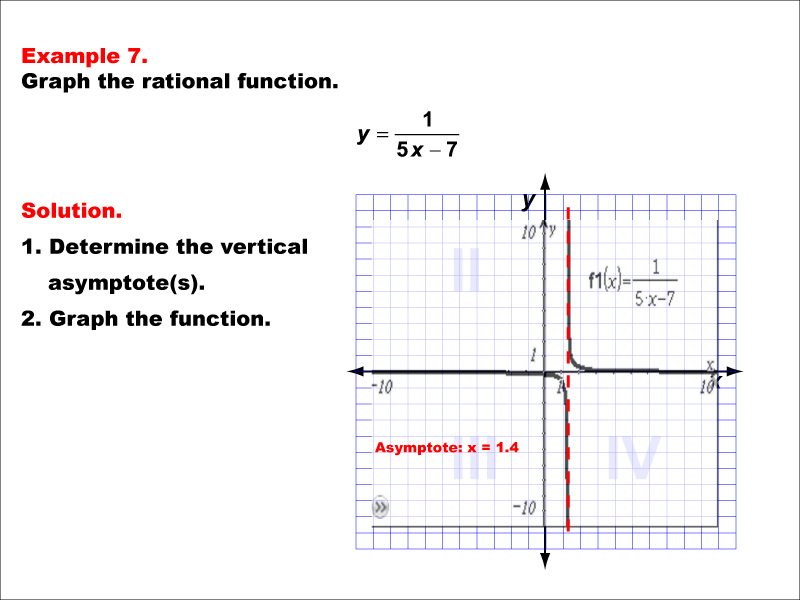
This example showcases the graph of the rational function y = 1 / (5x - 7). The graph features a vertical asymptote at x = 1.4, marked by a dashed red line on the grid. Students are tasked with graphing the function and determining its vertical asymptote.
Rational functions are a significant topic in algebra, involving fractions of polynomial functions. This collection of examples facilitates teaching the topic by providing visual representations of various rational functions, enabling students to observe how changes in the function affect its graph. By studying multiple examples, students can identify patterns and develop a deeper understanding of asymptotes and function behavior.
Exposure to multiple worked-out examples is crucial for students to fully grasp mathematical concepts. It allows them to encounter different scenarios, reinforces key ideas, and helps develop problem-solving skills. This repetitive exposure can boost confidence and enhance overall comprehension of rational functions.
Teacher's Script
In this example, we see that the vertical asymptote is at x = 1.4. This can be found by solving 5x - 7 = 0. Notice how the function approaches positive infinity as x approaches 1.4 from the right, and negative infinity as x approaches 1.4 from the left. Understanding how to determine the asymptote from the equation and visualize its effect on the graph is essential for mastering rational functions.
For a complete collection of math examples related to Rational Functions click on this link: Math Examples: Graphs of Rational Functions Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.F.B.5, CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.IF.C.7.D |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Rational Expressions and Functions • Rational Functions and Equations |
| Copyright Year | 2013 |
| Keywords | functions, rational functions |