Display Title
Math Example--Rational Concepts--Rational Functions in Tabular and Graph Form: Example 26
Display Title
Math Example--Rational Concepts--Rational Functions in Tabular and Graph Form: Example 26
Topic
Rational Functions
Description
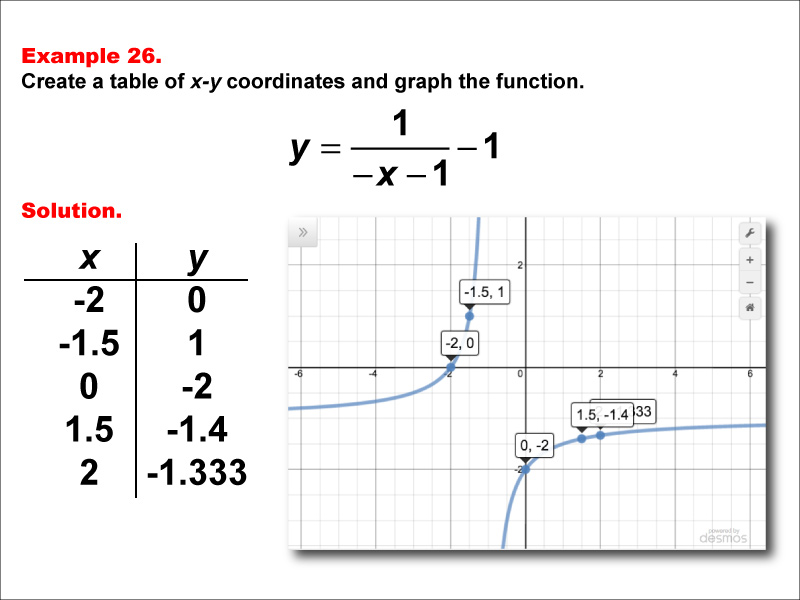
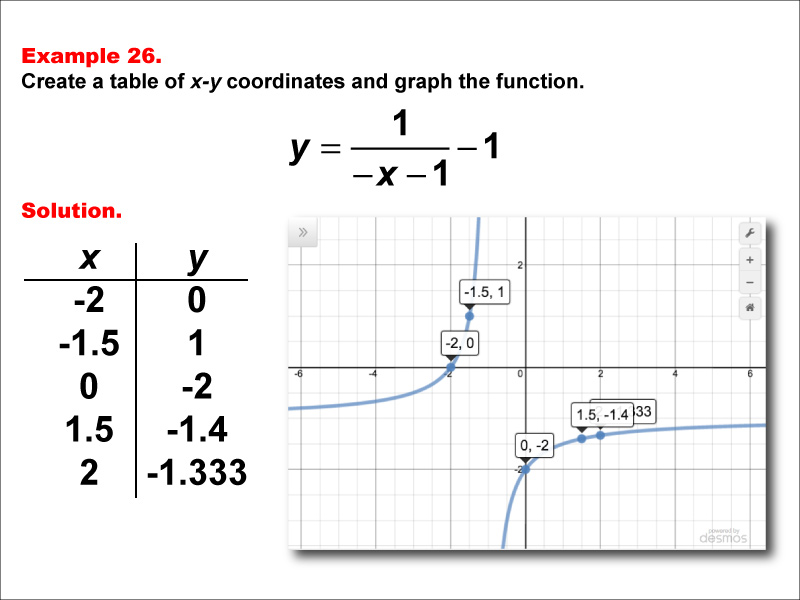
This math example focuses on creating a table of x-y coordinates and graphing the function y = 1 / (-x - 1) - 1. The image provided shows both a table of x-y coordinates and the corresponding graph of the function. The graph shows points plotted at (-2, 0), (-1.5, 1), (0, -2), (1.5, -1.4), and (2, -1.333), illustrating how negating both terms inside the parentheses and subtracting a constant from the entire fraction affects the graph's shape and position.
Rational functions are a crucial topic in algebra and calculus, representing the ratio of two polynomial functions with additional transformations. This collection of examples helps teach this topic by providing visual representations of various rational functions, allowing students to see how changes in the function affect its graph. By examining multiple examples, students can identify patterns and develop a deeper understanding of how negations and constants influence rational functions' behavior.
Seeing multiple worked-out examples is essential for students to fully grasp the concept of rational functions and their transformations. Each example builds upon the previous ones, introducing combinations of changes in the function that result in different graph shapes and positions. This approach helps students develop pattern recognition skills and enhances their ability to predict the behavior of rational functions based on their equations.
Teacher's Script: Now, let's analyze our twenty-sixth example: y = 1 / (-x - 1) - 1. How does this function compare to our previous example? Notice that we've kept the negations inside the parentheses but now we're subtracting 1 from the entire fraction instead of adding it. What effect do you think this change will have on the graph? As you observe the graph, can you explain why it's shifted vertically compared to the previous example? How does it compare to y = 1 / (x + 1) - 1? As we continue exploring more examples, try to predict how different combinations of negations and constants will affect the graphs.
For a complete collection of math examples related to Rational Functions click on this link: Math Examples: Rational Functions in Tables and Graphs Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.IF.C.7.D |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Rational Expressions and Functions • Rational Functions and Equations |
| Copyright Year | 2015 |
| Keywords | function, rational functions, graphs of rational functions, rational function tables |