
Display Title
Math Example--Ratios and Rates--Example 13
Display Title
Math Example--Ratios and Rates--Example 13
Topic
Ratios and Rates
Description
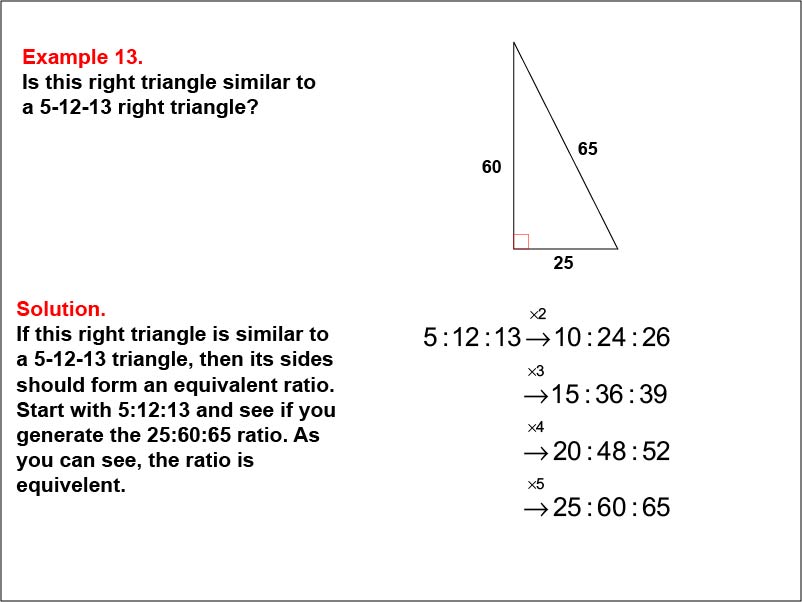
This example focuses on verifying the similarity of triangles using ratios. The image shows a right triangle with sides labeled 25, 60, and 65 units. Students are guided through a step-by-step process to determine if this triangle is similar to a 5-12-13 right triangle.
Understanding similar triangles and their ratios is a fundamental concept in geometry. This example illustrates how ratios can be used to compare the sides of different triangles and verify their similarity. By scaling the sides of a known triangle (5-12-13) and comparing them to the given triangle, students can see how ratios maintain proportionality in similar shapes.
Exposure to multiple worked-out examples is essential for students to fully comprehend the concept of ratios and their applications in geometry. Each example in this series presents a unique scenario, allowing students to practice identifying ratios, scaling them, and recognizing similarity. This repetition helps reinforce the concept and builds confidence in applying ratio skills to various geometric problems.
Teacher's Script: Let's examine this right triangle. Can you identify the lengths of its sides? Now, we want to check if this triangle is similar to a 5-12-13 triangle. How can we use ratios to determine this? Remember, similar triangles have proportional sides. What happens if we multiply each side of a 5-12-13 triangle by 5? How does this compare to our given triangle? Can you explain why this confirms similarity?
For a complete collection of math examples related to Ratios and Rates click on this link: Math Examples: Ratios and Rates Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.RP.A.1, CCSS.MATH.CONTENT.6.RP.A.2, CCSS.MATH.CONTENT.6.RP.A.3, CCSS.MATH.CONTENT.7.RP.A.1, CCSS.MATH.CONTENT.7.RP.A.2 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Algebra • Ratios, Proportions, and Percents • Ratios and Rates |
| Copyright Year | 2013 |
| Keywords | ratios, rates, unit rates, proportions, solving proportions, dimensional analysis |