Display Title
Math Example--Ratios and Rates--Example 26
Display Title
Math Example--Ratios and Rates--Example 26
Topic
Ratios and Rates
Description
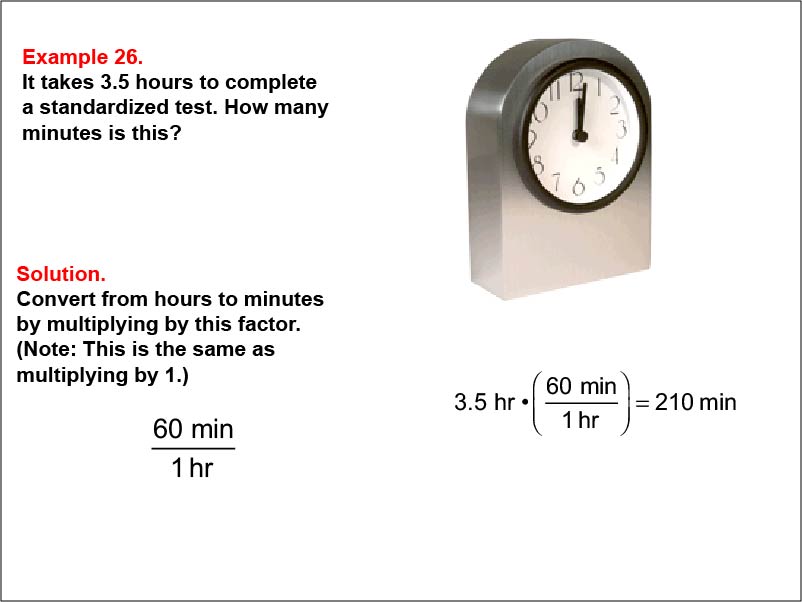
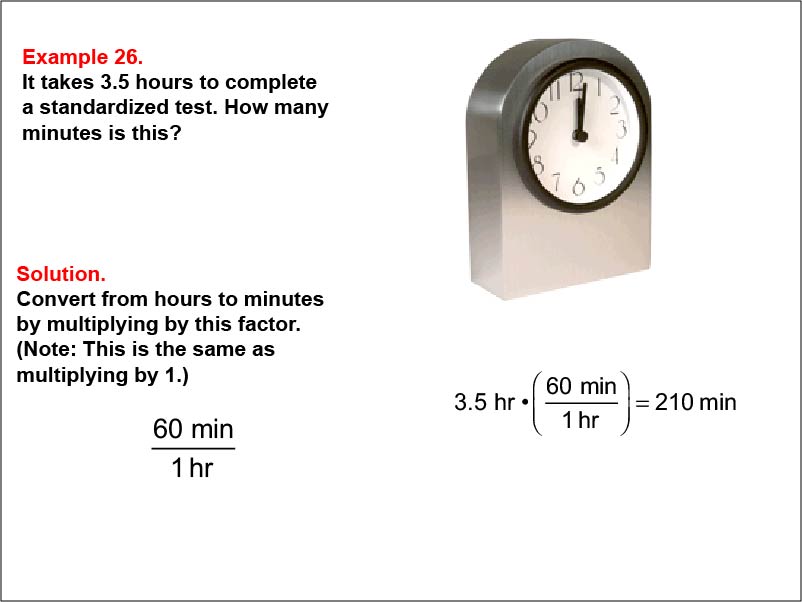
This example focuses on time unit conversion, specifically converting hours to minutes in the context of a standardized test duration. The image shows a clock, providing a visual representation of time for the mathematical problem.
Understanding time unit conversions is crucial in many aspects of daily life, including scheduling, time management, and test-taking. This example demonstrates how to use a conversion factor to change units, illustrating the practical application of multiplication in time-related scenarios.
Providing multiple worked-out examples is essential for students to fully grasp the concept of time unit conversions. Each example in this series presents a unique scenario, allowing students to practice converting between different time units. This repetition helps reinforce the concept and builds confidence in applying these skills to various real-world situations.
Teacher's Script: Let's examine this problem about the duration of a standardized test. We know the time in hours, but we want to express it in minutes. How can we convert from hours to minutes? What conversion factor do we need to use? Remember, we're essentially multiplying by 1, but in a form that allows us to change the unit. Can you walk me through the conversion process step by step? Why do we multiply by 60 in this case?
For a complete collection of math examples related to Ratios and Rates click on this link: Math Examples: Ratios and Rates Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.RP.A.1, CCSS.MATH.CONTENT.6.RP.A.2, CCSS.MATH.CONTENT.6.RP.A.3, CCSS.MATH.CONTENT.7.RP.A.1, CCSS.MATH.CONTENT.7.RP.A.2 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Algebra • Ratios, Proportions, and Percents • Ratios and Rates |
| Copyright Year | 2013 |
| Keywords | ratios, rates, unit rates, proportions, solving proportions, dimensional analysis |