Display Title
Math Example--Right Triangles-- Example 25
Display Title
Math Example--Right Triangles-- Example 25
Topic
Right Triangles
Description
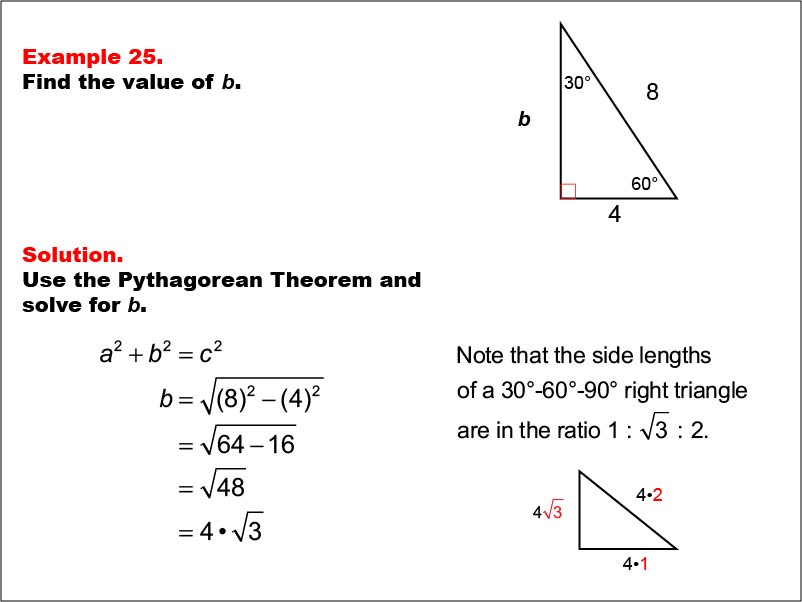
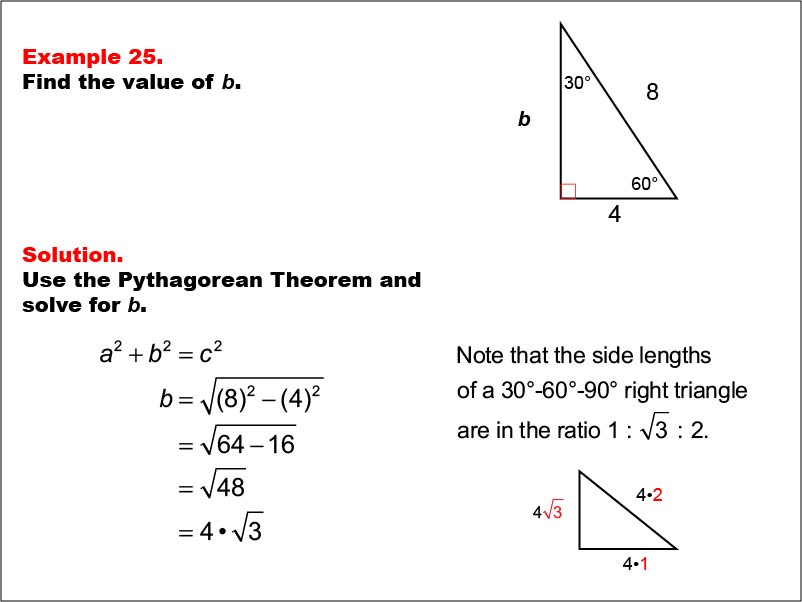
This example presents a 30-60-90 right triangle with a hypotenuse of 8, a shorter leg of 4, and an unknown longer leg b. Using the Pythagorean Theorem, we calculate that b = √(82 - 42) = √(64 - 16) = √(48) = 4 * √(3). This demonstrates that the side lengths of a 30-60-90 right triangle are in the ratio 1 : √(3) : 2, scaled by a factor of 4 in this case.
This example builds upon the previous one, showing how the properties of 30-60-90 triangles remain consistent when the dimensions are scaled. It helps students understand the relationship between different sized 30-60-90 triangles and how patterns emerge in their side lengths.
Providing multiple worked examples is crucial for students to fully grasp the concept of right triangles and their various applications. Each new example offers an opportunity to reinforce concepts, recognize patterns, and build problem-solving skills that can be applied to more complex geometric scenarios.
Teacher's Script: Let's examine our twenty-fifth example. We have another 30-60-90 triangle, but this time with larger dimensions. What do you notice about the hypotenuse compared to the shorter leg? That's right, it's twice the length. Let's solve for b and see how this relates to our previous example. How do you think scaling affects the properties of 30-60-90 triangles? Can you predict what the ratio of the sides will be before we calculate it?
For a complete collection of math examples related to Right Triangles click on this link: Math Examples: Right Triangles Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.B.6, CCSS.MATH.CONTENT.8.G.B.7, CCSS.MATH.CONTENT.6.G.A.1 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Geometry • Triangles • Right Triangles |
| Copyright Year | 2013 |
| Keywords | right triangles, leg, hypotenuse |