Display Title
Math Example--Right Triangles-- Example 26
Display Title
Math Example--Right Triangles-- Example 26
Topic
Right Triangles
Description
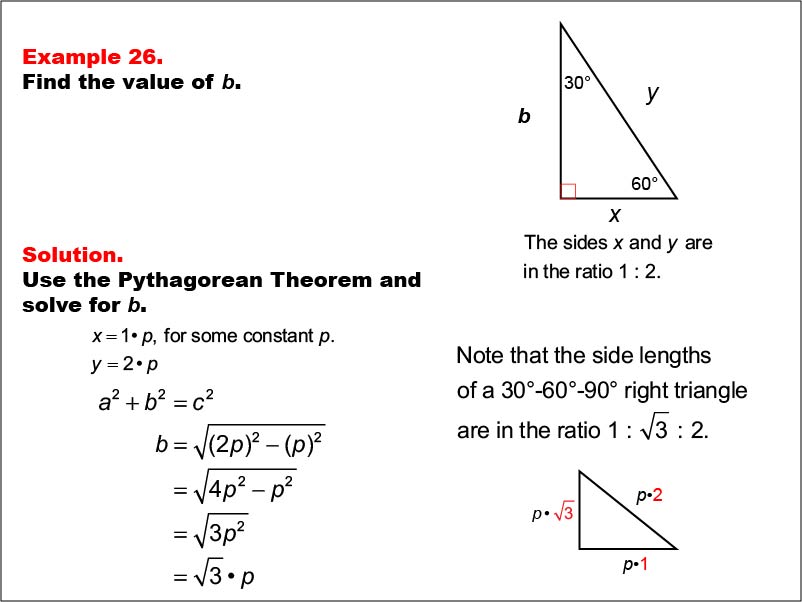
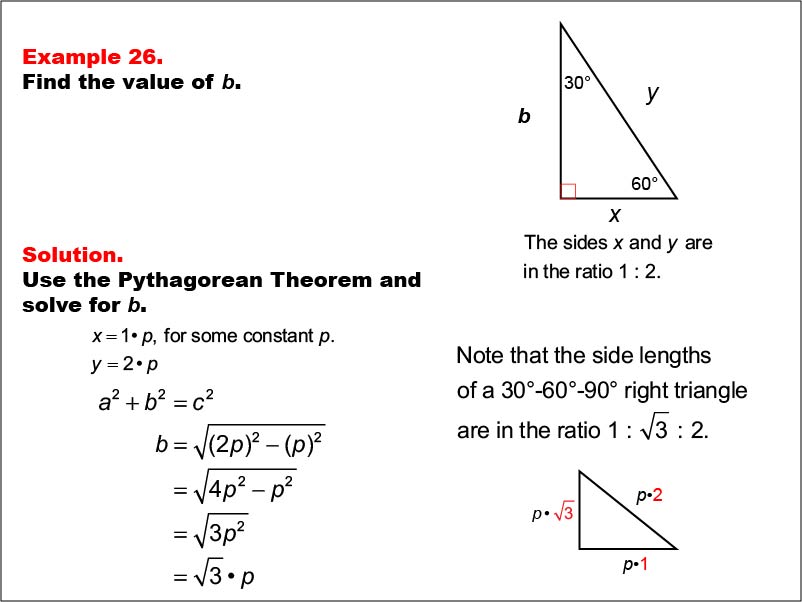
This example features a 30-60-90 right triangle with sides labeled in terms of a constant p. The shorter leg x = p, the hypotenuse y = 2p, and we need to find the longer leg b. Using the Pythagorean Theorem, we calculate that b = √((2p)2 - p2) = √(4p2 - p2) = √(3p2) = √(3) * p. This demonstrates that the side lengths of a 30-60-90 right triangle are always in the ratio 1 : √(3) : 2, regardless of the scale factor p.
This example generalizes the concept of 30-60-90 triangles, showing how the relationship between sides can be expressed algebraically for any scale factor p. It helps students understand the algebraic representation of geometric relationships and how variables can be used to express general rules in mathematics.
Exposing students to multiple worked examples is essential for developing a comprehensive understanding of right triangles and their properties. Each new example provides an opportunity to explore different aspects of right triangles, helping students recognize patterns and build problem-solving skills that can be applied to various geometric scenarios.
Teacher's Script: Now, let's look at our twenty-sixth example. We have a 30-60-90 triangle where the sides are expressed in terms of a constant p. How is this different from our previous examples? That's right, we're looking at a general case. Our task is to express b in terms of p. As we work through this, think about how this relates to the specific 30-60-90 triangles we've seen. How does using variables help us understand the general properties of these special triangles?
For a complete collection of math examples related to Right Triangles click on this link: Math Examples: Right Triangles Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.B.6, CCSS.MATH.CONTENT.8.G.B.7, CCSS.MATH.CONTENT.6.G.A.1 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Geometry • Triangles • Right Triangles |
| Copyright Year | 2013 |
| Keywords | right triangles, leg, hypotenuse |