Display Title
Math Example--Right Triangles-- Example 9
Display Title
Math Example--Right Triangles-- Example 9
Topic
Right Triangles
Description
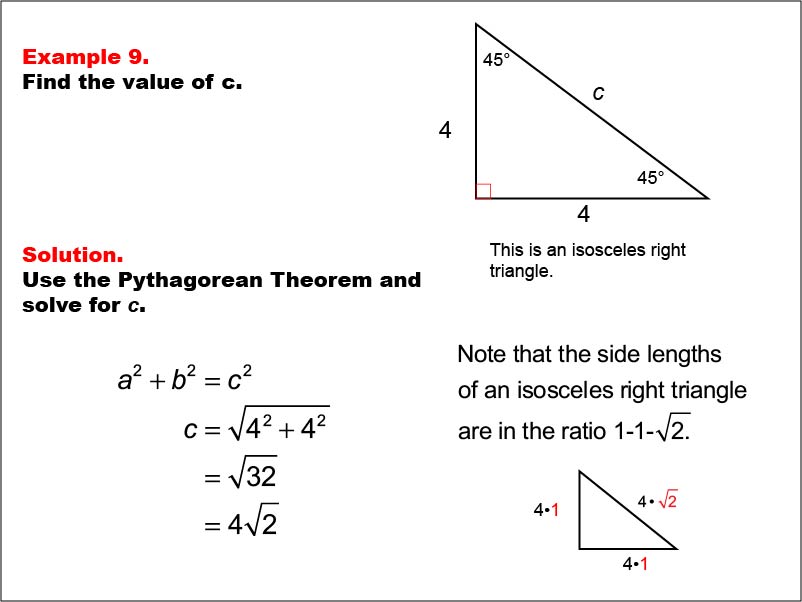
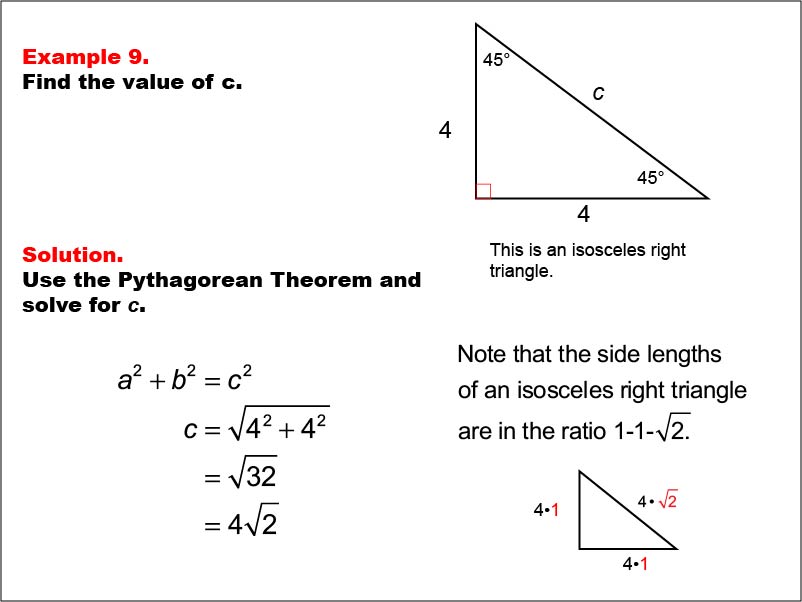
This example presents an isosceles right triangle with both legs measuring 4 units and an unknown hypotenuse c. The angles are 45°, 45°, and 90°. Using the Pythagorean Theorem, we calculate that c = √(42 + 42) = √(32) = 4 * √(2). This demonstrates that the side lengths are in the ratio 1:1:√(2), scaled by a factor of 4.
Isosceles right triangles are important in geometry due to their symmetry and consistent angle measures. This example builds on the previous one, showing how the 1:1:√(2) ratio applies even when the leg length changes, helping students understand the scalability of these relationships.
Exposing students to multiple worked examples is essential for developing a comprehensive understanding of right triangles and their properties. Each new example provides an opportunity to reinforce concepts, recognize patterns, and build problem-solving skills that can be applied to more complex geometric scenarios.
Teacher's Script: Now, let's look at our ninth example. We have another isosceles right triangle, but this time with legs of length 4. What do you notice about the angles? That's right, they're still 45°, 45°, and 90°. Our task is to find the length of the hypotenuse, c. Let's apply the Pythagorean Theorem and see how this result compares to our previous example. Can you predict how the ratio of side lengths might relate to what we found before?
For a complete collection of math examples related to Right Triangles click on this link: Math Examples: Right Triangles Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.B.6, CCSS.MATH.CONTENT.8.G.B.7, CCSS.MATH.CONTENT.6.G.A.1 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Geometry • Triangles • Right Triangles |
| Copyright Year | 2013 |
| Keywords | right triangles, leg, hypotenuse |