
Display Title
Math Example--Sequences and Series--Finding the Explicit Formula of an Arithmetic Sequence: Example 7
Display Title
Math Example--Sequences and Series--Finding the Explicit Formula of an Arithmetic Sequence: Example 7
Finding the Explicit Formula of an Arithmetic Sequence: Example 7
Topic
Sequences and Series
Description
Process for Finding the Explicit Formula
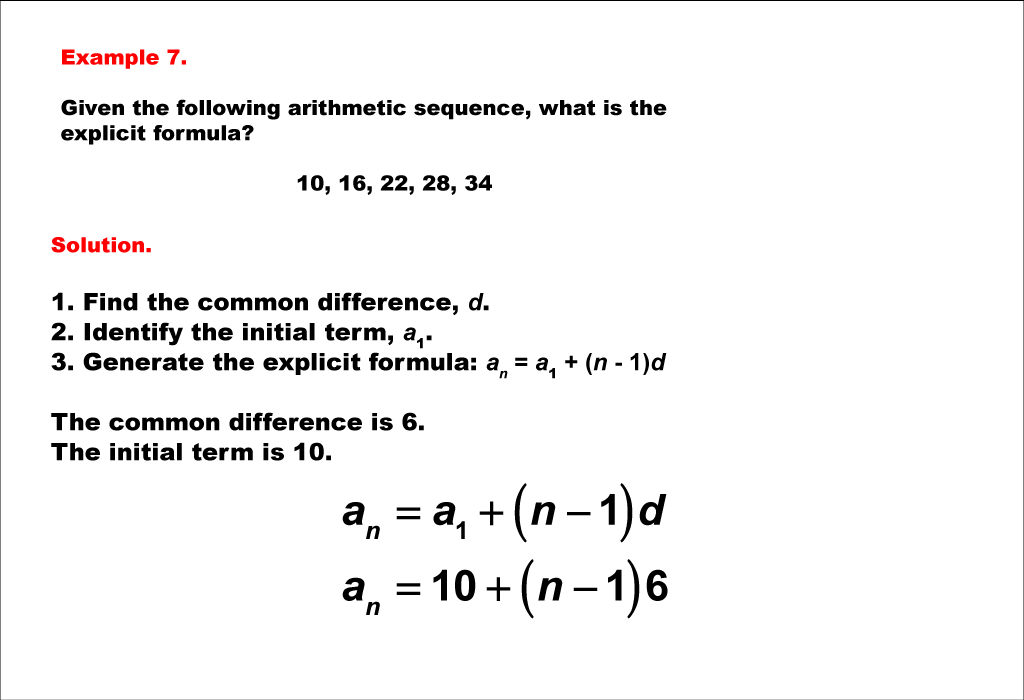
- Identify the First Term: The first term of the sequence is denoted as a1.
- Determine the Common Difference: The common difference d is found by subtracting the first term from the second term.
- Use the Explicit Formula: The nth term of an arithmetic sequence can be found using the formula:
an = a1 + d•( n − 1 )
where an is the nth term, a1 is the first term, d is the common difference, and n is the term number.
Given Sequence
Sequence: [10, 16, 22, 28, 34]
First term (a₁) = 10
Common difference (d) = 16 - 10 = 6
Explicit formula: an = 10 + (n - 1)6 = 6n + 4
For a complete collection of math examples related to Sequences and Series click on this link: Math Examples: Sequences and Series Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.A.2 |
|---|---|
| Grade Range | 9 - 11 |
| Curriculum Nodes |
Algebra • Sequences and Series • Sequences |
| Copyright Year | 2022 |
| Keywords | arithmetic sequences, explicit formula |