
Display Title
Math Example--Solving Equations--Equations with Angles from Parallel Lines Cut by a Transversal--Example 7
Display Title
Equations with Angles from Parallel Lines Cut by a Transversal--Example 7
Topic
Equations
Description
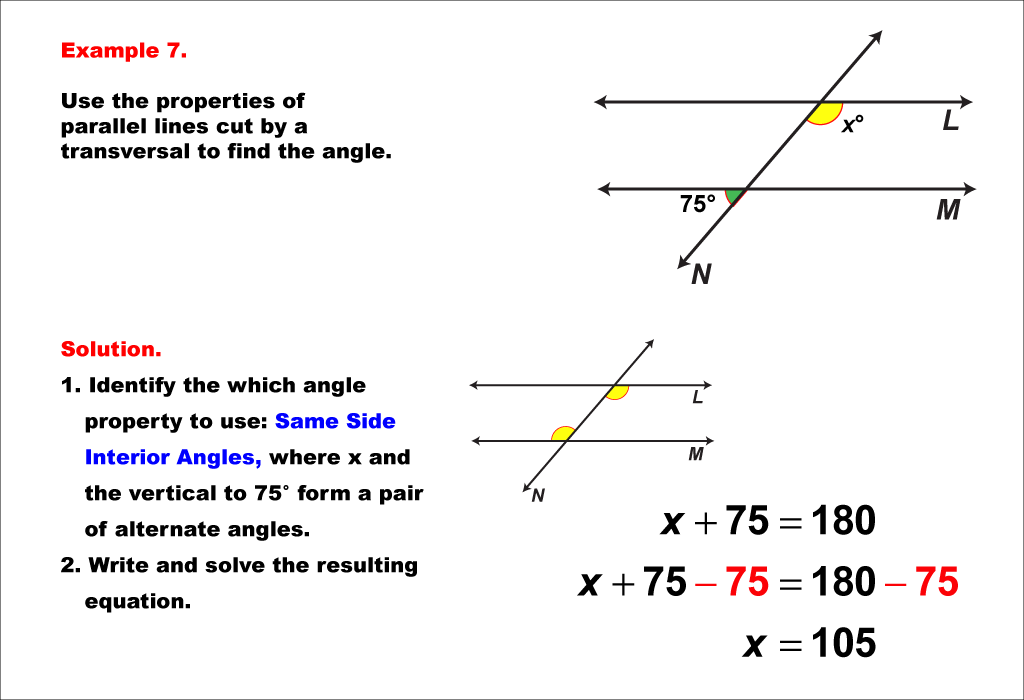
This example focuses on same interior angles formed when parallel lines are cut by a transversal and using the property of vertical angles. The image shows two parallel lines intersected by a transversal, with one same interior angle labeled as x° and the other as 75°. The vertical angle to 75° forms part of a pair of alternate interior angles with x. Same side interior angles are pairs of angles that lie on the same side of the transversal and between the parallel lines. In parallel line configurations, same side interior angles are supplementary. To solve for x, we simply set up the equation: x + 75 = 180. This straightforward relationship is a direct consequence of the parallel nature of the lines. Understanding this property is crucial for solving more complex geometric problems and proofs involving parallel lines and transversals. This example demonstrates how geometric principles can be directly translated into simple algebraic equations, bridging the gap between geometry and algebra.
For a complete collection of math examples related to Equations of Parallel Lines click on this link: Math Examples: Equations of Parallel Lines Collection.
| Common Core Standards | CCSS.MATH.CONTENT.4.G.A.2, CCSS.MATH.CONTENT.8.G.A.5 |
|---|---|
| Grade Range | 4 - 8 |
| Curriculum Nodes |
Geometry • Points and Lines • Parallel Lines • Angles and Planes • Applications of Angles and Planes Algebra • Expressions, Equations, and Inequalities • Applications of Equations and Inequalities |
| Copyright Year | 2021 |
| Keywords | Parallel Lines Cut by a Transversal, parallel lines, transversal, vertical angles, supplementary angles, alternate interior angles, alternate exterior angles |