Display Title
Math Example--Solving Equations--Equations Using the Exterior Angle Theorem--Example 2
Display Title
Equations Using the Exterior Angle Theorem--Example 2
Topic
Equations
Description
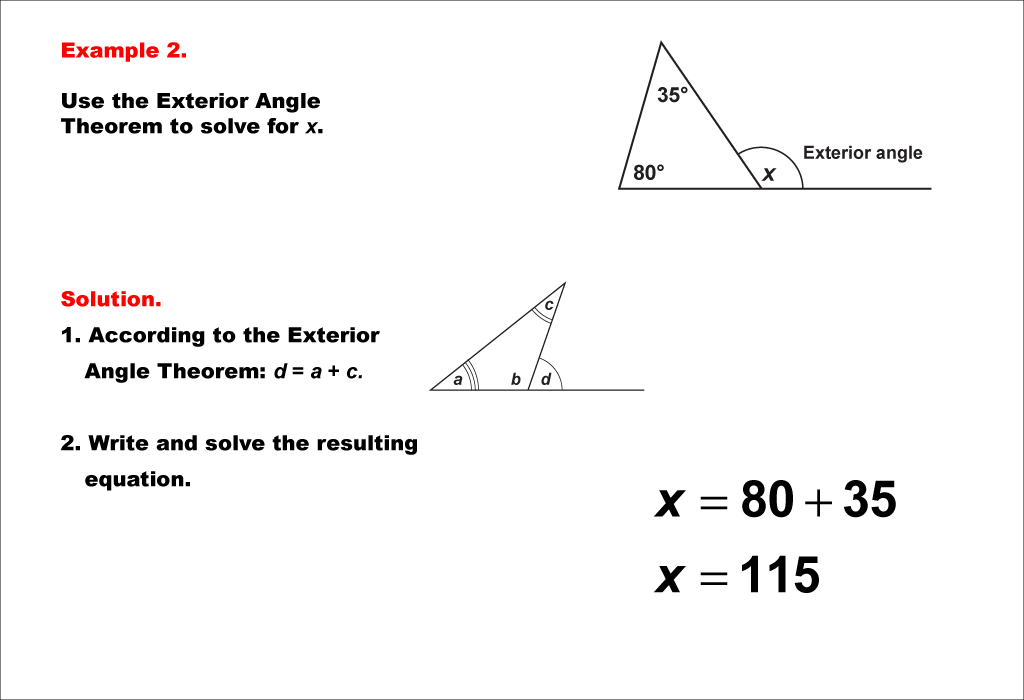
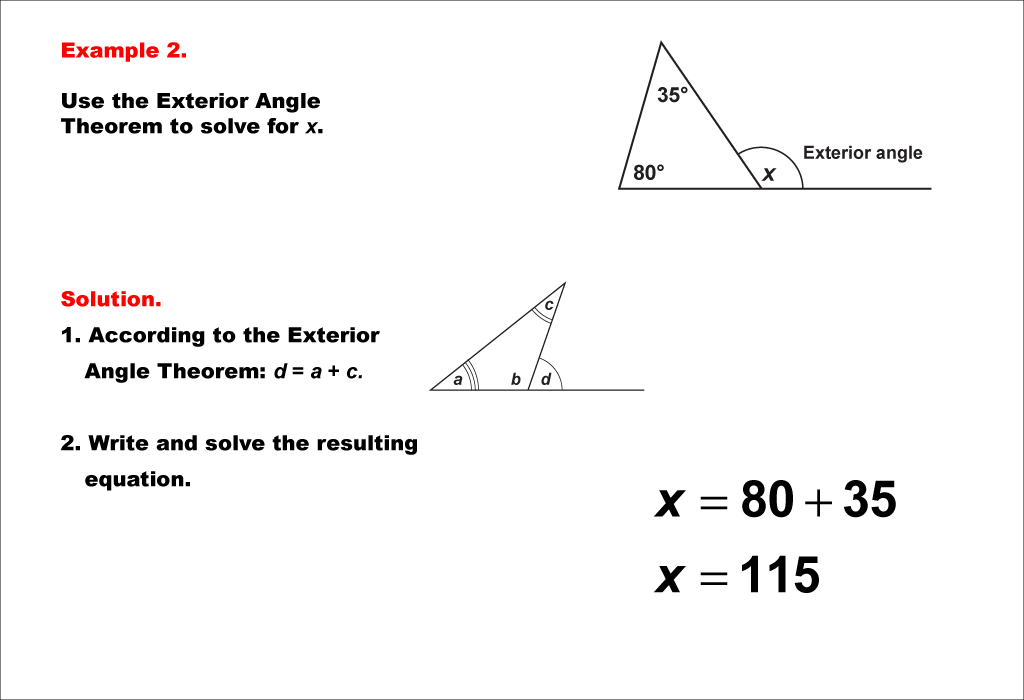
This example illustrates another application of the Exterior Angle Theorem in triangle geometry. Here, we have a triangle with two known interior angles of 80° and 35°, and we need to find the exterior angle x. The Exterior Angle Theorem states that an exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles. In this case, the equation is set up as x = 80° + 35°, which simplifies to x = 115°. This problem demonstrates how the theorem can be used to find an unknown exterior angle when given two interior angles. The methodology for solving such equations involves identifying the known interior angles and applying the theorem directly. This process relies on the fundamental geometric properties of triangles, particularly the relationships between interior and exterior angles. The simplicity of the calculation highlights the power of the theorem in quickly determining unknown angles in triangles. By mastering this concept, students can tackle more complex geometric problems involving triangles and angle relationships. This example serves as a building block for understanding more intricate applications of the Exterior Angle Theorem in various geometric scenarios.
For a complete collection of math examples related to Equations That Use the Exterior Angle Theorem click on this link: Math Examples: Equations That Use the Exterior Angle Theorem Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.A.5 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Geometry • Triangles • Applications of Triangles |
| Copyright Year | 2021 |
| Keywords | exterior angles, exterior angle theorem, supplementary angles |