Display Title
Math Example--Solving Equations--Solving Equations with Angle Measures 2--Example 10
Display Title
Solving Equations with Angle Measures 2--Example 10
Topic
Equations
Description
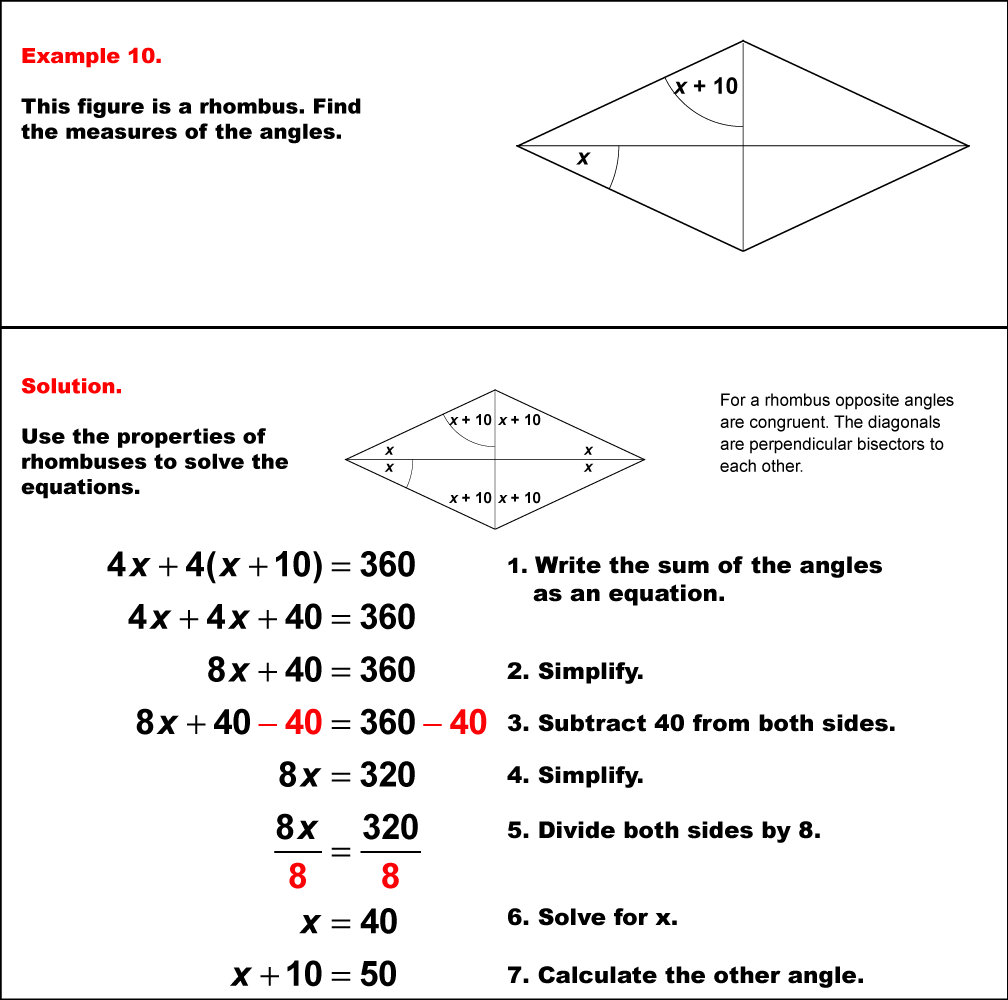
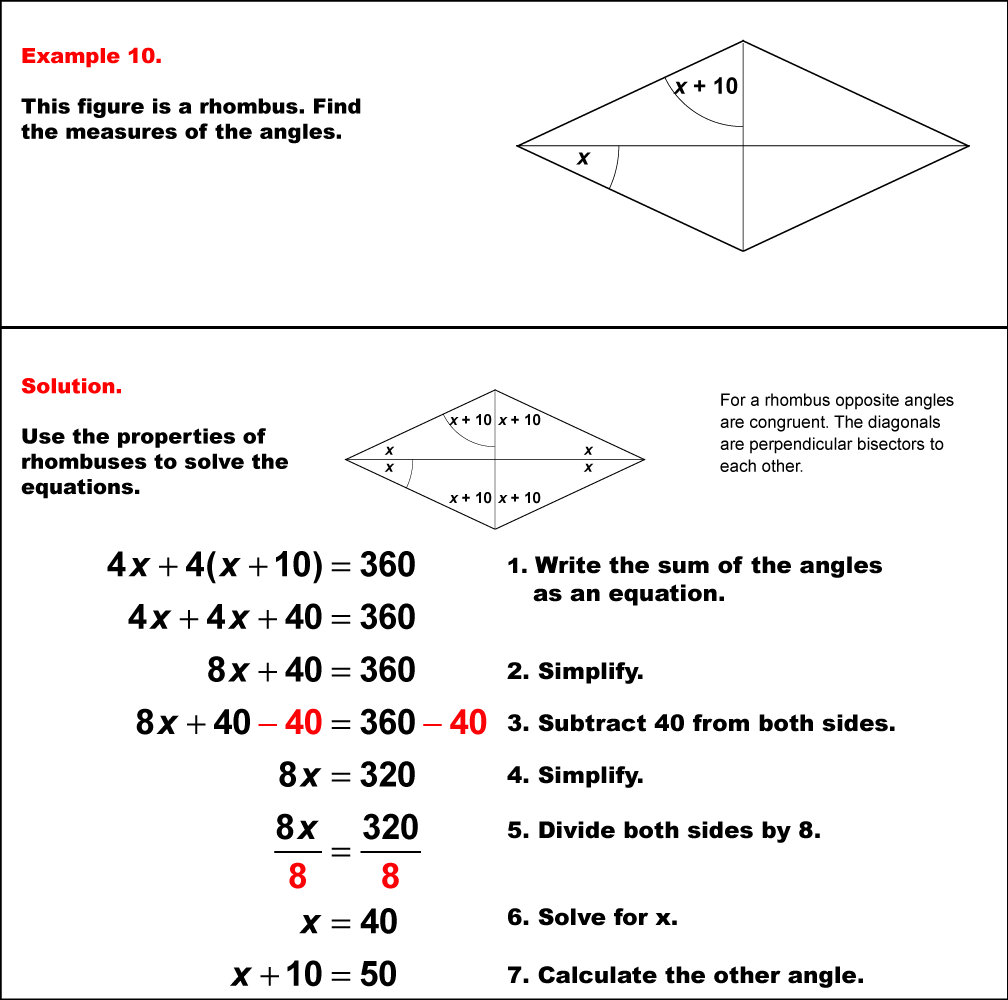
This example illustrates solving equations involving angle measures in a rhombus. The rhombus has angles represented as (2x+20)°, (2x+20)°, 2x°, and 2x°. To solve this problem, we apply two key principles: the sum of angles in a quadrilateral is 360°, and opposite angles in a rhombus are congruent. We can set up the equation: (2x+20)° + (2x+20)° + 2x° + 2x° = 360°. Simplifying, we get 8x° + 40° = 360°. Subtracting 40° from both sides yields 8x° = 320°. Dividing by 8, we find x° = 40°. Therefore, the angles of the rhombus are 100°, 100°, 80°, and 80°. This problem reinforces the properties of a rhombus, particularly that its angles occur in congruent pairs and that adjacent angles are supplementary. It challenges students to translate geometric properties into algebraic expressions and solve equations with multiple terms. Such problems are essential in developing critical thinking skills and deepening understanding of the relationships between algebra and geometry. They prepare students for more advanced mathematical concepts where the ability to model geometric situations algebraically becomes increasingly important.
For a complete collection of math examples related to Solving Equations with Angle Measures click on this link: Math Examples: Solving Equations with Angle Measures Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.A.5, CCSS.MATH.CONTENT.HSG.CO.C.10, CCSS.MATH.CONTENT.7.EE.B.4.A, CCSS.MATH.CONTENT.7.G.B.5 |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Multistep Equations Geometry • Quadrilaterals • Applications of Quadrilaterals |
| Copyright Year | 2020 |
| Keywords | quadrilaterals, equations |