Display Title
Math Example--Solving Equations--Solving Equations with Angle Measures--Example 1
Display Title
Solving Equations with Angle Measures--Example 1
Topic
Equations
Description
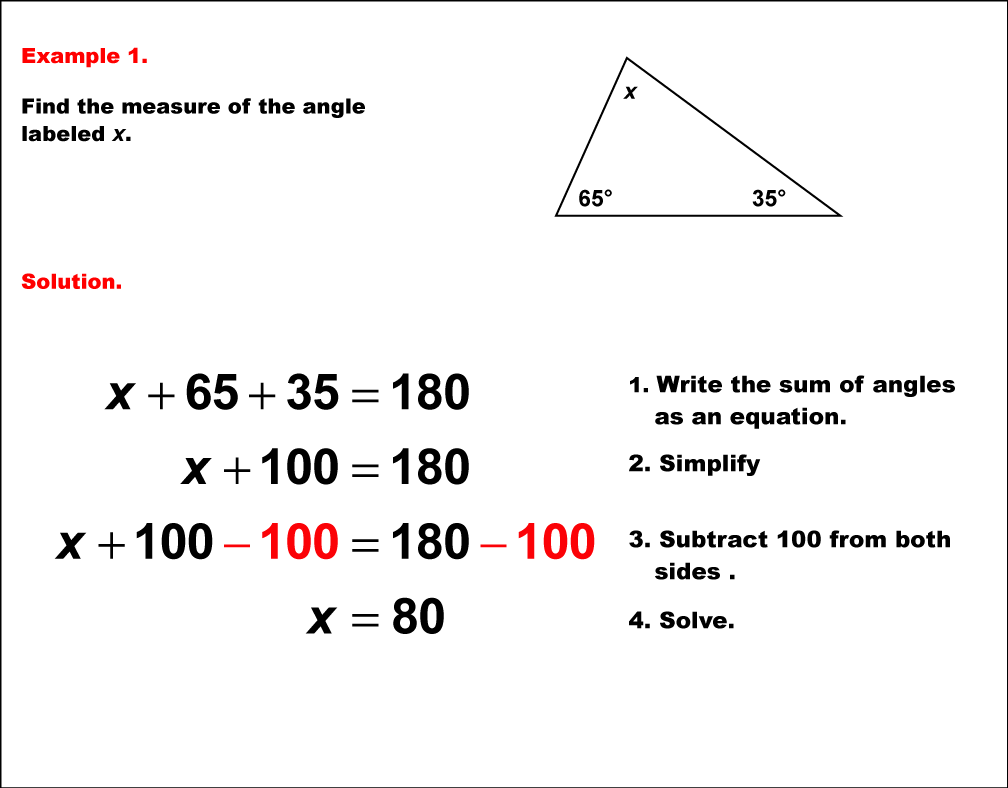
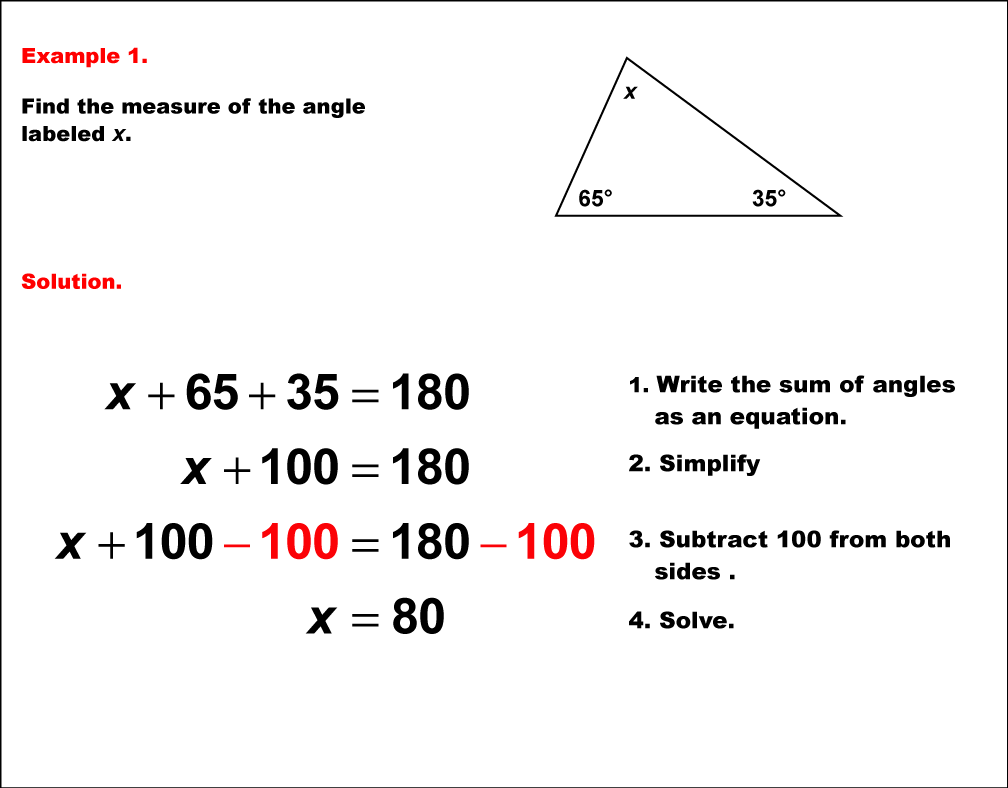
This example demonstrates solving an equation involving angle measures in a triangle. The problem presents a triangle with two known angles (65° and 35°) and one unknown angle represented by x°. To solve this, we use the fundamental property that the sum of angles in a triangle is always 180°. The equation can be set up as 65° + 35° + x° = 180°. Simplifying, we get 100° + x° = 180°. Subtracting 100° from both sides yields x° = 80°. This method of solving angle equations in triangles is crucial in geometry and trigonometry. It allows us to find unknown angles when given information about other angles in the triangle. Such problems help develop logical thinking and reinforce the concept of angle relationships in geometric figures. This particular example is straightforward, requiring only basic algebraic operations to solve for the unknown angle. It serves as an excellent introduction to more complex angle measure problems, laying the groundwork for understanding how to approach and solve equations involving multiple unknown angles or more complex relationships between angles in various geometric shapes.
For a complete collection of math examples related to Solving Equations with Angle Measures click on this link: Math Examples: Solving Equations with Angle Measures Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.A.5, CCSS.MATH.CONTENT.HSG.CO.C.10, CCSS.MATH.CONTENT.7.EE.B.4.A, CCSS.MATH.CONTENT.7.G.B.5 |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Multistep Equations Geometry • Angles and Planes • Applications of Angles and Planes |
| Copyright Year | 2020 |
| Keywords | angles, solving equations, triangles |