Display Title
Math Example--Solving Equations--Solving Equations with Angle Measures--Example 11
Display Title
Solving Equations with Angle Measures--Example 11
Topic
Equations
Description
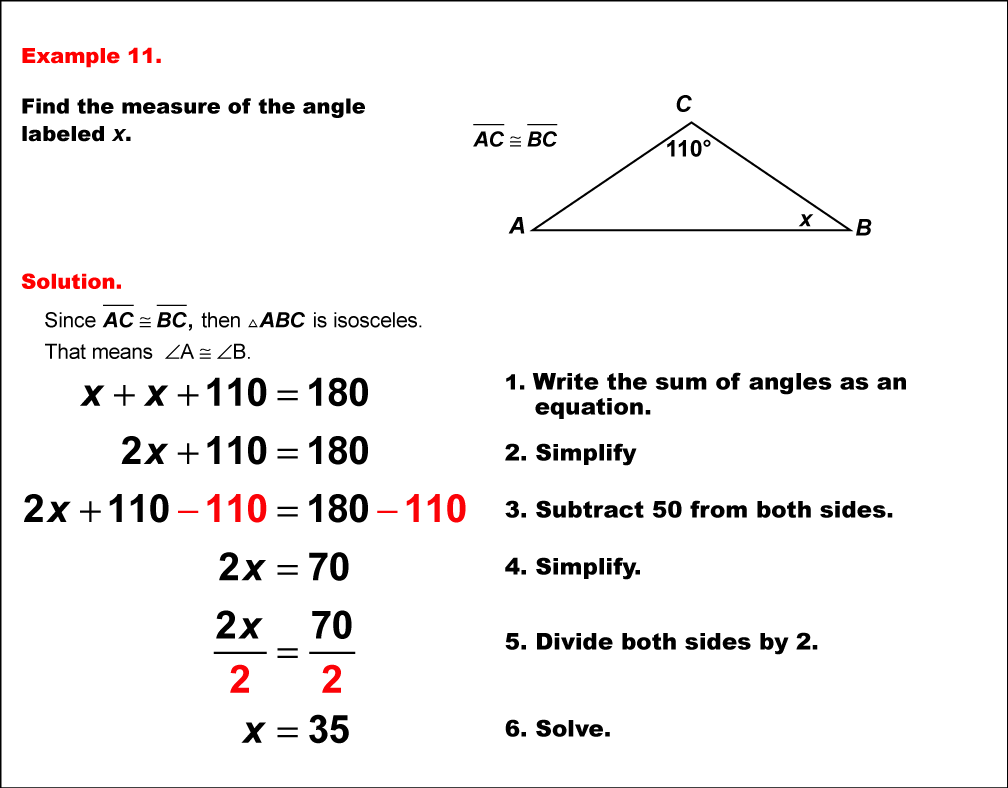
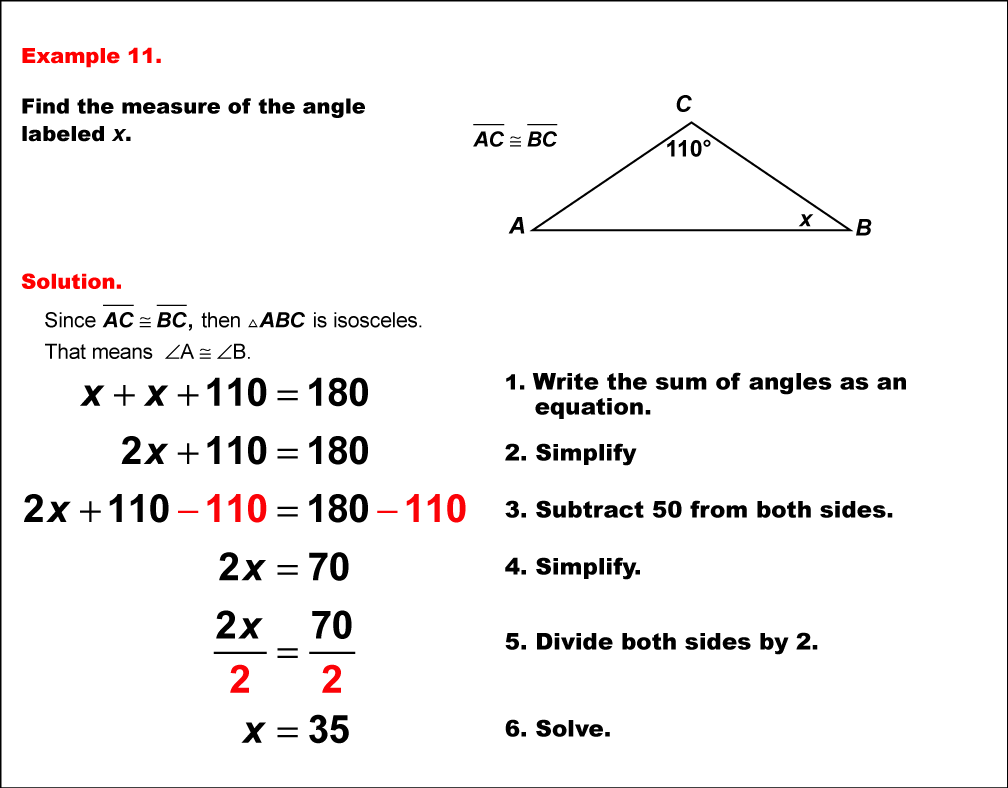
This example illustrates solving an equation involving angle measures in an isosceles triangle with a large base angle. The triangle has two equal angles represented by x° and a known angle of 110°. To solve this problem, we apply the principles that the sum of angles in a triangle is 180° and that an isosceles triangle has two equal angles. The equation can be set up as x° + x° + 110° = 180°. Simplifying, we get 2x° + 110° = 180°. Subtracting 110° from both sides yields 2x° = 70°. Dividing both sides by 2, we find x° = 35°. This problem is particularly interesting because it features an obtuse angle (110°) in the isosceles triangle, which is less common in introductory geometry problems. It helps students understand that isosceles triangles can have a wide range of shapes, from narrow to wide. This example reinforces the concept that the two equal angles in an isosceles triangle are always opposite the two equal sides, regardless of the triangle's overall shape. Such problems are essential in developing a comprehensive understanding of triangle properties and their application in more complex geometric scenarios.
For a complete collection of math examples related to Solving Equations with Angle Measures click on this link: Math Examples: Solving Equations with Angle Measures Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.A.5, CCSS.MATH.CONTENT.HSG.CO.C.10, CCSS.MATH.CONTENT.7.EE.B.4.A, CCSS.MATH.CONTENT.7.G.B.5 |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Multistep Equations Geometry • Angles and Planes • Applications of Angles and Planes |
| Copyright Year | 2020 |
| Keywords | angles, solving equations, triangles |