Display Title
Math Example--Solving Equations--Solving Equations with Angle Measures--Example 14
Display Title
Solving Equations with Angle Measures--Example 14
Topic
Equations
Description
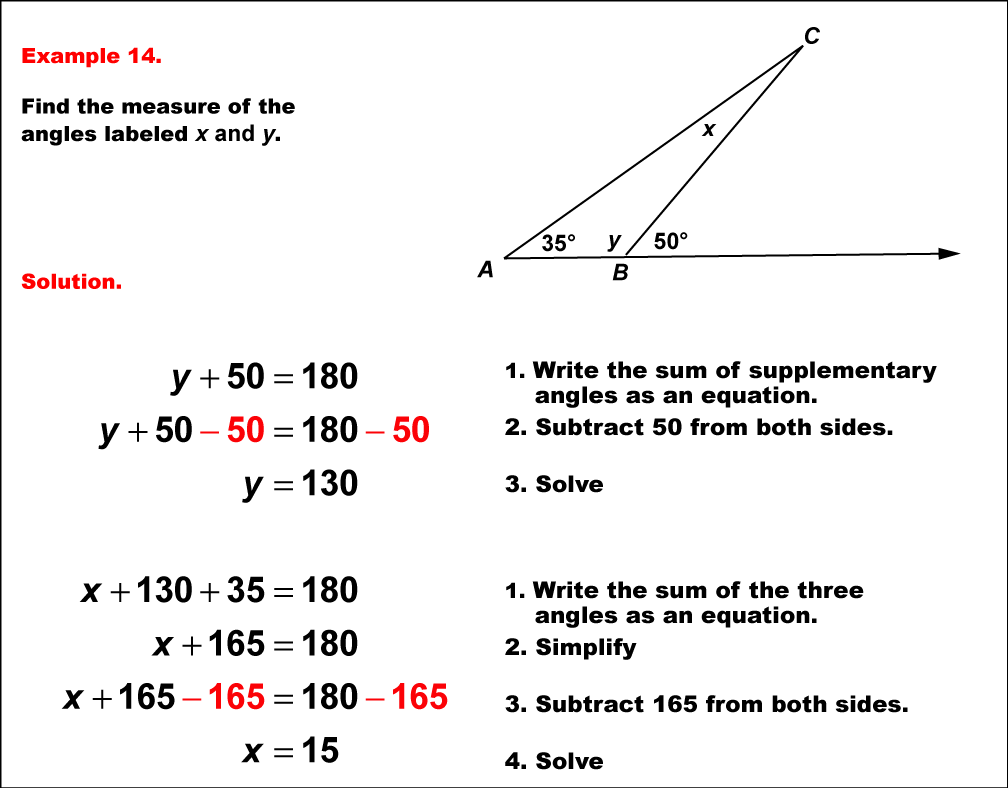
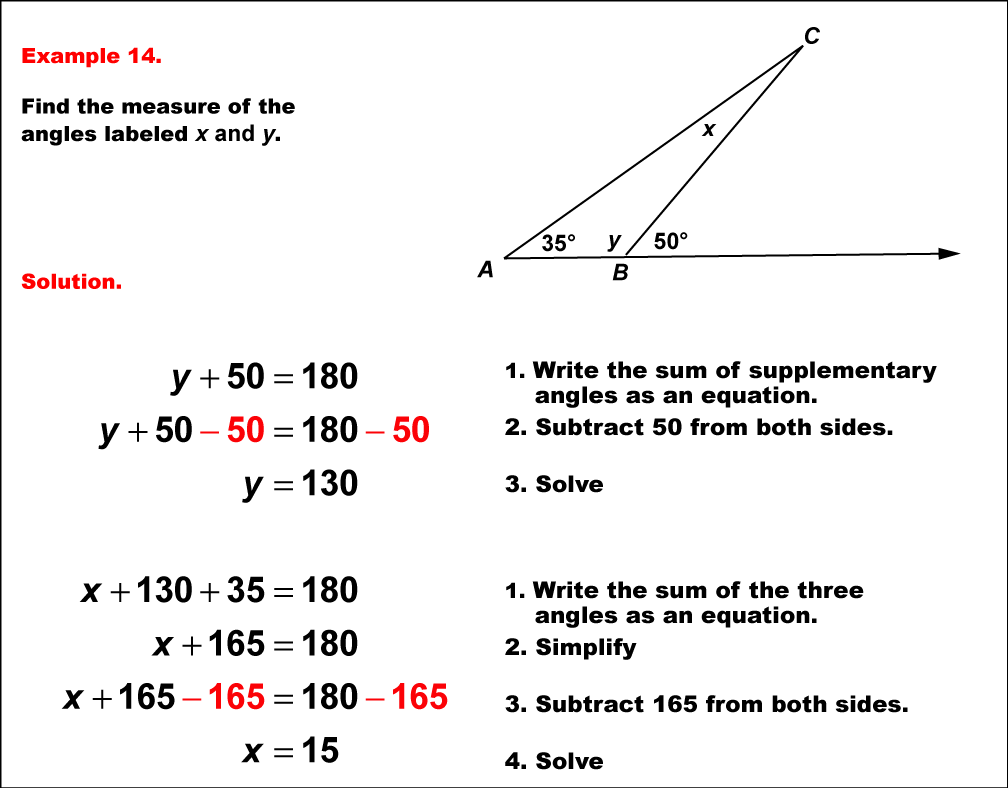
This example illustrates solving equations involving angle measures in a triangle using the properties of supplementary angles. The triangle has one known angle of 35° and two unknown angles represented by x° and y°. We're also given that the angle supplementary to y° is 50°. To solve this problem, we apply two fundamental principles: the sum of angles in a triangle is 180°, and supplementary angles sum to 180°. We begin by determining y°: y° + 50° = 180°, so y° = 130°. Now we can set up the equation for the triangle: 35° + x° + 130° = 180°. Solving for x°, we find x° = 15°. This problem is particularly interesting because it results in a very obtuse angle (130°) within the triangle, which is unusual in typical triangle problems. It challenges students to think beyond conventional triangle shapes and reinforces the idea that the sum of angles in a triangle is always 180°, regardless of the individual angle measures. Such problems are essential in developing critical thinking skills and a deeper understanding of geometric principles, preparing students for more advanced concepts in mathematics.
For a complete collection of math examples related to Solving Equations with Angle Measures click on this link: Math Examples: Solving Equations with Angle Measures Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.A.5, CCSS.MATH.CONTENT.HSG.CO.C.10, CCSS.MATH.CONTENT.7.EE.B.4.A, CCSS.MATH.CONTENT.7.G.B.5 |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Multistep Equations Geometry • Angles and Planes • Applications of Angles and Planes |
| Copyright Year | 2020 |
| Keywords | angles, solving equations, triangles |