Display Title
Math Example--Solving Equations--Solving Equations with Angle Measures--Example 7
Display Title
Solving Equations with Angle Measures--Example 7
Topic
Equations
Description
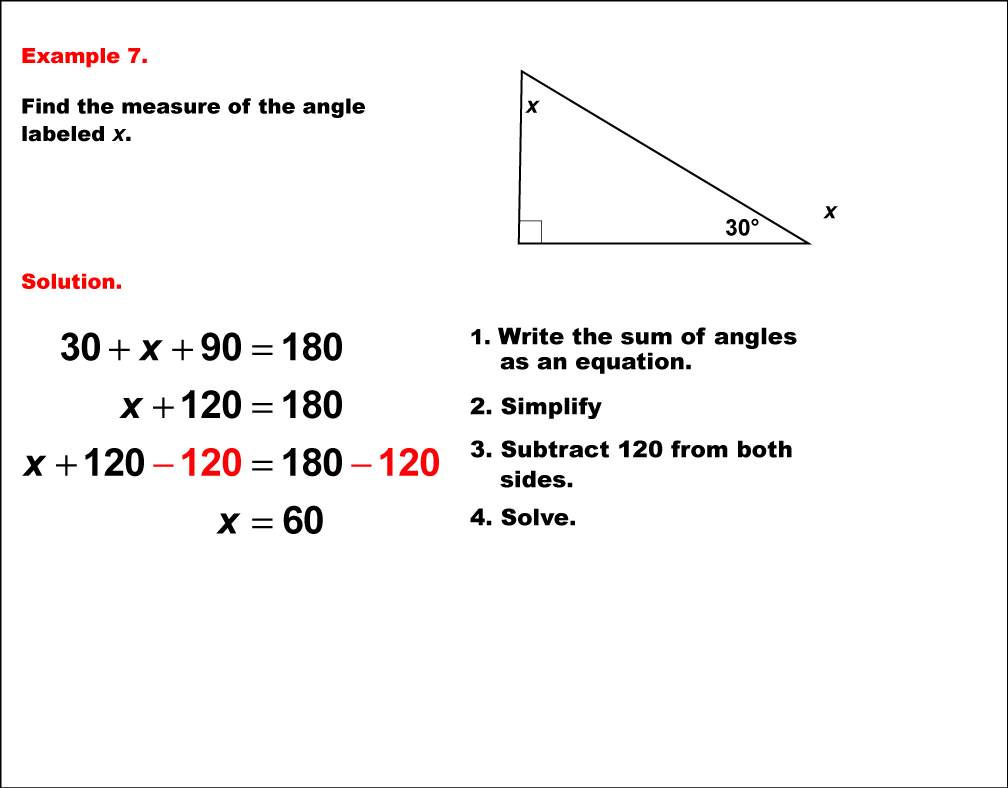
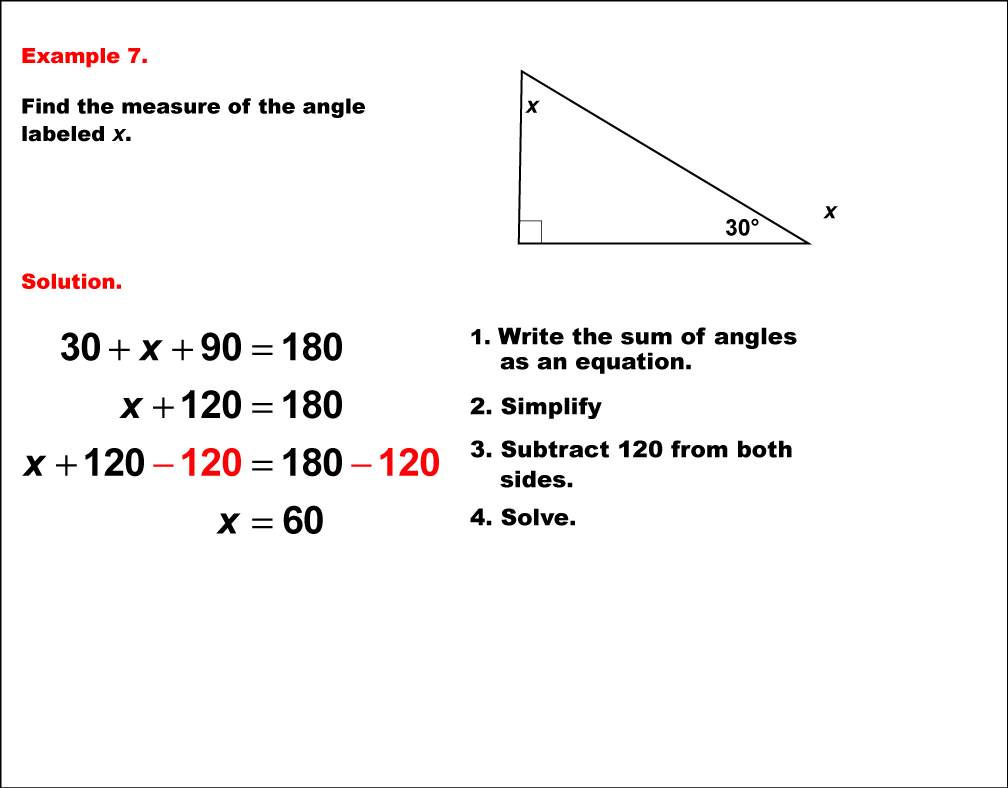
This example illustrates solving an equation involving angle measures in a right triangle. The triangle has two known angles: a right angle (90°) and an acute angle of 30°. The third angle is represented by the variable x°. To solve this problem, we apply the principle that the sum of angles in a triangle is 180°. We can set up the equation: 90° + 30° + x° = 180°. Simplifying, we get 120° + x° = 180°. Subtracting 120° from both sides yields x° = 60°. This problem showcases the properties of a 30-60-90 triangle, a special right triangle with well-known angle measures and side length ratios. It reinforces the concept that in a right triangle, the two acute angles are complementary, meaning they sum to 90°. Such problems are essential in developing a deeper understanding of triangle properties, particularly those of right triangles. They prepare students for more advanced concepts in geometry and trigonometry, including trigonometric ratios and the Pythagorean theorem. This example also helps students recognize special triangle types, which is crucial for solving more complex geometric problems efficiently.
For a complete collection of math examples related to Solving Equations with Angle Measures click on this link: Math Examples: Solving Equations with Angle Measures Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.A.5, CCSS.MATH.CONTENT.HSG.CO.C.10, CCSS.MATH.CONTENT.7.EE.B.4.A, CCSS.MATH.CONTENT.7.G.B.5 |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Multistep Equations Geometry • Angles and Planes • Applications of Angles and Planes |
| Copyright Year | 2020 |
| Keywords | angles, solving equations, triangles |