Display Title
Math Example--Solving Equations--Solving Equations Using Triangle Properties: Example 4
Display Title
Solving Equations Using Triangle Properties: Example 4
Topic
Equations
Description
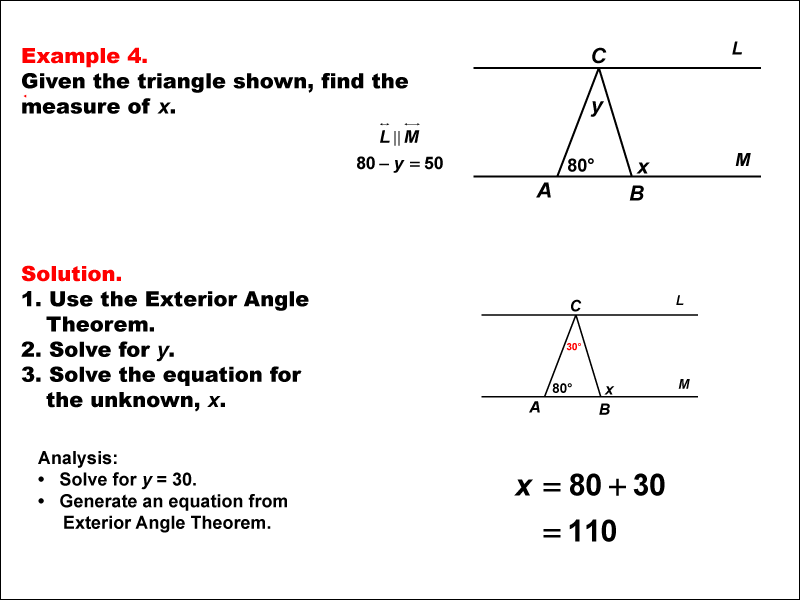
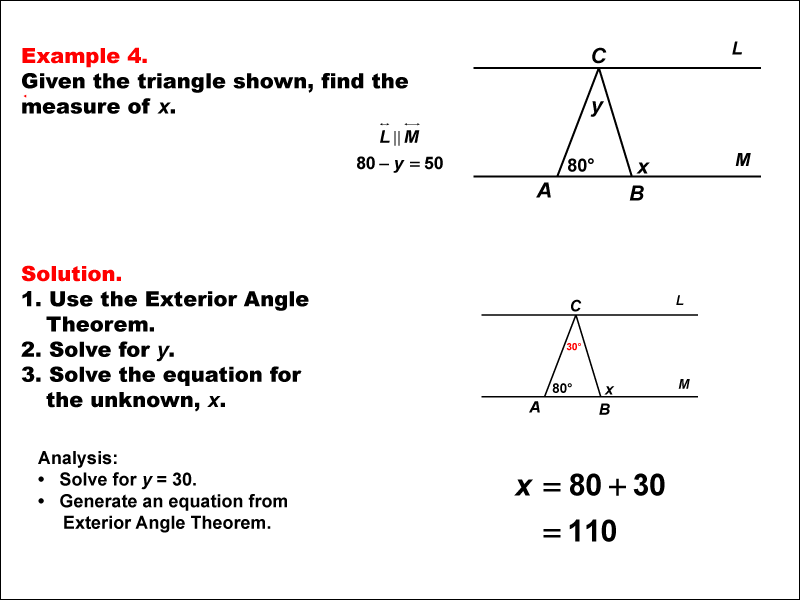
This example demonstrates solving equations using the Exterior Angle Theorem in the context of parallel lines cut by a transversal, two crucial concepts in geometry. The problem presents a triangle with two known interior angles of 80° and y, and an unknown exterior angle x°.
We are also given that 80 - y = 50, which simplifies to y = 30. The goal is to determine the value of x using the properties of triangles and the Exterior Angle Theorem. The Exterior Angle Theorem states that an exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles. Wo we get x = 80 + 30, or x = 110.
Therefore, the exterior angle x is 110°. This solution can be verified by noting that the exterior angle and its adjacent interior angle must sum to 180°. Indeed, 110° + 70° (the third interior angle, which can be calculated as 180° - 80° - 30°) equals 180°. This example illustrates how the Exterior Angle Theorem provides a direct method for solving equations involving triangle angles. It demonstrates the practical application of geometric theorems in problem-solving and reinforces the interconnectedness of algebraic and geometric concepts in mathematics.
For a complete collection of math examples related to Equations Using Triangle Properties click on this link: Math Examples: Equations Using Triangle Properties Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSG.CO.C.10, CCSS.MATH.CONTENT.HSA.CED.A.1 |
|---|---|
| Grade Range | 9 - 11 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Applications of Equations and Inequalities Geometry • Triangles • Applications of Triangles |
| Copyright Year | 2022 |
| Keywords | triangles, solving equations |