Display Title
Math Example: Solving Two-Step Equations: Example 1
Display Title
Math Example: Solving Two-Step Equations: Example 1
Topic
Solving Equations
Description
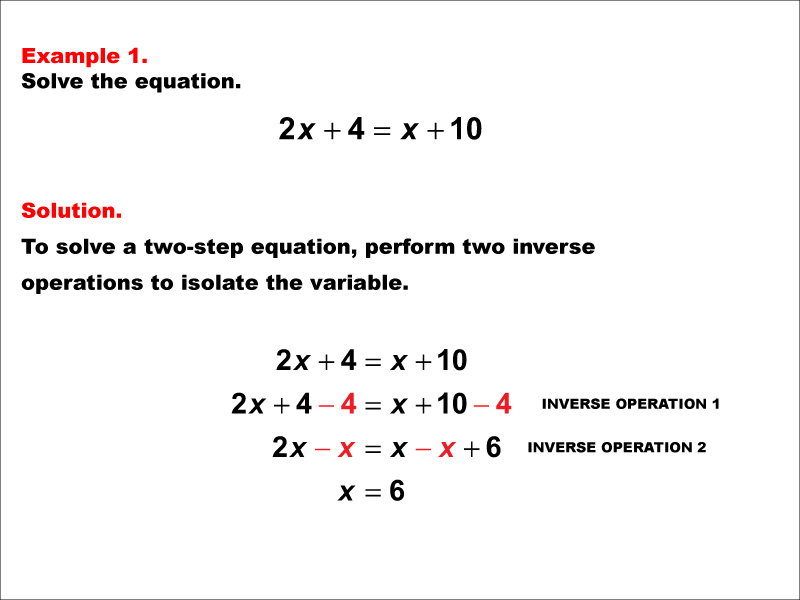
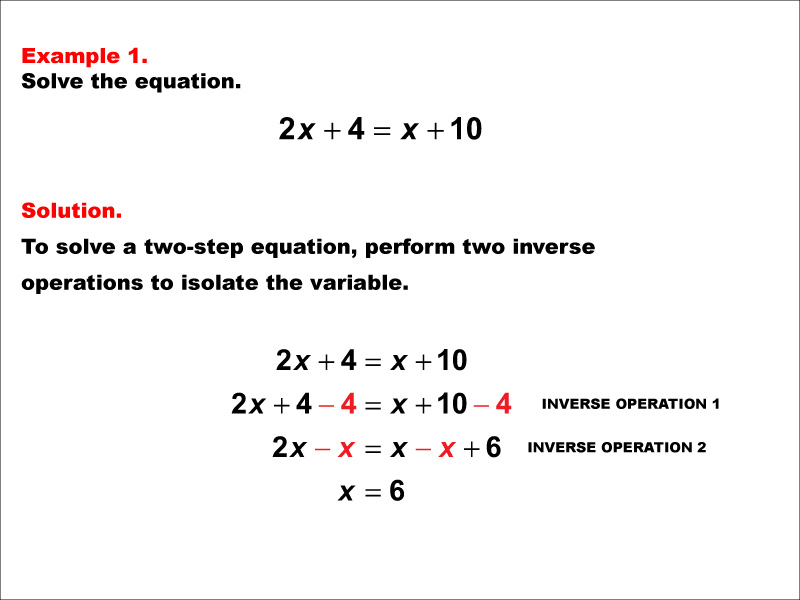
This example demonstrates how to solve the two-step equation 2x + 4 = x + 10. The solution process involves subtracting 4 from both sides of the equation, then subtracting x from both sides to isolate the variable. Through these steps, we find that x = 6.
Solving two-step equations is a fundamental skill in algebra. These equations typically involve two operations to isolate the variable, such as addition/subtraction and multiplication/division. This collection of examples helps teach this topic by presenting a variety of equation types, allowing students to recognize patterns and develop problem-solving strategies.
Seeing multiple worked-out examples is crucial for students to fully grasp the concept of solving two-step equations. Each example reinforces the problem-solving process, helps students identify common patterns, and builds confidence in tackling different equation structures. By working through various scenarios, students develop a more robust understanding of the underlying principles.
Teacher Script: "Let's look at our first example together. We have the equation 2x + 4 = x + 10. Our goal is to isolate x on one side. What do you think our first step should be? Remember, we want to get all the x terms on one side and all the numbers on the other. Take a moment to think about it, and then we'll work through it step-by-step."
For a complete collection of math examples related to Solving Two-Step Equations click on this link: Math Examples: Two-Step Equations Collection.
| Common Core Standards | CCSS.MATH.CONTENT.7.EE.B.4, CCSS.MATH.CONTENT.7.EE.B.4.A, CCSS.MATH.CONTENT.HSA.REI.B.3 |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Solving Two-Step Equations |
| Copyright Year | 2014 |
| Keywords | variable, equation, solution, two-step equation, solving two-step equations, two step equations, solving two step equations |