Math Example: Solving Two-Step Equations: Example 31
Register to SaveSubscribe to DownloadPreview Add to Slideshow
(Subscribers Only)

If you are a subscriber, please log in.

Algebra >> Expressions, Equations, and Inequalities >> Solving Two-Step Equations

Math Example: Solving Two-Step Equations: Example 31

Math Example: Solving Two-Step Equations: Example 31

Solving Two-Step Equations Example 31

Topic

Solving Equations

Description

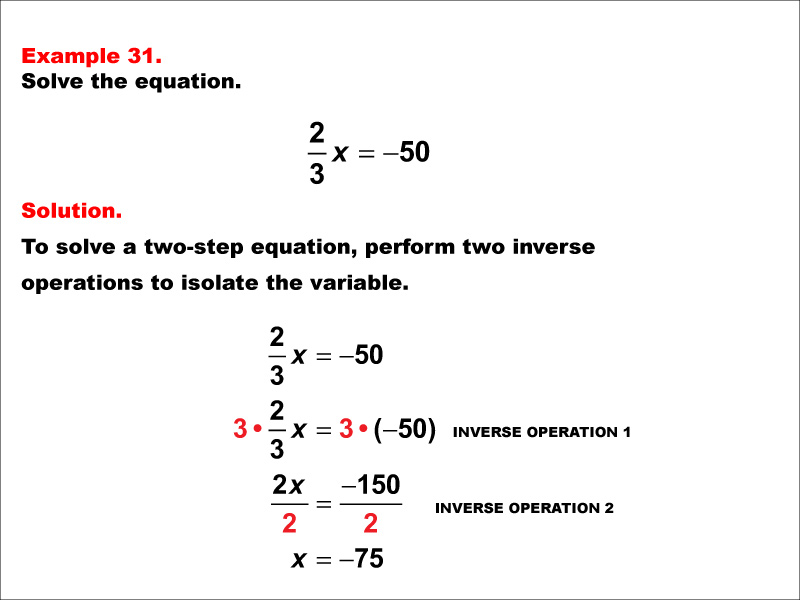
This math example demonstrates solving the equation (2/3)x = -50. The solution involves first multiplying both sides by 3 to eliminate the fraction, then dividing by 2 to isolate x. The final result is x = -75. This example showcases how to handle fractions and negative numbers in two-step equations.

The collection of examples in this set is designed to teach the topic of solving two-step equations by presenting a variety of equation types. These examples cover different combinations of operations, including addition, subtraction, multiplication, and division, as well as equations involving negative numbers, fractions, and parentheses. By working through these diverse problems, students can develop a comprehensive understanding of the steps required to solve two-step equations and learn to apply these skills to various scenarios.

Exposure to multiple worked-out examples is crucial for students to fully grasp the concept of solving two-step equations. Each example reinforces the problem-solving process while introducing slight variations, allowing students to recognize patterns and develop effective problem-solving strategies. This approach helps build confidence and flexibility in tackling different types of equations, preparing students for more complex mathematical challenges.

Teacher Script: "Let's examine this equation: (2/3)x = -50. What do you notice about the left side of the equation? That's right, we have a fraction multiplying our variable x. Our first step is to multiply both sides by 3 to eliminate the fraction. Then, we'll divide by 2 to isolate our variable. Can you work through the steps and tell me what our final answer will be? Remember to be careful with the negative number on the right side of the equation."

For a complete collection of math examples related to Solving Two-Step Equations click on this link: Math Examples: Two-Step Equations Collection.

Solving One-Step Equations

A one-step equation can literally be solved in one step. This is because the equation is written in a form such that an inverse operation is enough to solve it. Here are the different forms a one-step equation can take:

Equation TypeInverse OperationExample
AdditionSubtraction\begin{array}{l} x + 1 = 3\\ x + 1 - 1 = 3 - 1 \leftarrow {\rm{Inverse operation}}\\ x = 2 \end{array}
SubtractionAddition\begin{array}{l} x - 1 = 3\\ x - 1 + 1 = 3 + 1 \leftarrow {\rm{Inverse operation}}\\ x = 4 \end{array}
MultiplicationDivision\begin{array}{l} 2x = 4\\ \frac{{2x}}{2} = \frac{4}{2} \leftarrow {\rm{Inverse operation}}\\ x = 2 \end{array}
DivisionMultiplication\begin{array}{l} \frac{x}{2} = 3\\ \frac{x}{2} \bullet 2 = 3 \bullet 2\\ x = 6 \end{array}

 

With these four basic cases, there are a number of variations, depending on the numbers involved. The simplest types of these equations involve positive whole numbers. But these equations can involve integers and rational numbers.

 

The general form of each of the four types of basic one-step equations are summarized here.

Solving Two-Step Equations

You saw that with one-step equations, the “one step” involved one inverse operation. With two-step equations, there are two inverse operations involved in solving the equation. But this extra step introduces many more types of equations to solve, beyond the basic four from one-step equations.

 

There are 16 basic types of two-step equations that involve different combinations of the four basic operations. 

 AdditionSubtractionMultiplicationDivision
AdditionAAASAMAD
SubtractionSASSSMSD
MultiplicationMAMSMMMD
DivisionDADSDMDD

 

 

These 16 basic examples are summarized in the table below, where we show an example of such an equation using numbers, then followed by a general form of the equation using variables and constant terms.

 

Equation TypeInverse OperationsExampleGeneral Form
Addition and AdditionSubtraction and Subtraction2x + 3 = x + 4ax + b = (a - 1)x + c
Addition and SubtractionSubtraction and Addition3x + 4 = 2x - 5ax + b = (a - 1)x - c
Addition and MultiplicationSubtraction and Division2x + 4 = 8ax + b = c
Addition and DivisionSubtraction and Multiplication\frac{x}{2} + 4 = 8\frac{x}{a} + b = c
Subtraction and AdditionAddition and Subtraction2x - 3 = x + 4ax - b = (a - 1)x + c
Subtraction and SubtractionAddition and Addition2x - 3 = x - 4ax - b = (a - 1)x - c
Subtraction and MultiplicationAddition and Division5x - 7 = 18ax - b = c
Subtraction and DivisionAddition and Multiplication\frac{x}{2} - 4 = 8\frac{x}{a} - b = c
Multiplication and AdditionDivision and Subtraction3(x + 2) = 12a(x + b) = c
Multiplication and SubtractionDivision and Addition3(x - 2) = 15a(x - b) = c
Multiplication and MultiplicationDivision and Division14(15x) = 280a(bx) = c
Multiplication and DivisionDivision and Multiplication12(\frac{x}{5}) = 144a(\frac{x}{b}) = c
Division and AdditionMultiplication and Subtraction\frac{{x + 4}}{5} = 12\frac{{x + a}}{b} = c
Division and SubtractionMultiplication and Addition\frac{{x - 4}}{5} = 12\frac{{x - a}}{b} = c
Division and MultiplicationMultiplication and Division\frac{2}{3}x = 50\frac{a}{b}x = c
Division and DivisionMultiplication and Multiplication\frac{{\frac{x}{4}}}{5} = 30\frac{{\frac{x}{a}}}{b} = c

 

 

These 16 basic two-step equations come in different forms depending on the sign of the numbers and whether the numbers are integers or rational numbers. The simplest types of these equations involve positive whole numbers.

 

Equation TypeGeneral Form
Additionx + a = b,{\rm{ for real numbers }}a{\rm{ and }}b
Subtractionx - a = b,{\rm{ for real numbers }}a{\rm{ and }}b
Multiplicationax = b,{\rm{ for real numbers }}a{\rm{ and }}b,{\rm{ }}a \ne 0
Division\frac{x}{a} = b,{\rm{ for real numbers }}a{\rm{ and }}b,{\rm{ }}a \ne 0

 

Common Core Standards CCSS.MATH.CONTENT.7.EE.B.4, CCSS.MATH.CONTENT.7.EE.B.4.A, CCSS.MATH.CONTENT.HSA.REI.B.3
Grade Range 6 - 9
Curriculum Nodes Algebra
    • Expressions, Equations, and Inequalities
        • Solving Two-Step Equations
Copyright Year 2014
Keywords variable, equation, solution, two-step equation, solving two-step equations, two step equations, solving two step equations