Display Title
Math Example--Systems of Equations--Solving Linear Systems by Elimination: Example 9
Display Title
Math Example--Systems of Equations--Solving Linear Systems by Elimination: Example 9

Topic
Systems of Equations
Description
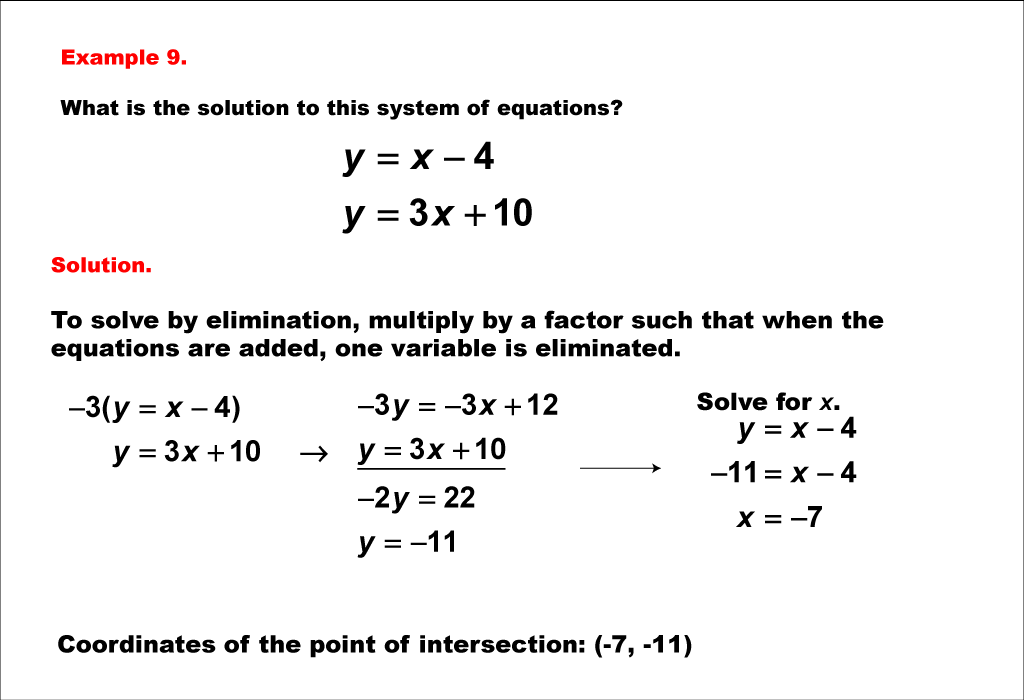
This example solves y = x - 4 and y = 3x + 10 through elimination. Multiplying the first equation by -3 gives -3y = -3x + 12. Adding eliminates x, yielding -2y = 22 or y = -11. Substituting y = -11 into y = x - 4 solves x = -7. The intersection point is (-7, -11).
Linear systems of equations are fundamental in algebra, providing a means to analyze and predict relationships between variables. By exploring worked-out examples, students gain confidence and clarity in solving these systems.
Seeing multiple worked-out examples is crucial for students. It allows them to identify patterns, understand different methods, and build problem-solving strategies.
Teacher Script: Students, let's look at this example together. Notice how we solve the equations step by step. Observe how we find the intersection point, which is the solution to the system. Pay attention to the process and the final verification.
For a complete collection of math examples related to Linear Systems click on this link: Math Examples: Linear Systems Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.EE.C.8 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Linear Systems • Solving Systems of Equations |
| Copyright Year | 2022 |
| Keywords | linear systems |