Display Title
Math Example--Systems of Equations--Solving Linear Systems by Substitution: Example 9
Display Title
Math Example--Systems of Equations--Solving Linear Systems by Substitution: Example 9

Topic
Systems of Equations
Description
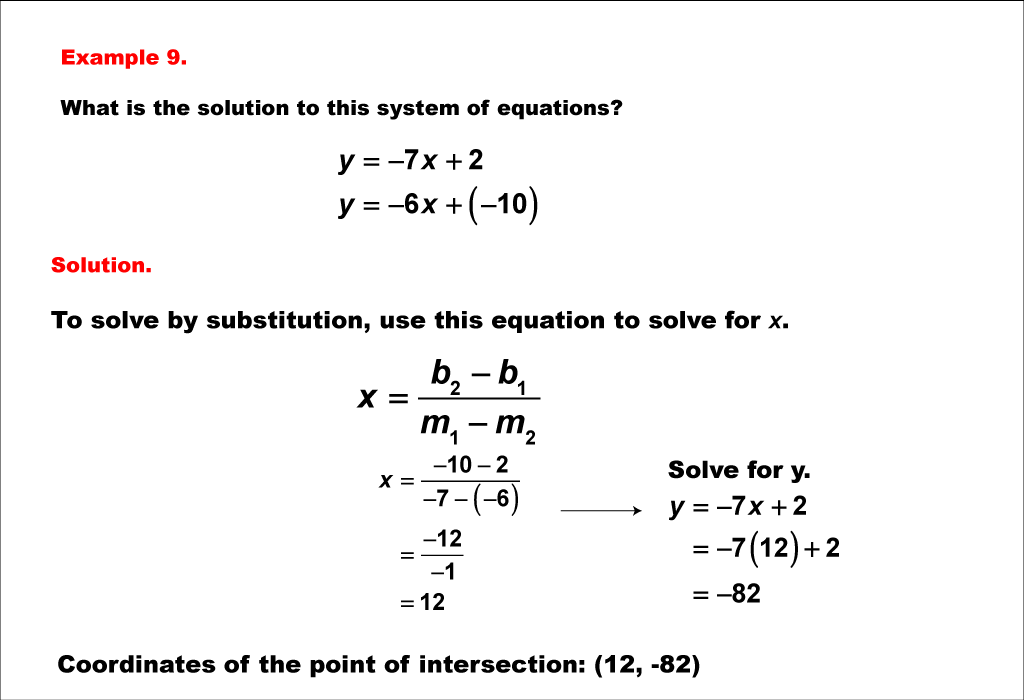
This example demonstrates solving a system of equations: y = -7x + 2 and y = -6x - 10 are solved. The solution involves finding the point of intersection of the two lines represented by these equations. The graph provides a visual representation, while algebraic techniques validate the intersection point. This dual approach helps in understanding the relationships described by the equations.
Systems of equations are foundational in algebra, enabling the determination of values that satisfy multiple conditions simultaneously. Techniques like graphing, substitution, and elimination are commonly used to solve these systems. By analyzing the examples in this collection, students can develop a comprehensive understanding of the concept.
Reviewing multiple worked-out examples helps students grasp diverse problem-solving strategies, recognize patterns, and build their confidence in applying these techniques to different scenarios.
Teacher Script: Let's examine the system of equations y = -7x + 2 and y = -6x - 10 are solved. Observe how the graphs intersect at a specific point, which is the solution to the system. This visual method, combined with algebraic checks, reinforces our understanding of how equations interact.
For a complete collection of math examples related to Linear Systems click on this link: Math Examples: Linear Systems Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.EE.C.8 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Linear Systems • Solving Systems of Equations |
| Copyright Year | 2022 |