edcom-728x90

IXL Ad

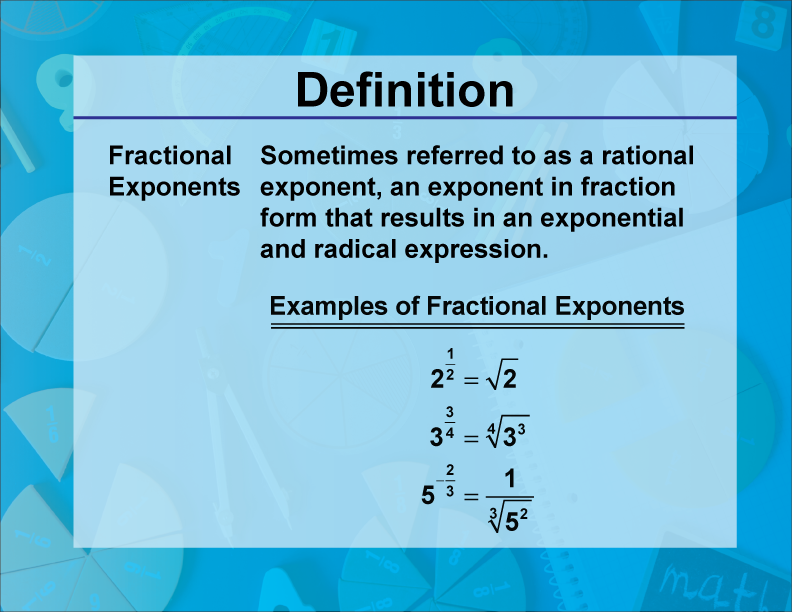
Video Definition 16--Fraction Concepts--Fractional Exponents
Register to SaveSubscribe to DownloadPreview Add to Slideshow
(Subscribers Only)

If you are a subscriber, please log in.

Arithmetic >> Fractions >> Fractions and Mixed Numbers

Video Definition 16--Fraction Concepts--Fractional Exponents

Video Definition 16--Fraction Concepts--Fractional Exponents

This is part of a collection of math video definitions related to to the topic of fractions. Note: The download is an MP4 video.

Common Core Standards CCSS.MATH.CONTENT.3.NF.A.1
Duration 1 minutes
Grade Range 3 - 6
Curriculum Nodes Arithmetic
    • Fractions
        • Fractions and Mixed Numbers
Copyright Year 2024
Keywords fraction