Video Definition 19--Polynomial Concepts--Difference of Cubes (Spanish Audio)
Register to SaveSubscribe to DownloadPreview Add to Slideshow
(Subscribers Only)

If you are a subscriber, please log in.

Algebra >> Polynomials >> Factoring Polynomials

Video Definition 19--Polynomial Concepts--Difference of Cubes (Spanish Audio)

Video Definition 19--Polynomial Concepts--Difference of Cubes (Spanish Audio)

Topic

Polynomials

Description

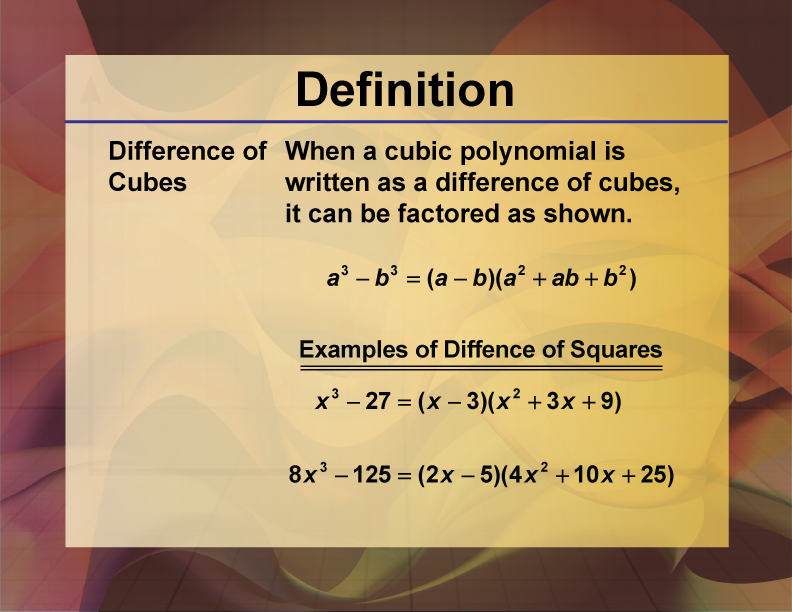
Difference of Cubes: When a cubic polynomial is written as a difference of cubes, it can be factored as a3 - b3 = (a - b)(a2 + ab + b2). Examples: x3 - 27 = (x - 3)(x2 + 3x + 9), 8x3 - 125 = (2x - 5)(4x2 + 10x + 25). Presents a fundamental factoring pattern frequently applied in algebraic problem solving.

This video is highly relevant to the topic of Polynomials. Understanding the underlying concepts can deepen your mathematical reasoning and problem-solving abilities. Topics like these are fundamental to a variety of applications in algebra and beyond.

Teacher's Script: Today, we're going to explore a concept that is foundational to understanding algebra. This video explains Difference of Cubes in a clear and engaging way. Pay attention to the definitions and examples, as they will help you apply these ideas in practice.

For a complete collection of videos related to Polynomials click on this link: Math Video Definitions: Polynomials Collection.

Common Core Standards CCSS.MATH.CONTENT.HSA.APR.A.1, CCSS.MATH.CONTENT.HSA.APR.B.2, CCSS.MATH.CONTENT.HSA.APR.C.5, CCSS.MATH.CONTENT.HSA.APR.C.4, CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSF.IF.C.7.C
Duration 1 minutes
Grade Range 8 - 12
Curriculum Nodes Algebra
    • Polynomials
        • Factoring Polynomials
Copyright Year 2024
Keywords polynomials, monomials, definitions, glossary term, difference of cubes