Video Definition 20--Polynomial Concepts--Sum of Cubes
Register to SaveSubscribe to DownloadPreview Add to Slideshow
(Subscribers Only)

If you are a subscriber, please log in.

Algebra >> Polynomials >> Factoring Polynomials

Video Definition 20--Polynomial Concepts--Sum of Cubes

Video Definition 20--Polynomial Concepts--Sum of Cubes

Topic

Polynomials

Description

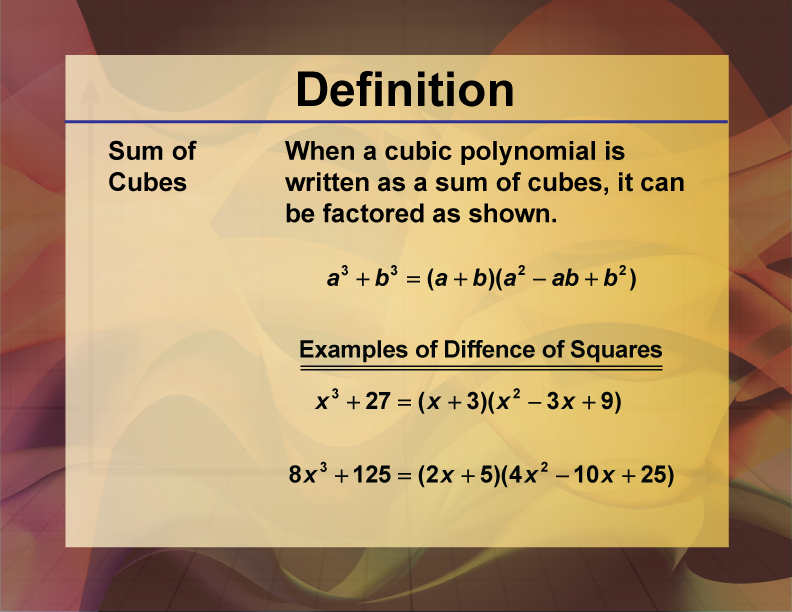
Sum of Cubes: When a cubic polynomial is written as a sum of cubes, it can be factored as a3 + b3 = (a + b)(a2 - ab + b2). Examples: x3 + 27 = (x + 3)(x2 - 3x + 9), 8x3 + 125 = (2x + 5)(4x2 - 10x + 25). Introduces a specific factoring technique for cubic polynomials, aiding in simplification and solving equations.

Polynomials are foundational to algebra, representing expressions with one or more terms. These terms are composed of variables raised to various powers and coefficients. Understanding their structure and function allows mathematicians to model real-world situations effectively. This video elucidates such concepts clearly.

Teacher’s Script: Let's delve into polynomials today! These mathematical expressions allow us to represent complex relationships with simple terms. The video you'll watch explores this idea in depth, focusing on how each term contributes to the polynomial's behavior. Pay attention to the examples provided; they show how to break down and understand these expressions step-by-step.

For a complete collection of videos related to Polynomials click on this link: Math Video Definitions: Polynomials Collection.

Common Core Standards CCSS.MATH.CONTENT.HSA.APR.A.1, CCSS.MATH.CONTENT.HSA.APR.B.2, CCSS.MATH.CONTENT.HSA.APR.C.5, CCSS.MATH.CONTENT.HSA.APR.C.4, CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSF.IF.C.7.C
Duration 1 minutes
Grade Range 8 - 12
Curriculum Nodes Algebra
    • Polynomials
        • Factoring Polynomials
Copyright Year 2024
Keywords polynomials, monomials, definitions, glossary term, sum of cubes