Display Title
Video Definition 26--Rationals and Radicals--Principal Square Root (Spanish Audio)
Display Title
Video Definition 26--Rationals and Radicals--Principal Square Root (Spanish Audio)
Topic
Rationals and Radicals
Description
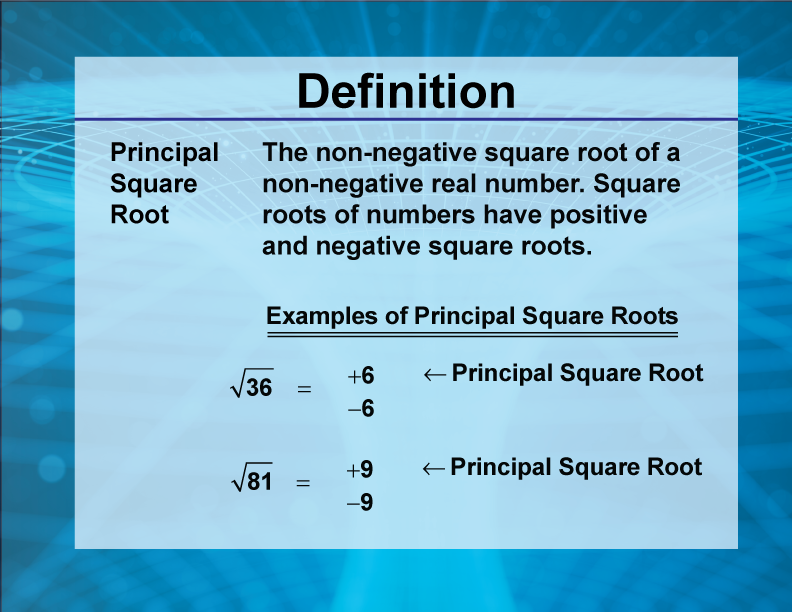
The principal square root is the non-negative square root of a non-negative real number. Examples include √36 = 6 and √81 = 9, excluding their negative counterparts. Clarifies the distinction between principal and negative roots, which is fundamental when solving radical equations.
This video includes Spanish audio and can be used with multilingual learners.
The video explains key concepts related to the topic of Rationals and Radicals. In particular, it delves into the mathematics of the topic, including the principal square root and related principles. These ideas are foundational for understanding Rationals and Radicals more deeply.
Teacher's Script: "Today, we’ll explore an essential concept in mathematics. The video you’re about to see will show us how the principal square root is used to solve problems and why it’s so powerful. As you watch, think about how these ideas connect to what we’ve learned about Rationals and Radicals."
Guión para el Maestro: " Hoy exploraremos un concepto esencial en matemáticas. El video que están a punto de ver nos mostrará cómo se usa principal square root para resolver problemas y por qué es tan poderoso. Mientras lo ven, piensen en cómo estas ideas se conectan con lo que hemos aprendido sobre Rationals and Radicals. "
For a complete collection of videos related to Rationals and Radicals click on this link: Math Video Definitions: Rationals and Radicals (Spanish Audio) Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSA.REI.A.2, CCSS.MATH.CONTENT.HSN.RN.A.1, CCSS.MATH.CONTENT.HSF.IF.C.7 |
|---|---|
| Duration | 1 minutes |
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Radical Expressions and Functions • Radical Expressions |
| Copyright Year | 2024 |
| Keywords | radicals, radical expressions, rational numbers, rational expressions, definitions, glossary term, rational functions |