Display Title
Video Definition 4--3D Geometry--Cavalieri's Principle
Display Title
Cavalieri's Principle
Topic
3D Geometry
Definition
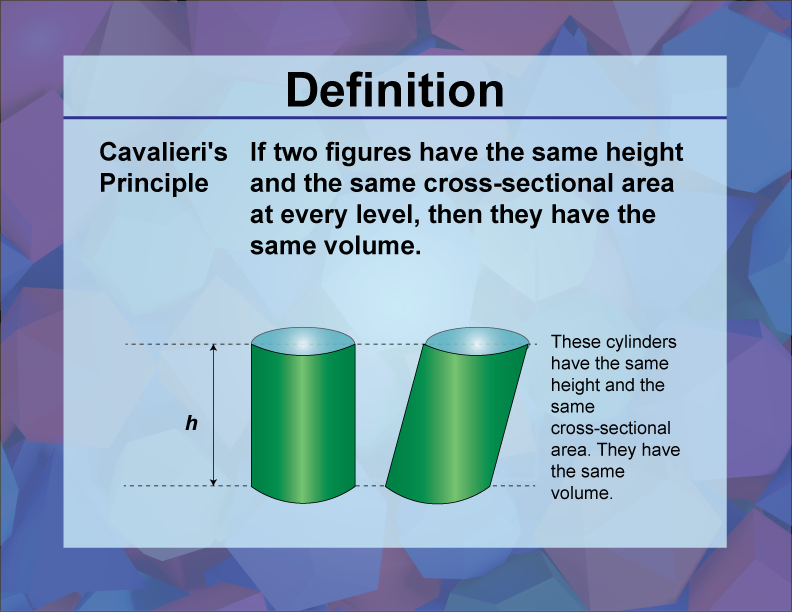
Cavalieri's Principle states that two solids with equal heights and equal cross-sectional areas at every level have equal volumes.
Description
Cavalieri's Principle is a powerful tool in geometry for comparing volumes of solids. It is widely used in calculus and mathematical modeling to simplify complex volume calculations. The principle is essential in understanding the equivalence of volume in different shapes, such as a cylinder and a prism with the same height and cross-sectional area. In math education, Cavalieri's Principle helps students develop a deeper understanding of volume and spatial reasoning. Teachers can illustrate this principle by using visual aids and experiments, showing how different shapes can have the same volume despite their appearance. An example of Cavalieri's Principle is comparing a cylinder and a stack of coins; if the coins are stacked to the same height as the cylinder and have the same cross-sectional area, their volumes are equal.

For a complete collection of terms related to 3D Geometry click on this link: 3D Geometry Video Collection.
| Common Core Standards | CCSS.MATH.CONTENT.5.MD.C.3 |
|---|---|
| Duration | 1 minutes |
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Geometry • 3D Geometry • 3-Dimensional Figures |
| Copyright Year | 2024 |
| Keywords | three-dimensional geometry, 3d Geometry, defnitions, glossary term, Cavalieri's Principle |