Lesson Plan: Introduction to Ratios and Unit Rates

Lesson Objectives

  • Introduce ratios and unit rates
  • Represent ratios
  • Calculate unit rates
  • Solve problems involving ratios and rates

This lesson plan is designed for one 50-minute class period but could be extended to two periods for more in-depth practice and discussion.

TEKS Standards

  • 7.4A: Represent constant rates of change in various forms
  • 7.4B: Calculate unit rates from rates
  • 7.4D: Solve problems involving ratios, rates, and percents

Prerequisite Skills

  • Basic fraction knowledge
  • Understanding of division
  • Graphing linear functions

Key Vocabulary

  • Ratio
  • Unit rate
  • Equivalent ratios
  • Conversion factor
  • Linear function
  • Slope

Warm-up Activity (10 minutes)

Present students with the following scenario:

A car travels 180 miles in 3 hours.

Ask students to:

  • Calculate the speed of the car in miles per hour
  • Determine how far the car would travel in 5 hours at this rate
  • Convert the speed to kilometers per hour (use 1 mile ≈ 1.60934 km)

Discuss how these calculations relate to unit rates and conversions between different units.

Teach (30 minutes)

Definitions

  • Ratio: A comparison between two quantities, often expressed as a:b, a to b, or a/b.
  • Unit rate: A ratio where the second term is 1, used to compare quantities per unit.
  • Equivalent ratios: Ratios that represent the same relationship between quantities.
  • Conversion factor: A ratio used to convert one unit of measurement to another.
  • Linear function: A function that produces a straight line when graphed, often in the form y = mx + b.
  • Slope: The steepness of a line, calculated as the change in y divided by the change in x.

Use this slide show to review these and related definitions that are used in this lesson:

https://www.media4math.com/library/slideshow/ratio-and-rate-definitions

Instruction

Use this slide show to introduce rates and unit rates:

https://www.media4math.com/library/slideshow/introduction-rates

As time allows show the following three examples of rates. 

Example 1: Physics - Speed Calculation (Finding the Unit Rate)

A cyclist travels 36 miles in 2.4 hours.

Question: What is the cyclist's speed in miles per hour?

Solution:

Unit rate (speed) = Distance ÷ Time
                      = 36 miles ÷ 2.4 hours
                      = 15 miles per hour

The cyclist's speed is 15 miles per hour.

Example 2: Business - Production Planning (Calculating and Using Unit Rate)

A factory produces 540 widgets in a 12-hour shift.

Questions:

  1. What is the production rate in widgets per hour?
  2. How many widgets can the factory produce in an 8-hour shift at this rate?

Solution:

a) Unit rate = Total widgets ÷ Total hours
                 = 540 widgets ÷ 12 hours
                 = 45 widgets per hour
b) Production in 8 hours = 45 widgets/hour × 8 hours = 360 widgets

The factory produces 45 widgets per hour and can produce 360 widgets in an 8-hour shift.

Example 3: Engineering - Fuel Efficiency (Graphing and Calculating Unit Rate)

A car's fuel consumption can be represented by the following data points. For each gallon of gas used, the car travels a certain number of miles.

GallonsMiles
260
5150
8240

Questions:

  1. Graph these points on a coordinate plane with gallons on the x-axis and miles on the y-axis.
  2. Draw a line through these points.
  3. Calculate the slope of this line.
  4. What does the slope represent, and what are its units?

Solution:

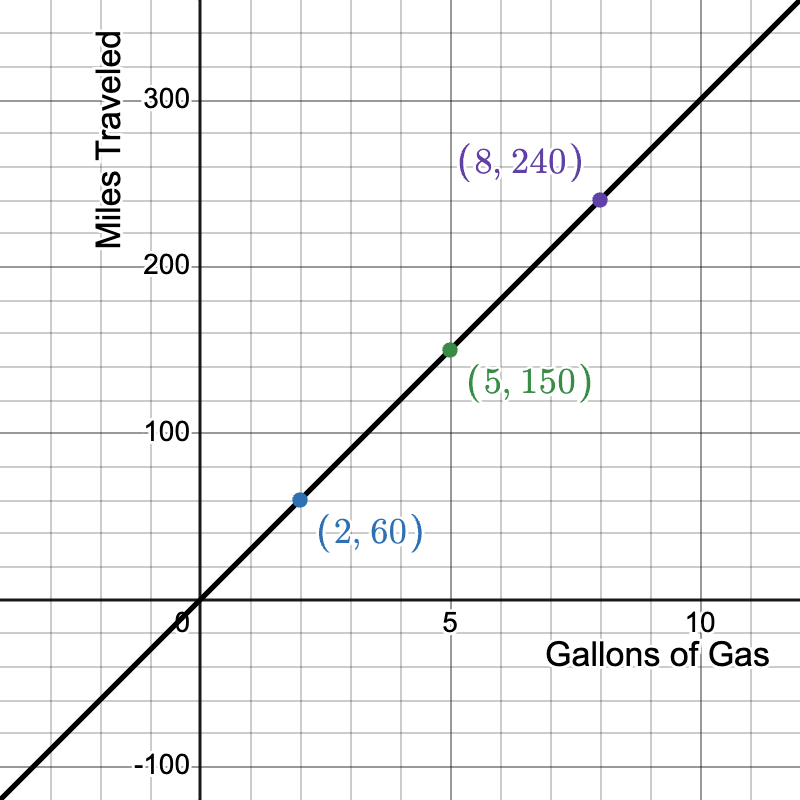
Coordinate Graph

 

Slope = Change in y ÷ Change in x
             = (240 - 60) ÷ (8 - 2)
             = 180 ÷ 6
             = 30
The slope represents the fuel efficiency of the car. Its units are miles per gallon (mpg).

The car's fuel efficiency is 30 miles per gallon.

Review (5 minutes)

Use this video to review unit rates:

https://www.media4math.com/library/1797/asset-preview

 If time allows, work through another detailed example with the class:

A coffee shop sells 180 cups of coffee in 3 hours.

Questions:

  1. What is the unit rate of coffee sales per hour?
  2. How many cups of coffee can they expect to sell in an 8-hour day at this rate?
  3. Graph the relationship between hours and cups of coffee sold from 0 to 10 hours.
  4. Using the graph, estimate how long it would take to sell 300 cups of coffee.

Guide students through each step, encouraging participation and discussion. If necessary, refer to these grade 6 lessons on ratios for additional review:

Assess (5 minutes)

Administer the 10-question quiz to gauge understanding.

Quiz

  1. If a car travels 240 miles in 4 hours, what is its speed in miles per hour?

     
  2. Convert 65 miles per hour to kilometers per hour. (1 mile ≈ 1.60934 km)

     
  3. A recipe calls for 2/3 cup of flour for every 1/4 cup of sugar. How much flour is needed for 1 cup of sugar?

     
  4. If 18 widgets are produced in 1.5 hours, what is the production rate in widgets per hour?

     
  5. A delivery truck uses 12 gallons of gas to travel 180 miles. What is its fuel efficiency in miles per gallon?

     
  6. Convert 30 miles per gallon to kilometers per liter. (1 gallon ≈ 3.78541 liters, 1 mile ≈ 1.60934 km)

     
  7. If 5/8 kg of rice costs \$2.40, what is the price per kg?

     
  8. A printer prints 80 pages in 2 minutes. How many pages can it print in 7 minutes?

     
  9. If 3/4 cup of flour weighs 90 grams, how many grams does 1 cup of flour weigh?

     
  10. A train travels 450 km in 3 hours. What is its speed in meters per second?

Answer Key

  1. 60 mph
  2. 104.6 km/h
  3. 2 2/3 cups of flour
  4. 12 widgets per hour
  5. 15 mpg
  6. 12.75 km/L
  7. \$3.84 per kg
  8. 280 pages
  9. 120 grams
  10. 41.67 m/s

 

Purchase the lesson plan bundle. Click here.