 Understanding Ratios, Proportional Relationships, and Rational Numbers
Understanding Ratios, Proportional Relationships, and Rational Numbers
This lesson plan can be completed in two 50-minute class periods.
Lesson Objectives
- Understand the concept of slope
- Graph proportional relationships
- Interpret unit rate as slope
- Use data to determine slope and y-intercept
- Represent linear relationships in various forms
TEKS Standards
- 8.4A: Use similar right triangles to understand slope in y = mx + b.
- 8.4B: Graph proportional relationships, interpreting unit rate as slope.
- 8.4C: Use data to determine rate of change or slope and y-intercept.
- 8.5A: Represent linear proportional situations with tables, graphs, and equations.
- 8.5B: Represent linear non-proportional situations with tables, graphs, and equations.
- 8.5I: Write an equation y = mx + b to model linear relationships.
Prerequisite Skills
- Basic understanding of ratios
- Graphing on a coordinate plane
- Knowledge of fractions and decimals
Key Vocabulary
- Ratio
- Proportion
- Constant of proportionality
- Unit rate
- Rational number
- Irrational number
- Repeating decimal
Warm-up Activity (15 minutes)
Ratio Review with Positive and Negative Numbers:
- Students are given a set of paired positive numbers and asked to express them as ratios in simplest form:
- 8 and 12
- 6 and 9
- 15 and 25
- 7 and 21
- Students consider ratios with negative numbers and discuss what it means for a ratio to have a negative number:
- -8 and 12
- 6 and -9
- -15 and -25
- 7 and -21
- Discuss how the signs of the numbers affect the ratio and what it means in real-world scenarios (e.g., profit/loss, temperature changes).
Teach (60 minutes)
Definitions (15 minutes)
- Ratio: A comparison of two quantities expressed as a fraction or using the word "to"
- Proportion: An equation stating that two ratios are equal
- Constant of proportionality: The constant ratio between two proportional quantities
- Unit rate: A rate expressed as a quantity per one unit of another quantity
- Rational number: A number that can be expressed as the ratio of two integers
- Irrational number: A number that cannot be expressed as the ratio of two integers
- Repeating decimal: A decimal in which a digit or group of digits repeats indefinitely
This slide show provides additional support for these terms by providing examples of the term:
https://www.media4math.com/library/slideshow/definitions-ratios-proportions-and-rational-numbers
Instruction (45 minutes)
Rational Numbers. Introduce rational numbers as ratios of integers, emphasizing that they can be positive or negative. You can use this slideshow to introduce rational numbers:
https://www.media4math.com/library/slideshow/ratios-and-rational-numbers
Summary:
- Rational numbers are expressed as a/b where a and b are integers and b ≠ 0
- Examples: 3/4, -2/5, 7/-3, -8/-9
- Discuss how the signs of the numerator and denominator affect the overall sign of the rational number
Remind students that rational numbers are based on ratios. Define proportional relationships and demonstrate how to identify them in tables, graphs, and equations, including negative proportions.
A number that consists of a repeating decimal can be written as a rational number:
| Write an equation: | x = 0.777... |
| Multiply by 10: | 10x = 7.777... |
| Subtract the original equation: | 10x - x = 7.777... - 0.777... |
| Simplify: | 9x = 7 |
| Solve for x: | x = 7/9 |
For a decimal with a repeating pattern of two digits, multiply by 100 and go through the same process.
Proportional Relationships. Use the following examples of ratios and proportions to see rational numbers in context.
Example 1: Real World Connection - Water Pressure vs Depth
As a diver descends underwater, the water pressure increases proportionally to the depth. The pressure increases by 1 atmosphere for every 10 meters of depth.
This History channel video provides additional context:
This table summarizes the change in pressure versus change in depth. Note the negative numbers in column 1.
| Depth (m) | Pressure change (atm) |
|---|---|
| 0 | 0 |
| -10 | 1 |
| -20 | 2 |
| -30 | 3 |
Graph: Plot points (0,0), (-10,1), (-20,2), (-30,3) on a coordinate plane.
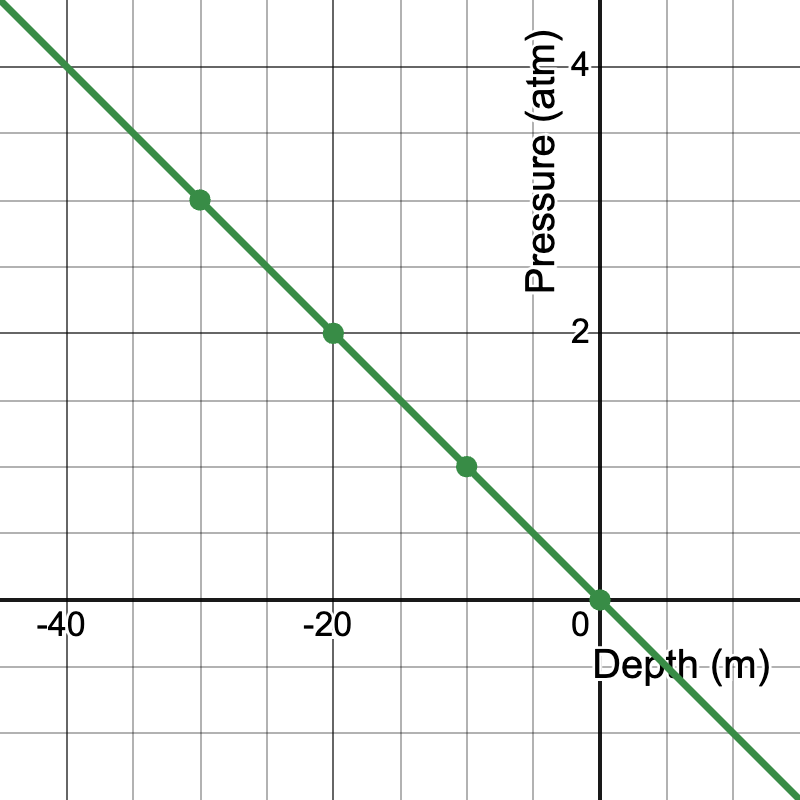
Equation: y = -0.1x, where x is the depth in meters and y is the pressure change in atmospheres.
Solution: This is a proportional relationship because the ratio of y to x is constant (-0.1:1) and the graph passes through the origin. The negative constant of proportionality indicates that as depth decreases negatively, pressure increases positively.
Use this Desmos activity to explore this activity:
https://www.desmos.com/calculator/jg3dnokjjx
Have students use the slider to confirm the constant of proportionality and the equation of the line shown.
Example 2: Real World Connection - Currency Exchange
Use this slide show to walk students through an application of proportional reasoning in the context of currency exchange rates:
https://www.media4math.com/library/slideshow/applications-proportions-exchange-rates
Summary:
- The exchange rate between US dollars and euros is 0.85 Euros per dollar. Write this as an equation and find the constant of proportionality
- Find the dollars-to-Euros exchange rate. Find the corresponding equation.
- An item sells for 45 Euros. Find the price in dollars.
- You want to exchange $500 into Euros. How many Euros will you get?
Solution:
- Unit rate = 0.85 euros per dollar.
- y = 0.85 x. The constant of proportionality is 0.85, representing the exchange rate.
- Dollars-to-Euros: y = 1.18x. The constant of proportionality is 1.18.
- Exchanges:
- 45 Euros = $53.10
- $500 = 425 Euros
Example 3: Real World Connection - Freezer Temperature Drop
The temperature in a freezer is dropping at a rate of -3°C every 5 minutes.
| Time (min) | Temperature change (°C) |
|---|---|
| 0 | 0 |
| 5 | -3 |
| 10 | -6 |
| 15 | -9 |
Equation: y = -0.6x, where x is the time in minutes and y is the temperature change in °C.
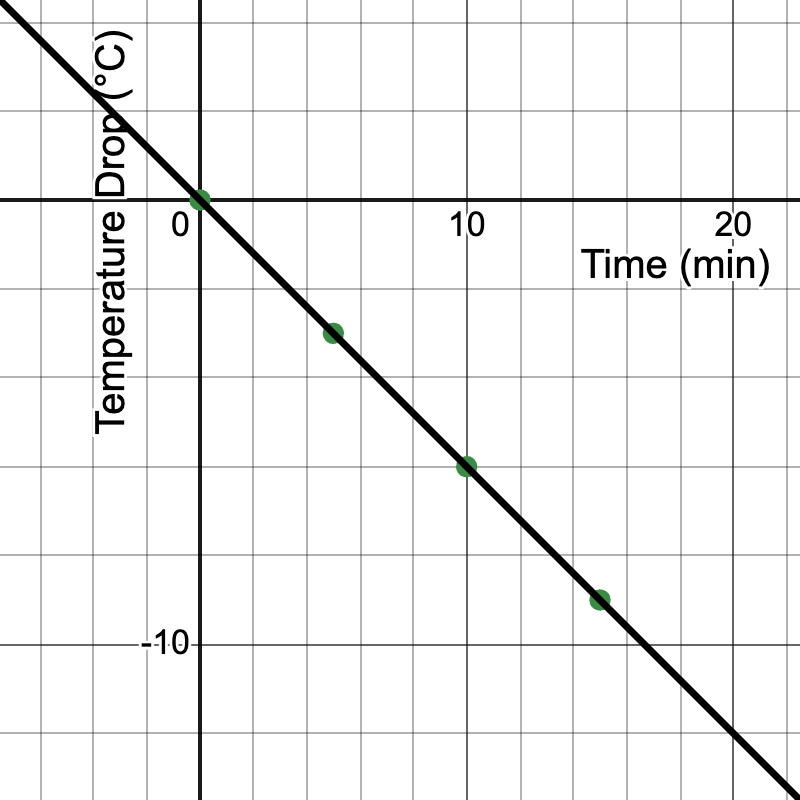
Solution: This is a proportional relationship with a negative constant of proportionality (-0.6). The graph is a straight line passing through the origin, with a negative slope indicating the temperature decrease over time.
Use this Desmos activity, if time allows:
Review (15 minutes)
Group activity: Students create posters showing proportional relationships in different representations and examples of rational numbers expressed as ratios.
Example 1: Positive Constant of Proportionality - Cycling and Grade
Introduce this video, which is an application of proportional reasing in the context of cycling. Grade is the slope of a cycling trail expressed as a percent.
https://www.media4math.com/library/1814/asset-preview
What is the slope of a hill with a grade of 12%? Write the equation that represents this.
Solution:
- The slope is 0.12.
- y = 0.12x
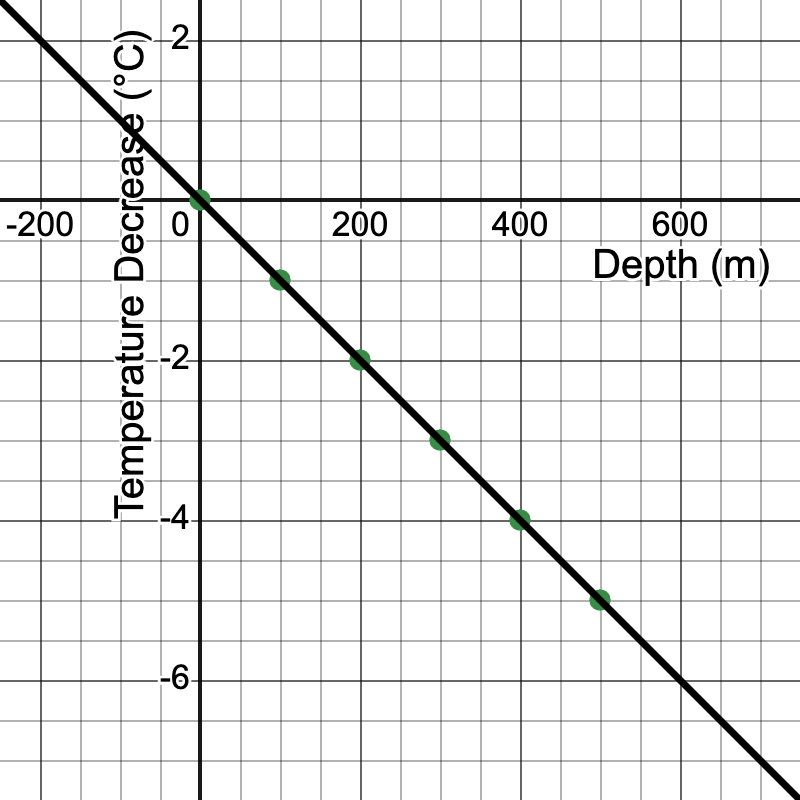
Example 2: Negative Constant of Proportionality - Underwater Temperature Change
As a submarine descends, the water temperature decreases by 1°C for every 100 meters of depth. Create a table and graph showing the temperature change as the depth increases.
| Depth (m) | Temperature Decrease (°C) |
| 0 | 0 |
| 100 | -1 |
| 200 | -2 |
| 300 | -3 |
| 400 | -4 |
| 500 | -5 |
You can also use this Desmos activity:
Assess (10 minutes)
Administer this 12-question quiz.
Quiz
- Is the relationship between x and y proportional? x: 2, 4, 6; y: 6, 12, 18
- What is the constant of proportionality in the equation y = 3x?
- If a car travels 240 miles in 4 hours at a constant speed, what is the unit rate?
- Does the graph of y = 2x + 1 represent a proportional relationship? Why or why not?
- In a recipe, 2 cups of flour are used for every 3 cups of milk. What is the ratio of flour to milk?
- What is the unit rate if 15 items cost $45?
- Convert the repeating decimal 0.363636... to a fraction.
- Express 5/8 as a ratio in proportion to 1.
- Convert 0.25 to a fraction in its simplest form.
- Is 0.333333... a rational or irrational number? Explain using ratio terminology.
- What type of decimal expansion do all rational numbers have?
- Convert 2.7777... to a fraction and express it as a ratio.
Answer Key
- Yes
- 3
- 60 miles per hour
- No, because it doesn't pass through (0,0)
- 2:3
- $3 per item
- 4/11
- 0.625:1 or 5:8
- 1/4
- Rational, because it can be expressed as the ratio 1:3.
- Terminating or repeating.
- 25/9 or 25:9
![]() Purchase the lesson plan bundle. Click here.
Purchase the lesson plan bundle. Click here.