
Illustrative Math Alignment: Grade 6 Unit 3
Unit Rates and Percentages
Lesson 9: Solving Rate Problems
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Nodes |
|---|---|---|---|
| Halloween Math Activities | Description
Turn the Halloween season into an opportunity to do some math activities! In this module, you'll find a clever collection of arithmetic, algebra, and geometry activities. Geometry activity: Watch a video about how spiders build webs and analyze the result geometrically. Students build polygon-based web designs in this hands-on activity. Algebra activity: Going to the pumpkin patch? Use different sized pumpkins on this data-gathering activity, where students measure diameters and circumferences and graph the results. Arithmetic activity: Two addictive divisibility games based on whack-a-mole. |
Applications of Polygons and Applications of Ratios, Proportions, and Percents | |
| Applications of Linear Functions: Temperature Conversion | Description
Temperature is one of the most important measurements that we deal with on a daily basis. Weather, climate, food preparation, health, and other phenomena involve some type of temperature measure. The two most common units of temperature measure are Fahrenheit and Celsius. There is a linear function that allows you to convert from one unit to another. In this module, you'll learn about this linear function. In fact, students will learn about this function and its inverse. The module starts with an analysis of Celsius-to-Fahrenheit data. They look at the functional relationship between the variables and develop a linear model using the Desmos graphing calculator. They analyze the properties of this linear function and look at its graph. Next, students analyze Fahrenheit-to-Celsius data. They also develop a linear function model using the Desmos graphing calculator. |
Applications of Linear Functions and Applications of Ratios, Proportions, and Percents | |
| Applications of Linear Functions: Circumference vs. Diameter | Description
As the size of a circle changes, so does the size of the diameter and that of the circumference. In fact, there is a linear relationship between these two measures. This relationship can be modeled with a linear function. In this module students will study this linear function and examine its properties, including the fact that the slope of this function is π itself. This is a hands-on module in which students will measure the diameters and circumferences of a number of different containers. This data gathering will lead to graphing the data. From that students develop a linear model using the Desmos graphing tool. Students will see that the relationship between circumference and diameter has to do with π. In fact, the slope of the linear function is π itself. |
Applications of Ratios, Proportions, and Percents and Applications of Circles | |

|
Lesson Plan Collection: SAT Math | SAT Math Prep Course | 35-Lesson Unit Prepare for the SAT Math Test with this comprehensive and growing collection of expertly designed SAT Math lesson plans from Media4Math. Whether you're a teacher, tutor, or student, these lessons offer clear, structured, and standards-aligned instruction across the full range of SAT Math topics. |
Slope-Intercept Form, Solving Two-Step Equations, Inequalities, Solving Systems of Equations, Applications of Linear Functions, Special Functions, Applications of Linear Systems, Applications of Ratios, Proportions, and Percents, Percents, Ratios and Rates, Variable Expressions, Data Analysis, Probability, Quadratic Equations and Functions, Quadratic Formula, Exponential and Logarithmic Functions and Equations, Rational Functions and Equations, Relations and Functions, Functions and Their Inverses, Polynomial Functions and Equations, Applications of Quadratic Functions, Applications of Exponential and Logarithmic Functions, Parallel Lines, Applications of Triangles, Applications of Circles, Geometric Constructions with Circles, Applications of Quadrilaterals, Definition of a Quadrilateral, Applications of Coordinate Geometry, The Distance Formula, Applications of Transformations, Trig Expressions and Identities, Trigonometric Functions and Complex Numbers |

|
Lesson Plan Collection: Ratios, Proportions, and Percents (Gr 8) | Ratios, Proportions, and Percents (Gr. 8) | 5-Lesson Unit This comprehensive collection of Grade 8 math lessons explores essential concepts in ratios, proportions, and linear relationships. Through engaging activities and real-world applications, students will develop a strong foundation in proportional reasoning, graphing, and algebraic problem-solving. Lessons in this collection include: |
Ratios and Rates, Percents, Proportions, Applications of Ratios, Proportions, and Percents, Applications of Linear Functions and Graphs of Linear Functions |

|
Lesson Plan Collection: Ratios, Proportions, and Percents (Gr 7) | Ratios, Proportions, and Percents (Gr. 7) | 5-Lesson Unit This comprehensive collection of Grade 7 math lessons focuses on developing students’ understanding of ratios, proportions, and percents through real-world applications and multi-step problem-solving strategies. Each lesson is designed to build critical thinking skills and reinforce proportional reasoning, preparing students for more advanced mathematical concepts. Topics in this collection include: |
Ratios and Rates, Applications of Ratios, Proportions, and Percents, Proportions and Applications of Linear Functions |

|
Lesson Plan Collection: Ratios, Proportions, and Percents (Gr 6) | Ratios, Proportions, and Percents (Gr. 6) | 5-Lesson Unit This comprehensive five-lesson unit is designed to provide sixth-grade students with a solid foundation in understanding and applying ratios, proportions, and percents. Each lesson builds upon the previous, ensuring a cohesive and thorough exploration of these fundamental mathematical concepts. |
Ratios and Rates, Applications of Ratios, Proportions, and Percents and Percents |

|
Math in the News Collection: Applications of Ratios |
OverviewThis is a collection of Math in the News stories that focus on the topic of Ratios, Proportions, and Percents.
|
Applications of Ratios, Proportions, and Percents and Proportions |

|
Math Definitions Collection: Ratios, Proportions, and Percents | OverviewThis collection of definitions on the topic of Ratios, Proportions, and Percents from Media4Math is an invaluable educational resource designed to enhance students' understanding of these fundamental mathematical concepts. This comprehensive collection includes essential terms such as ratio, proportion, percent, rate, unit rate, and scale model. |
Applications of Ratios, Proportions, and Percents, Ratios and Rates, Percents and Proportions |

|
Math Video Collection: Video Tutorials Series: Ratios |
OverviewThis collection aggregates all the math videos and resources in this series: Video Tutorials Series: Ratios. There are a total of 67 resources. This collection of resources is made up of downloadable MP4, transcripts, and other resources files that you can easily incorporate into a presentation.
|
Ratios and Rates, Applications of Ratios, Proportions, and Percents and Proportions |

|
Math Clip Art Collection: 3D Objects |
OverviewThis collection aggregates all the math clip art around the topic of 3D Objects. There are a total of 26 images. This collection of resources is made up of downloadable PNG files that you can easily incorporate into a presentation.To download the full set of these resources, click on this link.
|
3-Dimensional Figures, Applications of 3D Geometry, Applications of Polygons, Applications of Ratios, Proportions, and Percents and Proportions |
| Construction Site Math: Ratios | Description
In this real-world application of ratios, students will learn what a ratio is, including ratios with three terms. They will see different ways of writing ratios, including fractions in simplest form. a:b ab a to b Two short videos introduce the concept of ratios and each video includes real-world examples of ratios. Then several formative assessments are used to test for understanding. Students then look at the application of ratios to the mixing of concrete at a construction site, starting with a video then followed by an assessment. This lesson can be assigned to individual students or teams of students. The lesson can be completed in about 20 minutes. |
Applications of Ratios, Proportions, and Percents | |
| Why Do Rivers Meander? | Description
Rivers twist and turn as they make their way to the sea. Why does this happen and how can this be explained mathematically? In this module we look at the geometry of meandering rivers. In the process we'll discover some fascinating numerical relationships. Students will learn some basic concepts about the geophysics of how rivers form, but they will focus their analysis on what is called the sinuousity ratio. This is the ratio of the straight-line distance of the river and its meandering distance. This ratio reveals important characteristics of the river. Then, students will develop and examine geometric models involving circular arcs and segments to get a better understanding of the ratio. These models will first involve using a compass and straight edge to model a river. This is followed by an algebraic analysis. |
Applications of Ratios, Proportions, and Percents | |
| Why Do Elephants Have Wrinkled Skin? | Description
Have you noticed how wrinkled an elephant's skin is? What purpose does it serve and what does math have to do with explaining this phenomenon? Well, the explanation for an elephant's wrinkled skin is almost entirely a math story. In this module students explore rational expressions and functions in the context of the ratio of surface area and volume for various three-dimensional figures. Such figures can be used to model the basic shapes of animals. This ratio reveals a lot about how an animal is able to retain heat or lose it rapidly, depending on the animal's habitat. The geometry of heat transfer also has applications in architecture and design. What your students will learn: |
Surface Area and Applications of Ratios, Proportions, and Percents | |

|
Closed Captioned Video: Algebra Applications: Inequalities, 1 | Closed Captioned Video: Algebra Applications: Inequalities, 2TopicInequalities DescriptionThis video models an inequality to explain how floodgates in Venice prevent flooding during storms. The key concept is the activation threshold, where the water level exceeds a critical value. Vocabulary includes inequality, threshold, variable, and model. Using a TI-Nspire, the video demonstrates creating a random variable for water levels and a function to evaluate if floodgates should activate. It illustrates real-world applications in engineering and environmental management. |
Applications of Equations and Inequalities and Inequalities |

|
Closed Captioned Video: Algebra Applications: Inequalities, 1 | Closed Captioned Video: Algebra Applications: Inequalities, 2TopicInequalities DescriptionThis video models an inequality to explain how floodgates in Venice prevent flooding during storms. The key concept is the activation threshold, where the water level exceeds a critical value. Vocabulary includes inequality, threshold, variable, and model. Using a TI-Nspire, the video demonstrates creating a random variable for water levels and a function to evaluate if floodgates should activate. It illustrates real-world applications in engineering and environmental management. |
Applications of Equations and Inequalities and Inequalities |

|
Closed Captioned Video: Algebra Applications: Variables and Equations, 3 | Closed Captioned Video: Algebra Applications: Variables and Equations, 3TopicEquations DescriptionThis segment explores the impact of colony collapse disorder on honey production using statistical data. It introduces box and whisker plots and the calculation of mean as statistical tools to analyze honey yields. Key vocabulary includes colony collapse disorder, box plot, and mean. Applications include modeling bee population declines and their broader ecological and agricultural implications. |
Applications of Equations and Inequalities, Variables and Unknowns, Variable Expressions and Applications of Ratios, Proportions, and Percents |

|
Closed Captioned Video: Counting Strategies | Closed Captioned Video: Counting Strategies
In this video tutorial, students are shown how to use Skip Counting and Counting On to quickly and accurately count large numbers of items. This video lays the groundwork for developing addition skills. |
Counting |

|
Closed Captioned Video: Ratios and Rates: Converting Measurement Units | Closed Captioned Video: Ratios and Rates: Converting Measurement UnitsTopicRatios DescriptionThis video covers converting units using rates, with examples like speed conversion, currency exchange, and calculating seconds in a year. It emphasizes multiplication by conversion rates to transition between units effectively. |
Ratios and Rates |

|
Closed Captioned Video: Ratios and Rates: Rates and Slopes of Lines | Closed Captioned Video: Ratios and Rates: Rates and Slopes of LinesTopicRatios DescriptionRates are linked to slopes in linear functions. The video explores calculating rates of change for graphs of speed, savings growth, and loan repayment. It highlights using the slope formula to interpret and solve practical problems. |
Ratios and Rates |

|
Closed Captioned Video: Ratios and Rates: Rates from Data | Closed Captioned Video: Ratios and Rates: Rates from DataTopicRatios DescriptionThis video demonstrates calculating rates from data sets, focusing on patterns like distance-time relationships and wages. Examples include determining car speeds, hourly wages, and unit costs of gasoline. Data tables are used to visualize and compute rates. |
Ratios and Rates |

|
Closed Captioned Video: Ratios and Rates: Ratios as Decimals | Closed Captioned Video: Ratios and Rates: Ratios as DecimalsTopicRatios DescriptionThe video explores working with ratios involving decimals, such as finding unit costs for salmon or recycling rates. Scientific notation and multi-step conversions are used to calculate speeds of spacecraft like Voyager I. |
Ratios and Rates |

|
Closed Captioned Video: Ratios, Proportions, and Percents: Calculating Percents | Closed Captioned Video: Ratios, Proportions, and Percents: Calculating PercentsTopicRatios DescriptionRatios are connected to percentages in this video. Examples include finding percentages of colored socks or non-green items in collections. It develops a formula for converting part-to-whole ratios into percentages. |
Ratios and Rates |

|
Closed Captioned Video: Ratios: Application of Ratios: Roofs and Ramps | Closed Captioned Video: Ratios: Application of Ratios: Roofs and RampsTopicRatios DescriptionThe video covers practical applications of ratios for measuring slopes of roofs and ramps. Examples include comparing roof pitches, calculating base lengths of roofs, and determining ramp heights. Ratios provide clarity for gradual slopes. |
Ratios and Rates and Applications of Ratios, Proportions, and Percents |

|
Closed Captioned Video: Ratios: Rates | Closed Captioned Video: Ratios: RatesTopicRatios DescriptionThis video introduces rates, a type of ratio comparing different units, such as distance over time (speed) or dollars per hour (wage). Examples demonstrate calculating rates and converting units. Applications include measuring efficiency and performing unit conversions. |
Ratios and Rates |

|
Closed Captioned Video: Ratios: Unit Rates | Closed Captioned Video: Ratios: Unit RatesTopicRatios DescriptionThe video focuses on unit rates, where the denominator equals one. It includes practical examples like finding the cost per pound of bananas or determining hourly wages. Applications extend to conversions and scaling calculations for various real-world tasks. |
Ratios and Rates |

|
Closed Captioned Video: Ratios: Visual Models for Ratios and Percents | Closed Captioned Video: Ratios: Visual Models for Ratios and PercentsTopicRatios DescriptionThis video illustrates converting ratios to percents using visual aids like area models and grids. Examples include determining percentages of colored eggs, fruit types, and combinations of colored lights. |
Ratios and Rates |
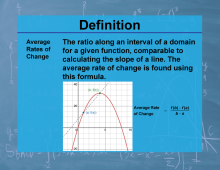
|
Definition--Calculus Topics--Average Rates of Change | Definition--Calculus Topics--Average Rates of ChangeTopicCalculus DefinitionThe average rate of change of a function over an interval is the slope of the secant line connecting the endpoints of that interval on the function's graph. DescriptionThe average rate of change is a fundamental concept in calculus that bridges the gap between algebra and calculus. It provides insight into how a function's output changes relative to its input over a specific interval. This concept is crucial in various real-world applications, such as calculating average speed, growth rates, or productivity changes. |
Calculus Vocabulary |
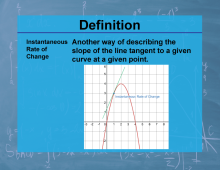
|
Definition--Calculus Topics--Instantaneous Rate of Change | Definition--Calculus Topics--Instantaneous Rate of ChangeTopicCalculus DefinitionThe instantaneous rate of change of a function at a point is the limit of the average rate of change as the interval over which it's calculated approaches zero. It is equivalent to the derivative of the function at that point. |
Calculus Vocabulary |

|
Definition--Financial Literacy--Exchange Rate | Exchange RateTopicFinancial Literacy DefinitionAn exchange rate is the rate at which one currency can be exchanged for another, influencing international trade and investment. |
Numerical and Algebraic Expressions |
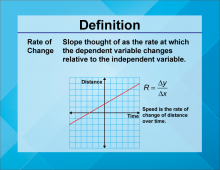
|
Definition--Linear Function Concepts--Rate of Change | Rate of Change
TopicLinear Functions DefinitionRate of change in a linear function is the ratio of the change in the dependent variable to the change in the independent variable, often represented as the slope m in the equation y = mx + b. DescriptionRate of change is a fundamental concept in understanding linear functions. It describes how one variable changes in relation to another, and is graphically represented by the slope of a line. |
Slope |

|
Definition--Ratios, Proportions, and Percents Concepts--Calculating Tax | Calculating TaxTopicRatios, Proportions, and Percents DefinitionCalculating tax involves determining the percentage amount to be added to the base price of a product or service. DescriptionCalculating tax is a fundamental application of percentages in real-world scenarios. When purchasing goods or services, the total cost is often the sum of the base price and the tax applied. Understanding how to calculate tax is essential for budgeting and financial literacy. For example, if a product costs $50 and the tax rate is 8%, the tax amount is calculated as 50 × 0.08 = 4 Therefore, the total cost is |
Applications of Ratios, Proportions, and Percents |

|
Definition--Ratios, Proportions, and Percents Concepts--Calculating Tips | Calculating TipsTopicRatios, Proportions, and Percents DefinitionCalculating tips involves determining the amount of money to give as a gratuity based on a percentage of the total bill. DescriptionCalculating tips is a common use of percentages in everyday life, particularly in service industries such as dining. Tips are usually calculated as a percentage of the total bill, and understanding how to compute this is important for both customers and service providers. For instance, if a meal costs $80 and you want to leave a 15% tip, the tip amount is calculated as 80 × 0.15 = 12 |
Applications of Ratios, Proportions, and Percents |
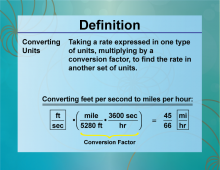
|
Definition--Ratios, Proportions, and Percents Concepts--Converting Units | Converting UnitsTopicRatios, Proportions, and Percents DefinitionConverting units involves changing a measurement from one unit to another using a conversion factor. DescriptionConverting units is essential in various fields such as science, engineering, and everyday life. It involves using ratios and proportions to switch between different measurement systems, such as converting inches to centimeters or gallons to liters. For example, to convert 5 miles to kilometers, knowing that 1 mile is approximately 1.60934 kilometers, you multiply 5 × 1.60934 = 8.0467 kilometers |
Ratios and Rates |

|
Definition--Ratios, Proportions, and Percents Concepts--Dimensional Analysis | Dimensional AnalysisTopicRatios, Proportions, and Percents DefinitionDimensional analysis is a method used to convert one unit of measurement to another using conversion factors. DescriptionDimensional analysis is a powerful tool in mathematics and science for converting units and solving problems involving measurements. It uses the principle of multiplying by conversion factors to ensure that units cancel out appropriately, leading to the desired unit. For example, to convert 50 meters per second to kilometers per hour, you use the conversion factors 1 meter = 0.001 kilometers and 1 hour = 3600 seconds: |
Applications of Ratios, Proportions, and Percents |

|
Definition--Ratios, Proportions, and Percents Concepts--Equivalent Ratios | Equivalent RatiosTopicRatios, Proportions, and Percents DefinitionEquivalent ratios are ratios that express the same relationship between quantities. DescriptionEquivalent ratios are fundamental in understanding proportions and scaling in mathematics. They represent the same relationship between quantities, even though the numbers themselves may differ. This concept is crucial in various applications, such as cooking, map reading, and creating models. For instance, the ratios 2:3 and 4:6 are equivalent because they both simplify to the same ratio when reduced. |
Ratios and Rates |

|
Definition--Ratios, Proportions, and Percents Concepts--Part-to-Part Ratios | Part-to-Part RatiosTopicRatios, Proportions, and Percents DefinitionPart-to-part ratios compare different parts of a whole to each other. DescriptionPart-to-part ratios are used to compare different parts of a whole, providing a way to understand the relationship between different components. This type of ratio is essential in fields such as statistics, biology, and economics. For example, if a class has 10 boys and 15 girls, the part-to-part ratio of boys to girls is 10:15, which simplifies to 2:3. |
Ratios and Rates |

|
Definition--Ratios, Proportions, and Percents Concepts--Part-to-Whole Ratios | Part-to-Whole RatiosTopicRatios, Proportions, and Percents DefinitionPart-to-whole ratios compare one part of a whole to the entire whole. These ratios are more commonly known as fractions. DescriptionPart-to-whole ratios are used to compare a part of a whole to the entire whole, providing insights into the composition of a dataset or population. This type of ratio, more commonly referred to as fractions, is widely used in statistics, finance, and everyday decision-making. |
Ratios and Rates |

|
Definition--Ratios, Proportions, and Percents Concepts--Percent | PercentTopicRatios, Proportions, and Percents DefinitionA percent is a ratio that compares a number to 100. DescriptionPercentages are a fundamental concept in mathematics, representing a ratio out of 100. They are used in various applications, including finance, statistics, and everyday calculations such as discounts and interest rates. For example, if you score 45 out of 50 on a test, your percentage score is (45/50) × 100 = 90% |
Percents |

|
Definition--Ratios, Proportions, and Percents Concepts--Percent Decrease | Percent DecreaseTopicRatios, Proportions, and Percents DefinitionPercent decrease measures the reduction in value expressed as a percentage of the original value. DescriptionPercent decrease is used to quantify the reduction in value over time, expressed as a percentage of the original value. It is commonly used in finance, economics, and everyday scenarios such as price reductions and weight loss. For example, if the price of a jacket drops from $80 to $60, the percent decrease is calculated as (80 − 60)/80 × 100 = 25%. |
Percents |

|
Definition--Ratios, Proportions, and Percents Concepts--Percent Increase | Percent IncreaseTopicRatios, Proportions, and Percents DefinitionPercent increase measures the growth in value expressed as a percentage of the original value. DescriptionPercent increase is used to quantify the growth in value over time, expressed as a percentage of the original value. It is commonly used in finance, economics, and everyday scenarios such as salary increases and population growth. For example, if the price of a stock rises from \$50 to \$75, the percent increase is calculated as (75 − 50)/50 × 100 = 50% |
Percents |

|
Definition--Ratios, Proportions, and Percents Concepts--Percent of a Number | Percent of a NumberTopicRatios, Proportions, and Percents DefinitionPercent of a number involves calculating the amount represented by a certain percentage of that number. DescriptionUnderstanding percentages is crucial for working with finances, statistics, and data analysis. For instance, to find 20% of 50, multiply 50 by 0.20, resulting in 10. Likewise, it's important for everyday scenarios, such as calculating discounts during shopping. |
Percents |

|
Definition--Ratios, Proportions, and Percents Concepts--Percent of an Unknown | Percent of an UnknownTopicRatios, Proportions, and Percents DefinitionPercent of an unknown refers to solving for an unknown quantity when given a percentage of that quantity. DescriptionKnowing how to find a percentage of an unknown variable is essential for solving equations in algebra. This concept appears in various situations, such as when determining discounts or portions of a total amount. For instance, if 20% of an unknown number equals 15, you can set up the equation: 0.20x = 15 |
Percents |

|
Definition--Ratios, Proportions, and Percents Concepts--Percents as Decimals | Percents as DecimalsTopicRatios, Proportions, and Percents DefinitionPercents as decimals involve converting a percentage into its decimal representation. DescriptionConverting percents to decimals is a key skill in mathematics, allowing students to perform calculations involving percentages more easily. To convert, divide the percent by 100. For example, 75% as a decimal is 0.75, calculated by dividing 75 by 100. This conversion is useful in many contexts, such as finance, where calculations are conducted using decimal values. Mastering this concept enables students to approach real-world problems with greater confidence and accuracy. |
Percents |

|
Definition--Ratios, Proportions, and Percents Concepts--Proportion | ProportionTopicRatios, Proportions, and Percents DefinitionA proportion is an equation that states that two ratios are equal. DescriptionUnderstanding proportions is essential in mathematics, as it is used to solve problems involving ratios and fractions. Proportions are commonly seen in real-world applications such as cooking, map measurements, and scale models. To illustrate, if there are 2 apples for every 3 oranges, the proportion can be expressed as 2:3. Solving proportions involves finding and solving an equivalent ratio. |
Proportions |

|
Definition--Ratios, Proportions, and Percents Concepts--Proportional | ProportionalTopicRatios, Proportions, and Percents DefinitionProportional refers to the relationship between two quantities where their ratio is constant. DescriptionProportional relationships are fundamental in mathematics and science, describing how one quantity changes in relation to another. This concept is used in various fields, including physics, economics, and engineering. For example, if the speed of a car is proportional to the time it travels, doubling the time will double the distance covered. Understanding proportionality helps students solve complex problems and apply mathematical reasoning in real-world situations. |
Proportions |

|
Definition--Ratios, Proportions, and Percents Concepts--Rate | RateTopicRatios, Proportions, and Percents DefinitionA rate is a ratio that compares two quantities with different units. DescriptionRates are used to compare different quantities, such as speed (miles per hour) or price (cost per item). Understanding rates is essential for interpreting data and making informed decisions in various contexts, such as travel and budgeting. For instance, if a car travels 60 miles in 2 hours, the rate is 30 miles per hour. Learning about rates helps students analyze real-world situations and apply mathematical reasoning to everyday problems. |
Ratios and Rates |

|
Definition--Ratios, Proportions, and Percents Concepts--Ratio | RatioTopicRatios, Proportions, and Percents DefinitionA ratio is a comparison of two quantities by division. DescriptionRatios are used to express the relationship between two quantities, providing a way to compare different amounts. They are fundamental in various fields, including mathematics, science, and finance. For example, the ratio of 4 to 5 can be written as 4:5 or 4/5. Understanding ratios helps students analyze data, solve problems, and make informed decisions in real-world situations. |
Ratios and Rates |

|
Definition--Ratios, Proportions, and Percents Concepts--Ratios and Fractions | Ratios and FractionsTopicRatios, Proportions, and Percents DefinitionRatios and fractions are both ways of comparing quantities, with fractions representing a part of a whole. DescriptionUnderstanding the connection between ratios and fractions is crucial for solving problems involving proportions and scaling. Ratios can be expressed as fractions, providing a way to understand the relationship between quantities. A fraction is a part-whole ratio. |
Ratios and Rates |
|
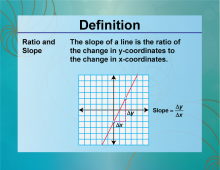
Definition--Ratios, Proportions, and Percents Concepts--Ratios and Slope | Ratios and SlopeTopicRatios, Proportions, and Percents DefinitionThe slope of a line is a ratio that represents the change in y over the change in x. DescriptionUnderstanding the relationship between ratios and slope is essential for interpreting graphs and solving problems in algebra and geometry. The slope is a measure of how steep a line is, calculated as the ratio of the vertical change to the horizontal change between two points. For example, if a line rises 2 units for every 3 units it runs horizontally, the slope is 2/3. This concept is crucial for understanding linear relationships and analyzing data in various fields. |
Ratios and Rates |