
Illustrative Math Alignment: Grade 7 Unit 7
Expressions, Equations, and Inequalities
Lesson 10: Different Options for Solving One Equation
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|

|
Video Definition 15--Equation Concepts--Identity Equation | Video Definition 15--Equation Concepts--Identity Equation
TopicEquations DescriptionAn Identity Equation is always true for all variable values. Examples include x = x and (x + 1)2 = x2 + 2x + 1. Identity equations often use the symbol ≡ to indicate their universal truth. This term introduces a special class of equations, linking algebraic manipulation and properties of equivalence. |
Applications of Equations and Inequalities |

|
Video Definition 15--Linear Function Concepts--Linear Equations in Standard Form | Video Definition 15--Linear Function Concepts--Linear Equations in Standard Form
TopicLinear Functions DescriptionThe term is "Linear Equation in Standard Form," defined as a linear equation with two variables and three constant terms, represented as Ax + By = C where A and B are not equal to zero. This term sets the stage for converting between forms of linear equations, essential for solving and graphing. |
Standard Form |

|
Video Definition 16--Equation Concepts--Inequation | Video Definition 16--Equation Concepts--Inequation
TopicEquations DescriptionAn Inequation is a mathematical statement that shows two expressions are not equal, as in 2 + 1 ≠ 4 or x + 3 ≥ 0, which includes inequalities. This term broadens the discussion to include inequalities, expanding the types of relationships between expressions. |
Inequalities |

|
Video Definition 17--Equation Concepts--Isolating the Variable | Video Definition 17--Equation Concepts--Isolating the Variable
TopicEquations DescriptionIsolating the Variable refers to the process of solving an equation by focusing on and isolating the variable of interest. For example, x + 3 = 5 becomes x = 2 by subtracting 3 from both sides. This term highlights a key step in solving equations, emphasizing strategies for simplifying equations to find solutions. |
Variables and Unknowns |

|
Video Definition 18--Equation Concepts--Left Side of the Equation | Video Definition 18--Equation Concepts--Left Side of the Equation
TopicEquations DescriptionThe Left Side of the Equation is the expression on the left of the equals sign. For example, in 2x + 3 = x - 5, the left side is 2x + 3. This term provides clarity in identifying components of equations, aiding in understanding structure and solving strategies. |
Applications of Equations and Inequalities |

|
Video Definition 19--Equation Concepts--Linear Equation | Video Definition 19--Equation Concepts--Linear Equation
TopicEquations DescriptionA Linear Equation is an equation where variables have an exponent of 1. Examples include x + 2 = 7, 2x + 3y = 4, and y = -2x + 10. Graphs of two-variable linear equations are straight lines. This term introduces a fundamental class of equations with specific properties, crucial for algebra and graphing. |
Applications of Linear Functions |

|
Video Definition 2--Equation Concepts--Addition Property of Equality | Video Definition 2--Equation Concepts--Addition Property of Equality
TopicEquations DescriptionThe Addition Property of Equality allows the addition of the same quantity to each side of an equation while maintaining equality. For example, if a = b, then a + c = b + c. This concept ensures equations can be manipulated while preserving their equality, demonstrated by solving x - 2 = 3 as x = 5 after adding 2 to both sides. This term introduces the basic manipulation of equations, forming the foundation for solving linear equations and maintaining equality. |
Applications of Equations and Inequalities |

|
Video Definition 20--Equation Concepts--Literal Equation | Video Definition 20--Equation Concepts--Literal Equation
TopicEquations DescriptionA Literal Equation includes more than one variable and often represents formulas, such as A = πr2, V = l*w*h, and SA = 2πr*h + 2πr2. This term connects to real-world applications and highlights the versatility of equations in representing formulas and relationships. |
Applications of Equations and Inequalities |

|
Video Definition 20--Linear Function Concepts--System of Linear Equations | Video Definition 20--Linear Function Concepts--System of Linear Equations
TopicLinear Functions DescriptionThe term is "System of Linear Equations," defined as a collection of linear equations where the number of equations matches the number of variables, allowing for an algebraic solution. Examples include 2-variable and 3-variable systems. This term introduces the concept of interacting linear equations, paving the way for multi-variable problem-solving. |
Applications of Linear Functions and Graphs of Linear Functions |

|
Video Definition 21--Equation Concepts--Multi-Step Equation | Video Definition 21--Equation Concepts--Multi-Step Equation
TopicEquations DescriptionA Multi-Step Equation involves more than two mathematical operations to solve. For example, 4x + 2 = 2x + 8 simplifies to x = 3 after subtraction and division steps. This term emphasizes solving more complex equations, showing sequential steps and reinforcing logical progression in algebra. |
Solving Multistep Equations |

|
Video Definition 21--Linear Function Concepts--Solution to a Linear Equation | Video Definition 21--Linear Function Concepts--Solution to a Linear Equation
TopicLinear Functions DescriptionThe term is "Solution to a Linear Equation," defined as finding the value(s) of a variable that make the equation true. An example includes solving 2x + 5 = 15 step-by-step to find x = 5. This term explains how linear equations are solved, demonstrating the logical process of isolating variables. |
Solving Systems of Equations |

|
Video Definition 22--Equation Concepts--Multiplication Property of Equality | Video Definition 22--Equation Concepts--Multiplication Property of Equality
TopicEquations DescriptionThe Multiplication Property of Equality allows multiplication by the same non-zero quantity on both sides of an equation, maintaining equality. For example, if a = b, then a * c = b * c. Solving x/2 = 3 yields x = 6 by multiplying both sides by 2. This term builds on equality principles and demonstrates another method for solving equations by manipulating terms. |
Applications of Equations and Inequalities |

|
Video Definition 23--Equation Concepts--Nonlinear Equation | Video Definition 23--Equation Concepts--Nonlinear Equation
TopicEquations DescriptionA Non-Linear Equation includes non-linear terms such as polynomials of degree 2 or higher, exponential, logarithmic, or trigonometric terms. Examples include y = x2 - 4x + 5 and y = sin(x). This term expands understanding beyond linear relationships, introducing diverse mathematical models and their applications. |
Applications of Equations and Inequalities |

|
Video Definition 24--Equation Concepts--Numerical Expression | Video Definition 24--Equation Concepts--Numerical Expression
TopicEquations DescriptionA Numerical Expression consists of numbers and operations without variables. Examples include 2 + 3 = 5 and 2(4 + 3) = 14. This term highlights fundamental operations, foundational for understanding and solving equations. The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Numerical Expressions |

|
Video Definition 25--Equation Concepts--One-Step Equation | Video Definition 25--Equation Concepts--One-Step Equation
TopicEquations DescriptionA One-Step Equation involves a single mathematical operation to solve. For example, x + 2 = 3 simplifies to x = 1 by subtraction. This term introduces the simplest form of equations, essential for beginners to build confidence and understanding in solving. |
Solving One-Step Equations |

|
Video Definition 26--Equation Concepts--Polynomial Equation | Video Definition 26--Equation Concepts--Polynomial Equation
TopicEquations DescriptionA Polynomial Equation consists of a polynomial expression equal to zero. Examples include x2 + 3x + 2 = 0 and x3 - 2x2 + 4x - 10 = 0. This term defines a key class of equations, critical for algebra and calculus studies. |
Polynomial Functions and Equations |

|
Video Definition 27--Equation Concepts--Quadratic Equation | Video Definition 27--Equation Concepts--Quadratic Equation
TopicEquations DescriptionA Quadratic Equation is a polynomial of degree 2. Examples include x2 - 2 = 23 and 2x2 + 3x + 2 = 0. Graphs of such equations are parabolas. This term extends understanding of polynomial equations, connecting algebra to geometry through parabolas. |
Quadratic Equations and Functions |

|
Video Definition 27--Rationals and Radicals--Radical Equations | Video Definition 27--Rationals and Radicals--Radical Equations
TopicRationals and Radicals DescriptionRadical equations involve solving expressions with radicals by raising both sides to a power. An example solves √(x + 2) = x, resulting in x = 2 or x = -1. This term demonstrates how radicals can be manipulated algebraically to find solutions, integrating concepts of nth roots and square roots. |
Radical Functions and Equations |

|
Video Definition 27--Rationals and Radicals--Radical Equations (Spanish Audio) | Video Definition 27--Rationals and Radicals--Radical Equations (Spanish Audio)
TopicRationals and Radicals DescriptionRadical equations involve solving expressions with radicals by raising both sides to a power. An example solves √(x + 2) = x, resulting in x = 2 or x = -1. This term demonstrates how radicals can be manipulated algebraically to find solutions, integrating concepts of nth roots and square roots. This video includes Spanish audio and can be used with multilingual learners. |
Radical Functions and Equations |

|
Video Definition 28--Equation Concepts--Reflexive Property of Equality | Video Definition 28--Equation Concepts--Reflexive Property of Equality
TopicEquations DescriptionThe Reflexive Property of Equality states that any quantity is equal to itself. Examples include 5 = 5 and x + 2 = x + 2. This term reinforces the foundational principle of equality, essential for logical reasoning in mathematics. |
Applications of Equations and Inequalities |

|
Video Definition 29--Equation Concepts--Right Side of the Equation | Video Definition 29--Equation Concepts--Right Side of the Equation
TopicEquations DescriptionRight Side of the Equation. The expression to the right side of the equals sign in an equation. 2x + 3 = x - 5 (right side highlighted) The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 3--Equation Concepts--Algebraic Equation | Video Definition 3--Equation Concepts--Algebraic Equation
TopicEquations DescriptionAn Algebraic Equation is another term for a polynomial equation, represented as a sum of terms equal to zero. Examples include x2 + 3x + 2 = 0 and x3 - 2x2 + 4x - 10 = 0. It highlights the structure of polynomial equations using constants, variables, and powers. This term connects to the topic by defining the types of equations learners encounter when solving or simplifying expressions. |
Applications of Equations and Inequalities |
|
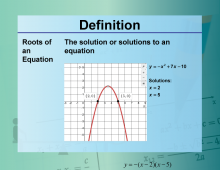
Video Definition 30--Equation Concepts--Roots of an Equation | Video Definition 30--Equation Concepts--Roots of an Equation
TopicEquations DescriptionRoots of an Equation. The solution or solutions to an equation. y = -x2 + 7x - 10; solutions: x = 2, x = 5 The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 31--Equation Concepts--Solution | Video Definition 31--Equation Concepts--Solution
TopicEquations DescriptionSolution. A value for the unknown that turns a conditional equation into a true equation. For example, x = 5 is a solution to x + 2 = 7 The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 32--Equation Concepts--Solving an Equation | Video Definition 32--Equation Concepts--Solving an Equation
TopicEquations DescriptionSolving an Equation. The process of finding the value(s) for a variable that result in a true equation. For example, x + 2 = 3 has a solution of x = 1 The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 33--Equation Concepts--Subtraction Property of Equality | Video Definition 33--Equation Concepts--Subtraction Property of Equality
TopicEquations DescriptionSubtraction Property of Equality. The property that allows the subtraction of the same quantity from each side of an equation while maintaining the equality. For example, x + 2 = 3 becomes x = 1 The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 33--Rationals and Radicals--Rational Equations | Video Definition 33--Rationals and Radicals--Rational Equations
TopicRationals and Radicals DescriptionRational Equations are equations involving fractions where both sides include variables. These often involve steps to solve for variables, like x in (2x + 6) / (x - 3) = 5. Extends the use of fractions by incorporating variables, creating a bridge between fractions and algebra. |
Rational Functions and Equations |

|
Video Definition 33--Rationals and Radicals--Rational Equations (Spanish Audio) | Video Definition 33--Rationals and Radicals--Rational Equations (Spanish Audio)
TopicRationals and Radicals DescriptionRational Equations are equations involving fractions where both sides include variables. These often involve steps to solve for variables, like x in (2x + 6) / (x - 3) = 5. Extends the use of fractions by incorporating variables, creating a bridge between fractions and algebra. This video includes Spanish audio and can be used with multilingual learners. |
Rational Functions and Equations |

|
Video Definition 34--Equation Concepts--Symmetric Property of Equality | Video Definition 34--Equation Concepts--Symmetric Property of Equality
TopicEquations DescriptionSymmetric Property of Equality. The property that states that an equation can be written two ways, with both sides interchanged. If a = b, then b = a. The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 35--Equation Concepts--The Unknown | Video Definition 35--Equation Concepts--The Unknown
TopicEquations DescriptionThe Unknown. In a conditional equation, the variable is also referred to as the unknown. 3x = 12, where x is the unknown The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Variables and Unknowns |

|
Video Definition 36--Equation Concepts--Transitive Property of Equality | Video Definition 36--Equation Concepts--Transitive Property of Equality
TopicEquations DescriptionTransitive Property of Equality. The property that states that two quantities equal to a third quantity are equal to each other. If a = b and b = c, then a = c The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 37--Equation Concepts--True Equation | Video Definition 37--Equation Concepts--True Equation
TopicEquations DescriptionTrue Equation. A mathematically accurate equation. If variables are involved, the true equation is for the values of the variable that make the equation true. Examples: 2 + 1 = 3; (2 + 3)2 = 25; x + 3 = 9 for x = 6 The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 38--Equation Concepts--Two-Step Equation | Video Definition 38--Equation Concepts--Two-Step Equation
TopicEquations DescriptionTwo-Step Equation. A two-step equation is an equation that involves two mathematical operations to solve. The example given is 2x + 2 = 8, where the first operation is subtraction, leading to 2x = 6, followed by division to solve for x = 3. This concept extends the understanding of solving equations by introducing a sequence of operations, crucial for solving more complex equations. |
Solving Two-Step Equations |

|
Video Definition 39--Equation Concepts--Variable Expression | Video Definition 39--Equation Concepts--Variable Expression
TopicEquations DescriptionVariable Expression. A variable expression is made up of numbers, operations, and variables. Examples include 2x + 3 = 5 (expression on the left), 4 = 3x + 7 (expression on the right), and 4x + 3 = x - 5 (expressions on both sides). This connects variables to equations and highlights their role in forming relationships within mathematical contexts. |
Variable Expressions |

|
Video Definition 4--Equation Concepts--Algebraic Expression | Video Definition 4--Equation Concepts--Algebraic Expression
TopicEquations DescriptionAn Algebraic Expression consists of numbers, operations, and variables. Unlike equations, expressions may not have an equality. Examples include 2x + 3, 4 = 3x + 7, and 4x + 3 = x - 5, demonstrating their versatility in representing mathematical relationships. This term distinguishes between expressions and equations, forming the basis for manipulating algebraic forms before solving equations. |
Numerical and Algebraic Expressions |
|
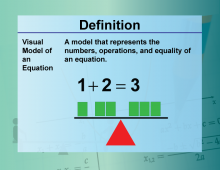
Video Definition 40--Equation Concepts--Visual Models for Equations | Video Definition 40--Equation Concepts--Visual Models for Equations
TopicEquations DescriptionVisual Model of an Equation. A visual model of an equation represents the numbers, operations, and equality using visuals. The example shows 1 + 2 = 3 with blocks illustrating the balance of the equation. This visual approach reinforces the foundational concept of equality and provides an intuitive way to understand equations, especially for visual learners. |
Applications of Equations and Inequalities |

|
Video Definition 41--Equation Concepts--Approximately Equal To | Video Definition 41--Equation Concepts--Approximately Equal To
TopicEquations DescriptionThe Approximately Equal To Sign (≈) indicates that two expressions are close in value but not equal. Examples include estimating sums (58 + 49 ≈ 100), square roots (√50 ≈ 7), and function values (f(x) ≈ 25). This term relates to the topic by introducing estimation techniques, valuable for checking solutions or simplifying problems. |
Applications of Equations and Inequalities |

|
Video Definition 42--Equation Concepts--Proportional To | Video Definition 42--Equation Concepts--Proportional To
TopicEquations DescriptionProportional To indicates a linear relationship between two variables. For example, distance (d) is proportional to time (t), written as d ∝ t. This term links linear equations to real-world relationships, reinforcing practical applications of mathematical concepts. |
Applications of Equations and Inequalities |
|
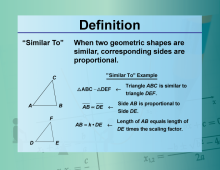
Video Definition 43--Equation Concepts--Similar To | Video Definition 43--Equation Concepts--Similar To
TopicEquations DescriptionSimilar To. When two geometric shapes are similar, corresponding sides are proportional. ΔABC ~ ΔDEF; AB ∝ DE The topic of this video is closely tied to Equations, providing an essential understanding of its fundamental concepts. This video explores the mathematics behind this topic by delving into how key principles are applied in practical contexts. |
Applications of Equations and Inequalities |

|
Video Definition 44--Equation Concepts--Congruent To | Video Definition 44--Equation Concepts--Congruent To
TopicEquations DescriptionCongruent To signifies that two geometric shapes are congruent, meaning their corresponding sides and angles are equal. For example, triangles ABC and DEF are congruent, so side AB equals side DE. This term introduces geometric equality, tying equations to geometric properties and congruence criteria. |
Applications of Equations and Inequalities |

|
Video Definition 5--Equation Concepts--Assigning Values to Variables | Video Definition 5--Equation Concepts--Assigning Values to Variables
TopicEquations DescriptionAssigning Values to Variables involves assigning a numerical value to a variable for evaluating functions. For instance, let x = 3 in f(x) = 2x + 8 yields f(3) = 14. This term supports understanding how to evaluate equations and functions by substituting values, a key step in problem-solving. |
Variable Expressions |

|
Video Definition 6--Equation Concepts--Conditional Equation | Video Definition 6--Equation Concepts--Conditional Equation
TopicEquations DescriptionA Conditional Equation is true only for specific variable values. Examples include x + 1 = 4 (x = 3), x2 - 25 = 0 (x = ±5), and 2x - 5 = x + 9 (x = 14). This term supports solving equations by emphasizing that solutions depend on satisfying the given conditions. |
Applications of Equations and Inequalities |

|
Video Definition 7--Equation Concepts--Constant Term | Video Definition 7--Equation Concepts--Constant Term
TopicEquations DescriptionA Constant Term in an algebraic equation is a number without a variable. Examples include 2 in x2 + 3x + 2 = 0, -10 in x3 - 2x2 + 4x - 10 = 0, and 5 in x4 - 2x3 + 4x2 - x + 5 = 0. This term explains the role of constants in equations, crucial for identifying and isolating terms when solving equations. |
Variables and Unknowns |
|
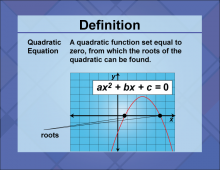
Video Definition 7--Quadratics Concepts--Quadratic Equation | Video Definition 7--Quadratics Concepts--Quadratic Equation
TopicQuadratics DescriptionQuadratic Equation: A quadratic function set equal to zero, from which the roots of the quadratic can be found. This is foundational in solving quadratic problems algebraically. Introduces the mathematical setup for finding solutions to quadratic functions. Relevance to Topic: This video series provides a deep dive into the topic of Quadratics. It explores mathematical concepts and demonstrates real-world applications to help solidify understanding. |
Quadratic Equations and Functions |

|
Video Definition 8--Equation Concepts--Division Property of Equality | Video Definition 8--Equation Concepts--Division Property of Equality
TopicEquations DescriptionThe Division Property of Equality allows division by the same non-zero quantity on both sides of an equation, maintaining equality. For example, if a = b, then a / c = b / c, where c ≠ 0. Solving 5x = 15 yields x = 3 by dividing both sides by 5. This term builds on equality principles, showing another method to isolate variables and solve equations. |
Applications of Equations and Inequalities |

|
Video Definition 9--Equation Concepts--Equality | Video Definition 9--Equation Concepts--Equality
TopicEquations DescriptionEquality refers to a mathematical statement where two expressions are equal. Examples include 1 + 2 = 3 and x + 3 = 7, where both sides represent the same value. This term defines the core concept of equations, ensuring all manipulations preserve equality to find solutions. |
Applications of Equations and Inequalities |

|
Video Definition 9--Linear Function Concepts--The Equation of a Line From Two Coordinates | Video Definition 9--Linear Function Concepts--The Equation of a Line From Two Coordinates
TopicLinear Functions DescriptionThe term is "The Equation of a Line from Two Coordinates," defined as deriving the equation of a line in slope-intercept form from two given points. The slope is calculated as m = (y2 - y1) / (x2 - x1), and the equation is written as y - y1 = m(x - x1). This term builds the connection between points on a graph and their corresponding linear equation, demonstrating practical derivations. |
Point-Slope Form |

|
Video Transcript: Algebra Applications: Systems of Equations | Video Transcript: Algebra Applications: Systems of Equations
This is the transcript for the video of same title. Video contents: In this episode of Algebra Applications, students explore various scenarios that can be explained through the use of systems of equations. Such disparate phenomena as profit and loss, secret codes, and ballistic missile shields can be explored through systems of equations. |
Applications of Linear Systems, Matrix Operations and Solving Systems of Equations |

|
Video Transcript: Algebra Applications: Systems of Equations, Segment 1: Profit and Loss | Video Transcript: Algebra Applications: Systems of Equations, Segment 1: Profit and Loss
This is the transcript for the video of same title. Video contents: Profit and loss are the key measures in a business. A system of equations that includes an equation for income and one for expenses can be used to determine profit and loss. Students solve a system graphically. |
Applications of Linear Systems, Matrix Operations and Solving Systems of Equations |

|
Video Transcript: Algebra Applications: Systems of Equations, Segment 2: Encryption | Video Transcript: Algebra Applications: Systems of Equations, Segment 2: Encryption
This is the transcript for the video of same title. Video contents: Secret codes and encryption are ideal examples of a system of equations. In this activity, students encrypt and decrypt a message. This is part of a collection of video transcript from the Algebra Applications video series. To see the complete collection of transcripts, click on this link. Note: The download is a PDF file. Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Applications of Linear Systems, Matrix Operations and Solving Systems of Equations |