
Illustrative Math Alignment: Grade 8 Unit 1
Rigid Transformations and Congruence
Lesson 10: Composing Figures
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Topic |
|---|---|---|---|

|
Math Clip Art--Geometry Concepts--Composite Figures--Simple Composite Shapes: 07 | Math Clip Art--Composite Figures--Simple Composite Shapes: 07TopicGeometry Concepts DescriptionThis image depicts a composite shape made up of a rectangle and two parallelograms. This configuration is often used in architectural designs and various structural layouts. To calculate the total area of this composite figure, we would add the area of the rectangle (l * w) to the areas of the two parallelograms (bh for each). The perimeter would involve summing the lengths of all outer sides, excluding any shared edges. |
Modeling Shapes |

|
Math Clip Art--Geometry Concepts--Composite Figures--Simple Composite Shapes: 08 | Math Clip Art--Composite Figures--Simple Composite Shapes: 08TopicGeometry Concepts DescriptionThis image illustrates a sawtooth composite shape made up of a rectangle and four congruent equilateral triangles. This configuration is often seen in mechanical designs and architectural elements. To calculate the total area of this composite figure, we would add the area of the rectangle (l * w) to the areas of the four equilateral triangles (A = (√3/4)s² for each). The perimeter would involve summing the lengths of all outer sides, excluding any shared edges. |
Modeling Shapes |

|
Math Clip Art--Geometry Concepts--Composite Figures--Simple Composite Shapes: 09 | Math Clip Art--Composite Figures--Simple Composite Shapes: 09TopicGeometry Concepts DescriptionThis image shows an hourglass composite shape made up of two congruent equilateral triangles, next to two congruent circular sectors. This configuration is often used in artistic designs and educational contexts. This design also has a shape that looks somewhat elliptical. To calculate the total area of this composite figure, we would add the areas of the two equilateral triangles (A = (√3/4)s² for each) to the areas of the two circular sectors (½r²θ for each, where θ is the central angle in radians). The perimeter would involve summing the lengths of all outer sides, excluding any shared edges. |
Modeling Shapes |

|
Math Clip Art--Geometry Concepts--Composite Figures--Simple Composite Shapes: 10 | Math Clip Art--Composite Figures--Simple Composite Shapes: 10TopicGeometry Concepts DescriptionThis image depicts a composite shape made up of a rectangle, a parallelogram, and two congruent right triangles. This configuration is often used in architectural designs and various structural layouts. To calculate the total area of this composite figure, we would add the area of the rectangle (l * w), the area of the parallelogram (bh), and the areas of the two right triangles (½bh for each). The perimeter would involve summing the lengths of all outer sides, excluding any shared edges. |
Modeling Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 01 | Math Clip Art--Roadside Geometry: 01TopicGeometry Concepts DescriptionThis image illustrates the "Do Not Enter" sign, which includes a circle and a square. A circle is a simple closed shape where all points are equidistant from the center. A square is a four-sided figure with congruent sides and four right angles. This sign has curved corners, but you can see the underlying geometry. Circles are used in road signs to convey clear, direct messages that need to be easily recognizable and universally understood. The symmetry and uniformity of a circle make it an effective shape for such signs. The same goes for square |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 02 | Math Clip Art--Roadside Geometry: 02TopicGeometry Concepts DescriptionThis image depicts the "Stop" sign, which is an octagon. An octagon is an eight-sided polygon, and the regular octagon used for stop signs has equal sides and angles, with each interior angle measuring 135 degrees. The octagonal shape is unique and immediately recognizable, which is why it is used for stop signs. This distinct shape helps drivers quickly identify the sign and understand its message, enhancing road safety. Teacher's Script: "Look at the octagonal shape of the 'Stop' sign. Why do you think an octagon is used for stop signs? How does its unique shape help convey the message effectively?" |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 03 | Math Clip Art--Roadside Geometry: 03TopicGeometry Concepts DescriptionThis image illustrates the "School Crossing" sign, which is typically a pentagon. A pentagon is a five-sided polygon, and the irregular pentagon used for school signs does not have equal sides and angles. A regular pentagon has five equal sides and angles, with each interior angle measuring 108 degrees. The pentagonal shape is used to denote school zones and crossings, providing a distinct and recognizable form that alerts drivers to the presence of children and the need for caution. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 04 | Math Clip Art--Roadside Geometry: 04TopicGeometry Concepts DescriptionThis image depicts the "Yield" sign, which is a downward-pointing equilateral triangle. An equilateral triangle has three equal sides and angles, with each interior angle measuring 60 degrees. The triangular shape is used for yield signs to convey caution and alert drivers to give way to oncoming traffic. The distinct shape and orientation make it easily identifiable and effective in communicating the need to yield. Teacher's Script: "Look at the triangular shape of the 'Yield' sign. Why do you think a triangle is used for yield signs? How does its shape and orientation help convey the message effectively?" |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 05 | Math Clip Art--Roadside Geometry: 05TopicGeometry Concepts DescriptionThis image illustrates the "County Road" sign, which is typically an irregular pentagon. A pentagon is a five-sided polygon. Teacher's Script: "Observe the pentagon shape of the 'County Road' sign. Why do you think pentagons are used for these types of signs? How does the shape help convey the message effectively?" For a complete collection of math clip art related to Geometry, click on this link: Math Clip Art: Geometry Concepts Collection. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 06 | Math Clip Art--Roadside Geometry: 06TopicGeometry Concepts DescriptionThis image illustrates the "Railroad Crossing" sign, which is composed of two intersecting rectangles forming an X shape. Each rectangle has opposite sides that are equal and parallel, and all angles are right angles. The intersecting rectangles create a distinct X shape, which is used to denote railroad crossings. This configuration is easily recognizable and alerts drivers to the presence of train tracks, emphasizing caution and awareness. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 07 | Math Clip Art--Roadside Geometry: 07TopicGeometry Concepts DescriptionThis image illustrates the "Bike Lane" sign, which is typically rectangular. A rectangle is a four-sided polygon with opposite sides that are equal and parallel, and four right angles. Rectangular signs are commonly used for informational purposes, providing clear and straightforward messages. The symmetry and simplicity of a rectangle make it a practical choice for road signs that convey directions or lane designations. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 08 | Math Clip Art--Roadside Geometry: 08TopicGeometry Concepts DescriptionThis image depicts the "Road Closed" sign, which is typically rectangular. A rectangle is a four-sided polygon with opposite sides that are equal and parallel, and four right angles. Rectangular signs are commonly used for informational purposes, providing clear and straightforward messages. The symmetry and simplicity of a rectangle make it a practical choice for road signs that convey closures or restrictions. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 09 | Math Clip Art--Roadside Geometry: 09TopicGeometry Concepts DescriptionThis image illustrates the "No Parking" sign, which is primarily rectangular in shape. A rectangle is a four-sided polygon with opposite sides that are equal and parallel, and four right angles. This rectangular shape serves as the main structure of the sign, providing a clear and straightforward format for conveying information. Inside the rectangle, there is a circular symbol, which is often used to emphasize regulatory messages. The circle, a simple closed shape where all points are equidistant from the center, draws attention to the specific prohibition or instruction, such as "No Parking." |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 10 | Math Clip Art--Roadside Geometry: 10TopicGeometry Concepts DescriptionThis image depicts the "No U-Turn" road sign, which is primarily square in shape. A square is a four-sided polygon with equal sides and angles, providing a symmetrical form that is easily recognizable. Inside the square is a circular section that displays the prohibition of making a U-turn, highlighting the specific action being regulated. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 11 | Math Clip Art--Roadside Geometry: 11TopicGeometry Concepts DescriptionThis image illustrates the "Railroad Crossing" sign, which is circular. A circle is a simple closed shape where all points are equidistant from the center. Circular signs are often used for regulatory purposes, drawing attention due to their distinct shape. The interior of the sign shows an X, which highlights the railorad crossing. Another variation of this sign is the X-shaped version. Circles are used in road signs to convey clear, direct messages that need to be easily recognizable and universally understood. The symmetry and uniformity of a circle make it an effective shape for such signs. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 12 | Math Clip Art--Roadside Geometry: 12TopicGeometry Concepts DescriptionThis image depicts the "Road Work" sign, which is typically diamond-shaped. A diamond shape, or rhombus, is a four-sided polygon with equal sides and opposite equal angles. Diamond-shaped signs are used to convey warnings and cautionary messages. The distinct shape draws attention and helps drivers quickly recognize the need for caution due to road work or other temporary conditions. Teacher's Script: "Look at the diamond shape of the 'Road Work' sign. Why do you think diamond shapes are used for warning signs? How does the shape help convey the message effectively?" |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 13 | Math Clip Art--Roadside Geometry: 13TopicGeometry Concepts DescriptionThis image illustrates the "Speed Limit" sign, which is typically rectangular. A rectangle is a four-sided polygon with opposite sides that are equal and parallel, and four right angles. Rectangular signs are commonly used for informational purposes, providing clear and straightforward messages. The symmetry and simplicity of a rectangle make it a practical choice for road signs that convey speed limits or other regulatory information. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 14 | Math Clip Art--Roadside Geometry: 14TopicGeometry Concepts DescriptionThis image depicts the "No Passing Zone" sign, which is typically a pennant-shaped isosceles triangle. This triangle has two equal sides and a distinct pointed shape that indicates caution. The pennant shape is used to signal no passing zones, providing a clear and recognizable warning to drivers. Its unique orientation and shape help convey the message effectively, alerting drivers to maintain their lane. |
Identifying Shapes |

|
Math Clip Art--Geometry Concepts--Roadside Geometry: 15 | Math Clip Art--Roadside Geometry: 15TopicGeometry Concepts DescriptionThis image illustrates the "Street Light" sign, which is typically rectangular. A rectangle is a four-sided polygon with opposite sides that are equal and parallel, and four right angles. In this case the rectangle is rotated to look like a diamond shape. Rectangular signs are commonly used for informational purposes, providing clear and straightforward messages. The symmetry and simplicity of a rectangle make it a practical choice for road signs that convey information about street lighting or other amenities. |
Identifying Shapes |

|
Math Example--Geometric Transformation--Transformations: Example 1 | Math Example--Geometric Transformation--Transformations: Example 1TopicTransformations DescriptionThis example demonstrates a simple translation in geometric transformations. Two "T" shapes are shown on a grid, labeled "Before" and "After". The "Before" shape on the left is translated to become the "After" shape on the right, illustrating how an object can move position without changing its size or orientation. |
Definition of Transformations |
|
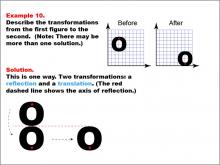
Math Example--Geometric Transformation--Transformations: Example 10 | Math Example--Geometric Transformation--Transformations: Example 10TopicTransformations DescriptionThis example showcases a combination of reflection and translation in geometric transformations. Two circular "O" shapes are displayed on a grid, one labeled "Before" and the other "After". A red dashed line indicates the axis of reflection. The transformation involves first reflecting the shape across this axis and then translating it to a new position. This demonstrates how multiple transformations can be applied in sequence to create more complex movements. |
Definition of Transformations |
|
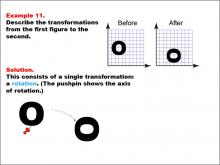
Math Example--Geometric Transformation--Transformations: Example 11 | Math Example--Geometric Transformation--Transformations: Example 11TopicTransformations DescriptionThis example demonstrates a single rotation in geometric transformations. Two circular "O" shapes are shown on a grid, one labeled "Before" and the other "After". The shape undergoes a rotation around a point indicated by a pushpin, which serves as the axis of rotation. This illustrates how an object can change its orientation without altering its size or position relative to the rotation point. |
Definition of Transformations |

|
Math Example--Geometric Transformation--Transformations: Example 12 | Math Example--Geometric Transformation--Transformations: Example 12TopicTransformations DescriptionThis example illustrates a combination of rotation and reflection in geometric transformations. Two circular "O" shapes are displayed on a grid, one labeled "Before" and the other "After". The transformation involves two steps: first, a rotation around a point indicated by a pushpin, which serves as the axis of rotation, followed by a reflection across a red dashed line representing the axis of reflection. This demonstrates how multiple transformations can be applied sequentially to create more complex changes in orientation and position. |
Definition of Transformations |
|
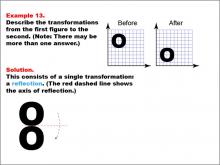
Math Example--Geometric Transformation--Transformations: Example 13 | Math Example--Geometric Transformation--Transformations: Example 13TopicTransformations DescriptionThis example demonstrates a single reflection in geometric transformations. Two grids are shown with the letter "O" before and after a transformation. The "Before" grid has the "O" on the left side, while the "After" grid has it on the right side. A red dashed line indicates the axis of reflection in the middle of the two "O"s. Below, there is a diagram showing the reflection transformation along this axis, illustrating how the shape is flipped across the line while maintaining its size and shape. |
Definition of Transformations |
|
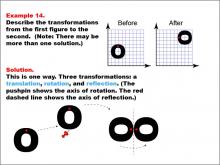
Math Example--Geometric Transformation--Transformations: Example 14 | Math Example--Geometric Transformation--Transformations: Example 14TopicTransformations DescriptionThis example illustrates a complex combination of transformations: translation, rotation, and reflection. Two grids are shown with an "O" shape before and after transformations. The "Before" grid has an "O" with a red dot at its center, while the "After" grid shows the same "O" but rotated and reflected. Below, a step-by-step diagram illustrates three sequential transformations: first, a translation moves the shape, then a rotation turns it around a pushpin (indicating the axis of rotation), and finally, a reflection flips it across a red dashed line. |
Definition of Transformations |
|
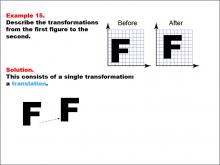
Math Example--Geometric Transformation--Transformations: Example 15 | Math Example--Geometric Transformation--Transformations: Example 15TopicTransformations DescriptionThis example demonstrates a simple translation in geometric transformations. Two grids are displayed with the letter "F" before and after a transformation. The "Before" grid shows an "F" on the left side, and the "After" grid shows it on the right side. Below, there is an arrow indicating that only a translation occurred between the two positions of the letter, showcasing how an object can move position without changing its size or orientation. |
Definition of Transformations |
|
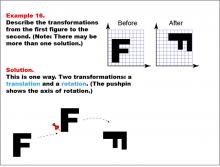
Math Example--Geometric Transformation--Transformations: Example 16 | Math Example--Geometric Transformation--Transformations: Example 16TopicTransformations DescriptionThis example demonstrates a combination of translation and rotation in geometric transformations. Two grids are shown with the letter "F" before and after transformations. The "Before" grid has an upright "F", while the "After" grid shows it rotated 90 degrees clockwise. Below, there is a diagram illustrating two steps: first, a translation moves the shape, then a rotation turns it around a pushpin axis. |
Definition of Transformations |

|
Math Example--Geometric Transformation--Transformations: Example 17 | Math Example--Geometric Transformation--Transformations: Example 17TopicTransformations DescriptionThis example illustrates a combination of reflection and translation in geometric transformations. The image shows a large letter "F" on a grid transforming into a letter "L". The transformation involves two steps: first, a reflection across an axis represented by a red dashed line, and then a translation to a new position. |
Definition of Transformations |
|
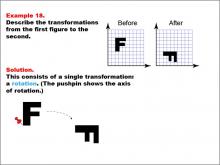
Math Example--Geometric Transformation--Transformations: Example 18 | Math Example--Geometric Transformation--Transformations: Example 18TopicTransformations DescriptionThis example demonstrates a single rotation in geometric transformations. The image shows the letter "F" on a grid being rotated to form the same letter in a different orientation. A pushpin indicates the axis of rotation, and an arrow shows the direction of rotation, illustrating how an object can change its orientation without altering its size or shape. |
Definition of Transformations |
|
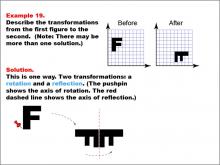
Math Example--Geometric Transformation--Transformations: Example 19 | Math Example--Geometric Transformation--Transformations: Example 19TopicTransformations DescriptionThis example illustrates a combination of rotation and reflection in geometric transformations. The image shows the letter "F" being transformed into an upside-down version of itself. This transformation involves two steps: first, a rotation around an axis indicated by a pushpin, followed by a reflection across a line represented by a red dashed line. |
Definition of Transformations |
|
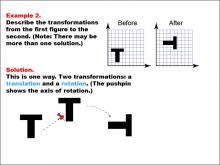
Math Example--Geometric Transformation--Transformations: Example 2 | Math Example--Geometric Transformation--Transformations: Example 2TopicTransformations DescriptionThis example illustrates a combination of transformations: translation and rotation. Two "T" shapes are shown on a grid, labeled "Before" and "After". The shape undergoes a translation (movement) and a rotation, with a pushpin indicating the axis of rotation. This demonstrates how multiple transformations can be applied sequentially to a shape. |
Definition of Transformations |
|
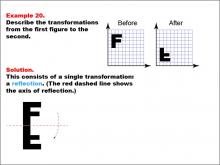
Math Example--Geometric Transformation--Transformations: Example 20 | Math Example--Geometric Transformation--Transformations: Example 20TopicTransformations DescriptionThis example demonstrates a single reflection in geometric transformations. The image shows the letter "F" being reflected to form the letter "L". A red dashed line indicates the axis of reflection, illustrating how the letter changes its orientation through this single transformation. This showcases how an object can be flipped over a line while changing its shape and orientation. |
Definition of Transformations |
|
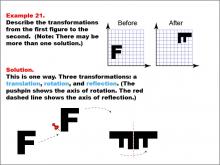
Math Example--Geometric Transformation--Transformations: Example 21 | Math Example--Geometric Transformation--Transformations: Example 21TopicTransformations DescriptionThis example demonstrates a complex combination of transformations: translation, rotation, and reflection. The image shows an uppercase letter "F" on a grid labeled "Before" and a transformed version of the letter "F" upside down on another grid labeled "After." Below, there are illustrations showing the three steps of the transformation process. |
Definition of Transformations |
|
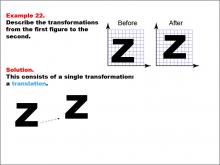
Math Example--Geometric Transformation--Transformations: Example 22 | Math Example--Geometric Transformation--Transformations: Example 22TopicTransformations DescriptionThis example demonstrates a simple translation in geometric transformations. The image shows an uppercase letter "Z" on a grid labeled "Before" and a horizontally shifted version of the same letter on another grid labeled "After." Below, there is an illustration showing just a translation, indicating that the letter "Z" is moved horizontally without any rotation or reflection. |
Definition of Transformations |
|
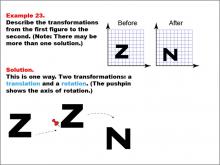
Math Example--Geometric Transformation--Transformations: Example 23 | Math Example--Geometric Transformation--Transformations: Example 23TopicTransformations DescriptionThis example illustrates a combination of translation and rotation in geometric transformations. The image shows an uppercase letter "Z" on a grid labeled "Before" and an uppercase letter "N" on another grid labeled "After." Below, there are illustrations showing the two steps of the transformation: first a translation (moving the figure) followed by a rotation (turning the figure). A pushpin indicates the axis of rotation. |
Definition of Transformations |
|
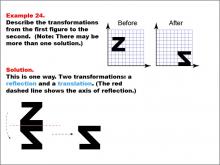
Math Example--Geometric Transformation--Transformations: Example 24 | Math Example--Geometric Transformation--Transformations: Example 24TopicTransformations DescriptionThis example demonstrates a combination of reflection and translation in geometric transformations. The image shows an uppercase letter "Z" on a grid labeled "Before" and a rotated and translated version of the same letter in another position on another grid labeled "After." Below, there are illustrations showing the two steps of the transformation: first, a reflection (flipping the figure across an axis shown by the red dashed line) and then a translation (moving the figure). |
Definition of Transformations |
|
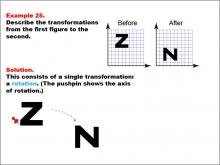
Math Example--Geometric Transformation--Transformations: Example 25 | Math Example--Geometric Transformation--Transformations: Example 25TopicTransformations DescriptionThis example demonstrates a single rotation in geometric transformations. The image shows a transformation of the letter "Z" from an upright position to a rotated position resembling "N". The grid background helps visualize the transformation, and a pushpin icon indicates the axis of rotation, illustrating how an object can change its orientation without altering its size or shape. |
Definition of Transformations |
|
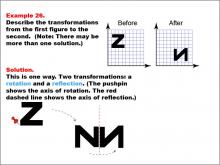
Math Example--Geometric Transformation--Transformations: Example 26 | Math Example--Geometric Transformation--Transformations: Example 26TopicTransformations DescriptionThis example illustrates a combination of rotation and reflection in geometric transformations. The image shows a transformation of the letter "Z" into an upside-down "N". A pushpin indicates the axis of rotation, and a red dashed line represents the axis of reflection. This demonstrates how multiple transformations can be applied sequentially to create more complex changes in orientation and position. |
Definition of Transformations |
|
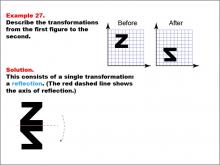
Math Example--Geometric Transformation--Transformations: Example 27 | Math Example--Geometric Transformation--Transformations: Example 27TopicTransformations DescriptionThis example demonstrates a single reflection in geometric transformations. The image shows a reflection of the letter "Z" across a horizontal red dashed line, resulting in a backward "Z". The grid background helps visualize the reflection, illustrating how an object can be flipped over a line while maintaining its size and shape but changing its orientation. |
Definition of Transformations |
|
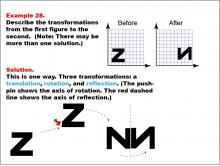
Math Example--Geometric Transformation--Transformations: Example 28 | Math Example--Geometric Transformation--Transformations: Example 28TopicTransformations DescriptionThis example demonstrates a complex combination of transformations: translation, rotation, and reflection. The image shows a transformation of the letter "Z" into an upside-down "N". This transformation involves three steps: first, a translation moves the shape, then a rotation turns it (indicated by a pushpin showing the axis of rotation), and finally, a reflection flips it across a red dashed line representing the axis of reflection. |
Definition of Transformations |
|
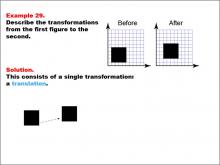
Math Example--Geometric Transformation--Transformations: Example 29 | Math Example--Geometric Transformation--Transformations: Example 29TopicTransformations DescriptionThis example demonstrates a simple translation in geometric transformations. The image shows a "Before" and "After" transformation of a black square on a grid. The square moves horizontally to the right, and a dotted arrow indicates the direction of movement. This showcases how an object can move position without changing its size or orientation. |
Definition of Transformations |
|
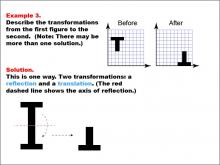
Math Example--Geometric Transformation--Transformations: Example 3 | Math Example--Geometric Transformation--Transformations: Example 3TopicTransformations DescriptionThis example showcases a combination of reflection and translation in geometric transformations. Two "T" shapes are displayed on a grid, labeled "Before" and "After". The shape is first reflected across a red dashed line (the axis of reflection) and then translated to a new position. This demonstrates how multiple transformations can be applied in sequence to create more complex movements. |
Definition of Transformations |
|
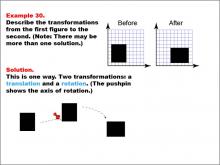
Math Example--Geometric Transformation--Transformations: Example 30 | Math Example--Geometric Transformation--Transformations: Example 30TopicTransformations DescriptionThis example illustrates a combination of translation and rotation in geometric transformations. The image depicts a "Before" and "After" transformation of a black square on a grid. The square moves down and rotates 90 degrees clockwise. A pushpin marks the axis of rotation, and dotted arrows indicate the movement and rotation. This demonstrates how multiple transformations can be applied sequentially to create more complex changes in position and orientation. |
Definition of Transformations |

|
Math Example--Geometric Transformation--Transformations: Example 31 | Math Example--Geometric Transformation--Transformations: Example 31TopicTransformations DescriptionThis example demonstrates a combination of reflection and translation in geometric transformations. The image shows a "Before" and "After" transformation of a black rectangle on a grid. The rectangle first reflects across a horizontal axis, marked by a red dashed line, and then translates horizontally to the right. This illustrates how multiple transformations can be applied sequentially to create more complex changes in position and orientation. |
Definition of Transformations |

|
Math Example--Geometric Transformation--Transformations: Example 32 | Math Example--Geometric Transformation--Transformations: Example 32TopicTransformations DescriptionThis example demonstrates a single rotation in geometric transformations. The image shows a "Before" and "After" transformation of a black rectangle on a grid. The rectangle rotates 90 degrees clockwise around an axis marked by a pushpin. This illustrates how an object can change its orientation without altering its size or shape. |
Definition of Transformations |
|
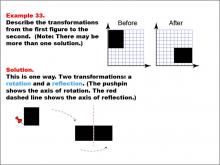
Math Example--Geometric Transformation--Transformations: Example 33 | Math Example--Geometric Transformation--Transformations: Example 33TopicTransformations DescriptionThis example illustrates a combination of rotation and reflection in geometric transformations. The image shows a transformation of a black L-shaped figure on a grid. The "Before" figure is at the top left, and the "After" figure is at the top right. Below, there is a sequence of two transformations: first, a rotation (indicated by a pushpin showing the axis of rotation), followed by a reflection (shown by a red dashed line representing the axis of reflection). |
Definition of Transformations |
|
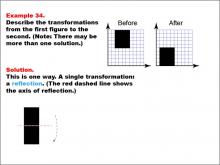
Math Example--Geometric Transformation--Transformations: Example 34 | Math Example--Geometric Transformation--Transformations: Example 34TopicTransformations DescriptionThis example demonstrates a single reflection in geometric transformations. The image shows a black L-shaped figure on a grid. The "Before" figure is at the top left, and the "After" figure is at the top right. Below, there is a single transformation: a reflection across a vertical axis shown with a red dashed line. This showcases how an object can be flipped over a line while maintaining its size and shape but changing its orientation. |
Definition of Transformations |
|
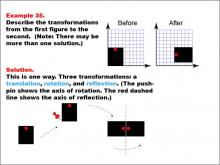
Math Example--Geometric Transformation--Transformations: Example 35 | Math Example--Geometric Transformation--Transformations: Example 35TopicTransformations DescriptionThis example demonstrates a complex combination of transformations: translation, rotation, and reflection. The image shows a black L-shaped figure with a red dot on a grid. The "Before" figure is at the top left, and the "After" figure is at the top right. Below, there are three transformations: first, a translation moves the shape, then a rotation turns it around an axis shown by a pushpin, and finally, a reflection flips it across an axis shown by a red dashed line. |
Definition of Transformations |

|
Math Example--Geometric Transformation--Transformations: Example 36 | Math Example--Geometric Transformation--Transformations: Example 36TopicTransformations DescriptionThis example demonstrates a simple translation in geometric transformations. The image shows two hexagons on grids. The "Before" figure is at the top left, and the "After" figure is at the top right. Below, there is a single transformation: translation, as indicated by an arrow showing movement from one position to another. This showcases how an object can move position without changing its size or orientation. |
Definition of Transformations |
|
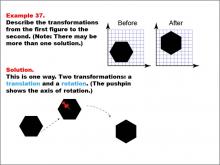
Math Example--Geometric Transformation--Transformations: Example 37 | Math Example--Geometric Transformation--Transformations: Example 37TopicTransformations DescriptionThis example illustrates a combination of translation and rotation in geometric transformations. The image shows a hexagon on a grid, with a "Before" and "After" transformation. The pushpin indicates the axis of rotation, and the hexagon is translated and rotated. This demonstrates how multiple transformations can be applied sequentially to create more complex changes in position and orientation. |
Definition of Transformations |