Digital Resources on the Topic of Linear Equations and Functions
|
|
| Thumbnail Image | Description | |
|---|---|---|
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 5: Ax - By = -C |

|
This is the transcript for the video entitled, Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 5: Ax - By = -C. In this video tutorial, analyze the steps in converting a linear equation in Standard Form to a linear function in Slope-Intercept Form. In this video we work with this version of the Standard Form: Ax - By = -C. |
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 4: -Ax + By = C |

|
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 4: -Ax + By = C
This is the transcript for the video entitled, Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 4: -Ax + By = C. |
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 6: -Ax + By = -C |

|
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 6: -Ax + By = -C
This is the transcript for the video entitled, Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 6: -Ax + By = -C. |
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 7: -Ax - By = C |

|
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 7: -Ax - By = C
This is the transcript for the video entitled, Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 7: -Ax - By = C. |
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 8:-Ax - By = -C |

|
Video Transcript: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 8:-Ax - By = -C
This is the transcript for the video entitled, Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 8:-Ax - By = -C. |
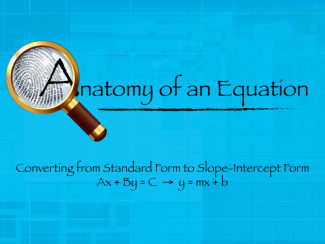
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 1: Ax + By = C |
|
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 1: Ax + By = C
|
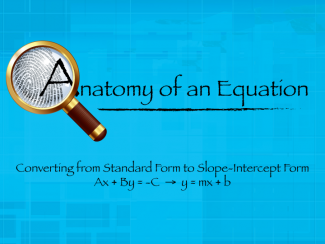
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 2: Ax + By = -C |
|
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 2: Ax + By = -C
|
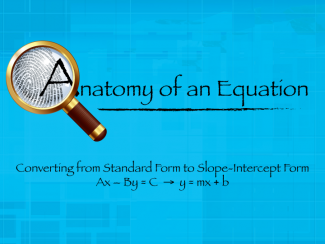
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 3: Ax - By = C |
|
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 3: Ax - By = C
|
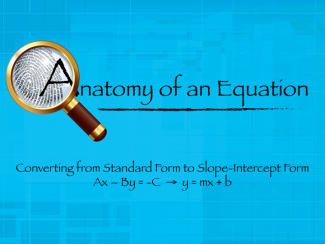
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 5: Ax - By = -C |
|
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 4: -Ax + By = C
|
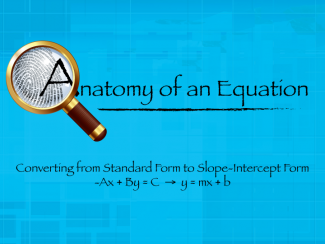
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 4: -Ax + By = C |
|
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 5: Ax - By = -C
|
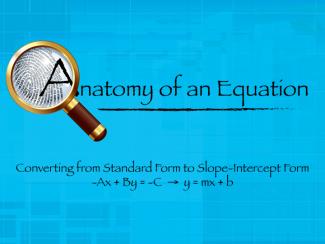
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 6: -Ax + By = -C |
|
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 6: -Ax + By = -C
|
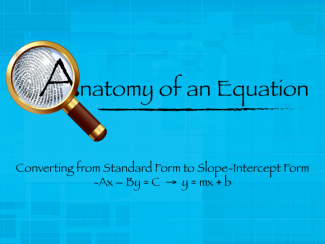
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 7: -Ax - By = C |
|
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 7: -Ax - By = C
|
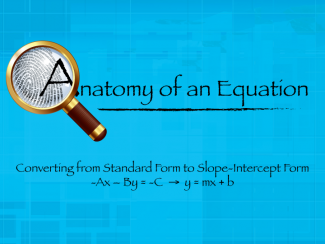
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 8:-Ax - By = -C |
|
Video Tutorial: Anatomy of an Equation: Linear Equations in Standard Form to Slope-Intercept Form 8:-Ax - By = -C
|
Desmos Activity: Linear Functions 1: y = mx |

|
In this graphing calculator activity, have your students explore linear functions of the form y = mx. This Desmos template allows students to explore the effect of changes in the slope have on the graph of the line. A companion downloadable worksheet uses the graphing calculator template to explore the properties of these linear functions. Note: The download is a PDF worksheet. |
Closed Captioned Video: Exp the Slope-Intercept form |

|
Closed Captioned Video: Exp the Slope-Intercept form
In this TI Nspire tutorial, the Graph window is used to create a slider-based graph of a linear function in slope-intercept form. This video supports the TI-Nspire Clickpad and Touchpad. This Mini-Tutorial Video includes a worksheet. . |
Closed Captioned Video: Linear Function |

|
Closed Captioned Video: Linear Function
In this TI Nspire tutorial, the Graph window is used to graph a linear function. This video supports the TI-Nspire Clickpad and Touchpad. This Mini-Tutorial Video includes a worksheet. |
Video Transcript: TI-Nspire Mini-Tutorial: Exploring the Slope-Intercept form Using Sliders |

|
Video Transcript: TI-Nspire Mini-Tutorial: Exploring the Slope-Intercept form Using Sliders
This is the transcript for the TI-Nspire Mini-Tutorial entitled, Exploring the Slope-Intercept form Using Sliders. |
Video Transcript: TI-Nspire Mini-Tutorial: Graphing a Linear Function |

|
Video Transcript: TI-Nspire Mini-Tutorial: Graphing a Linear Function
This is the transcript for the TI-Nspire Mini-Tutorial entitled, Graphing a Linear Function. |
Closed Captioned Video: Algebra Nspirations: Linear Functions, 3 |

|
Closed Captioned Video: Algebra Nspirations: Linear Functions, Segment 3
In this Investigation we look at a linear regression for carbon dioxide emission data. This video is Segment 3 of a 4 segment series related to Algebra Nspirations: Linear Functions. Segments 3 and 4 are grouped together. |
Closed Captioned Video: Algebra Nspirations: Linear Functions, 1 |

|
Closed Captioned Video: Algebra Nspirations: Linear Functions, Segment 1
In this Investigation we look at linear models for objects moving at a constant speed. This video is Segment 1 of a 4 segment series related to Algebra Nspirations: Linear Functions. Segments 1 and 2 are grouped together. |
Closed Captioned Video: Algebra Nspirations: Linear Functions |

|
Closed Captioned Video: Algebra Nspirations: Linear Functions
In this program, internationally acclaimed mathematics educator Dr. Monica Neagoy, explores the nature of linear functions through the use TI graphing calculators. |
Closed Captioned Video: Algebra Nspirations: Functions and Relations, 3 |

|
Closed Captioned Video: Algebra Nspirations: Functions and Relations, Segment 3
In this Investigation we look at functions. This video is Segment 3 of a 4 segment series related to Functions and Relations. Segments 3 and 4 are grouped together. |
Closed Captioned Video: Algebra Nspirations: Functions and Relations, 1 |

|
Closed Captioned Video: Algebra Nspirations: Functions and Relations, Segment 1
In this Investigation we explore the definition of a Relation. This video is Segment 1 of a 4 segment series related to Functions and Relations. Segments 1 and 2 are grouped together. |
Closed Captioned Video: Algebra Nspirations: Functions and Relations |

|
Closed Captioned Video: Algebra Nspirations: Functions and Relations
Functions are relationships between quantities that change. Written and hosted by internationally acclaimed math educator Dr. Monica Neagoy, this video explores the definition of a function, its vocabulary and notations, and distinguishes the concept of function from a general relation. |
Closed Captioned Video: Algebra Applications: Linear Functions, 4 |

|
Closed Captioned Video: Algebra Applications: Linear Functions, 4 |

