Lesson Plan: Introduction to Linear Functions 

Lesson Objectives

  • Define linear functions
  • Identify linear functions from equations and graphs
  • Understand the concept of slope

Florida BEST Standards

  • MA.912.F.1.1: Classify functions as linear, quadratic, cubic, exponential, logarithmic, rational, or absolute value.
  • MA.912.F.1.2: Determine key features of function graphs including extrema, end behavior, intercepts, domain, and range.
  • MA.912.F.1.3: Calculate and interpret average rate of change in various representations.
  • MA.912.F.1.8: Determine the best function model for a real-world situation.
  • MA.912.F.2.1: Identify effects of transformations on function graphs.
  • MA.912.AR.2.1: Write and solve one-variable linear equations in context.
  • MA.912.AR.2.3: Write linear two-variable equations from various representations.
  • MA.912.AR.2.4: Graph linear functions and determine key features.

Prerequisite Skills

  • Basic algebraic operations
  • Plotting points on the Cartesian coordinate plane

Key Vocabulary

  • Linear function
  • Slope
  • Y-intercept
  • Constant rate of change

Warm-up Activity (10 minutes)

Introduce the concept of linear functions by showing real-life examples. Use this slide show:

https://www.media4math.com/library/slideshow/applications-linear-equations

Or, use one of these these slide shows, which show a more detailed explanation of these real-world applications of linear functions.

Teach (25 minutes)

Definitions

Define linear functions as relationships where the rate of change between variables is constant. Review these definitions:

 If necessary, review the definition of slope with this video definition:

 Examples

Use this slide show to review the properties of linear functions before showing examples of graphs of linear functions:

https://www.media4math.com/library/slideshow/graphs-linear-functions

Use this slide show to demonstrate examples of graphs of linear functions given the slope and y-intercept:

https://www.media4math.com/library/slideshow/math-examples-slope-intercept-form

Use this Desmos activity to explore graphs of linear functions in slope-intercept form:

https://www.media4math.com/library/40088/asset-preview

 Here is a worksheet to accompany this activity:

https://www.media4math.com/library/40089/asset-preview

Review (5 minutes)

Use this drag-and-drop activity to review linear functions in slope-intercept form. Match the equation to the description:

https://www.media4math.com/library/4829/asset-preview

Assess (10 minutes)

Administer a 10-question quiz to assess understanding of linear functions, slope, and graphing.

Quiz

  1. Which equation represents a linear function?
    a) y = 2x + 3 
    b) y = x² 
    c) y = 3ˣ 
    d) y = 1/x

     
  2. What is the slope of the line y = -4x + 7?

     
  3. Identify the y-intercept of the function f(x) = 3x - 5.

     
  4. Graph the linear function y = 2x - 1.
    Coordinate Grid

     
  5. Is the function y = √x linear? Explain why or why not.

     
  6. Find the slope of the line passing through points (2, 5) and (4, 9).

     
  7. Write the equation of a line with slope 3 and y-intercept -2.

     
  8. Determine if the following table represents a linear function:
    x | 1 | 2 | 3 | 4
    y | 3 | 5 | 7 | 9

     
  9. What is the slope of a horizontal line?

     
  10. Sketch a graph of a linear function with a negative slope and positive y-intercept.
    Coordinate Grid

     

Answer Key

  1. a
  2. -4
  3. -5
  4. Coordinate Grid
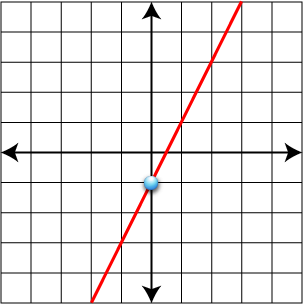
  5. No, because it is not in slope-intercept or standard form.
  6. 2
  7. y = 3x - 2
  8. Yes, the rate of change is constant (2)
  9. 0
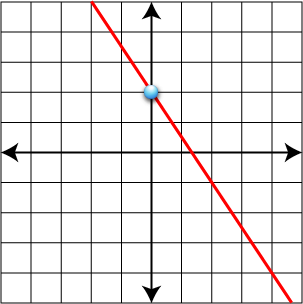
  10. Check that student graph shows a downward sloping line crossing the positive y-axis. Here is an example:
    Coordinate Graph

 

Purchase the lesson plan bundle. Click here.