
Illustrative Math Alignment: Grade 6 Unit 1
Reasoning to Find Area
Lesson 3: Comparing Regions
Use the following Media4Math resources with this Illustrative Math lesson.
| Thumbnail Image | Title | Body | Curriculum Nodes |
|---|---|---|---|
|
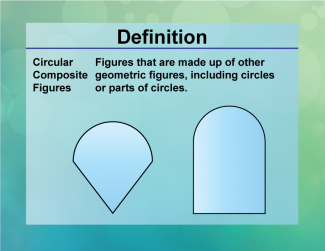
Definition--Circle Concepts--Circular Composite Figures |
Circular Composite FiguresTopicCircles DefinitionCircular composite figures are shapes that include circles or parts of circles combined with other geometric figures. |
Definition of a Circle |
|
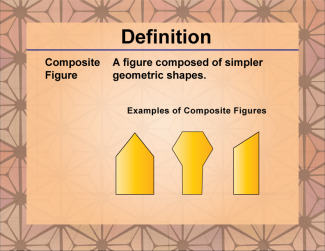
Definition--Polygon Concepts--Composite Figure |
Compound FigureTopicPolygons DefinitionA compound figure is a geometric shape that is composed of two or more simple polygons combined to form a more complex figure. DescriptionIn geometry, a compound figure is a shape that is formed by combining two or more polygons. These figures are significant because they allow for the exploration of more complex shapes and their properties, which can be broken down into simpler, more manageable components. Understanding compound figures is essential for solving problems related to area, perimeter, and other geometric properties. |
Definition of a Polygon |
|
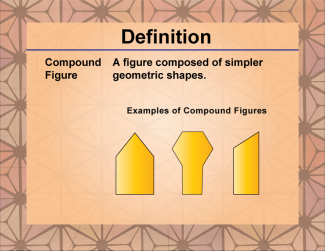
Definition--Polygon Concepts--Compound Figure |
Compound FigureTopicPolygons DefinitionA polygon is a closed two-dimensional shape with straight line segments, while a compound figure is a shape formed by combining two or more geometric figures. |
Definition of a Polygon |
|
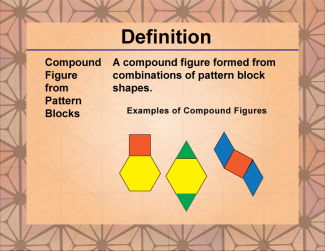
Definition--Polygon Concepts--Compound Figure from Pattern Blocks |
Compound Figure from Pattern BlocksTopicPolygons DefinitionA compound figure, also known as a composite figure, is a shape that is made up of two or more simple geometric figures such as triangles, rectangles, squares, parallelograms, trapezoids, and circles. |
Definition of a Polygon |
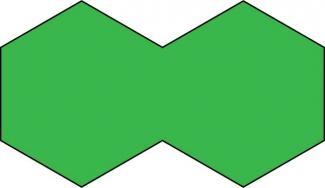
|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 1 |
Math Clip Art--Composite Figures--Composite Figure 1TopicGeometry Concepts DescriptionThis image depicts a composite figure consisting of a square and a triangle. Composite figures are essential in geometry as they allow students to apply area and perimeter formulas to more complex shapes. To calculate the total area of this figure, we would add the area of the square (s²) to the area of the triangle (½bh). The perimeter would involve adding the lengths of all outer sides. This combination of shapes is common in architecture and design, demonstrating how basic geometric shapes can be combined to create more complex structures. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 1 |
Math Clip Art--Composite Figures--Composite Figure 1TopicGeometry Concepts DescriptionThis image depicts a composite figure consisting of a square and a triangle. Composite figures are essential in geometry as they allow students to apply area and perimeter formulas to more complex shapes. To calculate the total area of this figure, we would add the area of the square (s²) to the area of the triangle (½bh). The perimeter would involve adding the lengths of all outer sides. This combination of shapes is common in architecture and design, demonstrating how basic geometric shapes can be combined to create more complex structures. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 2 |
TopicGeometry Concepts DescriptionThis image illustrates a composite figure composed of one rectangle and one semicircle. This combination of shapes is often seen in architectural designs and mechanical parts. To calculate the total area, we would add the areas of the rectangle (l * w) and the semicircle (πr² / 2, where r is half the width of the rectangle). The perimeter calculation would involve the straight edges of the rectangle and the curved edge of the semicircle. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 2 |
TopicGeometry Concepts DescriptionThis image illustrates a composite figure composed of one rectangle and one semicircle. This combination of shapes is often seen in architectural designs and mechanical parts. To calculate the total area, we would add the areas of the rectangle (l * w) and the semicircle (πr² / 2, where r is half the width of the rectangle). The perimeter calculation would involve the straight edges of the rectangle and the curved edge of the semicircle. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 3 |
Math Clip Art--Composite Figures--Composite Figure 3TopicGeometry Concepts DescriptionThis image illustrates a composite figure consisting of a semicircle and a triangle. Each of these shapes is a unique component, combining to create a more complex geometric form. To calculate the total area of this composite figure, we would add the area of the semicircle (πr²/2) to the area of the triangle (½bh). The perimeter calculation would involve the circumference of the semicircle plus the lengths of the triangle's sides that are not overlapping with the semicircle. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 3 |
Math Clip Art--Composite Figures--Composite Figure 3TopicGeometry Concepts DescriptionThis image illustrates a composite figure consisting of a semicircle and a triangle. Each of these shapes is a unique component, combining to create a more complex geometric form. To calculate the total area of this composite figure, we would add the area of the semicircle (πr²/2) to the area of the triangle (½bh). The perimeter calculation would involve the circumference of the semicircle plus the lengths of the triangle's sides that are not overlapping with the semicircle. |
Applications of Polygons |
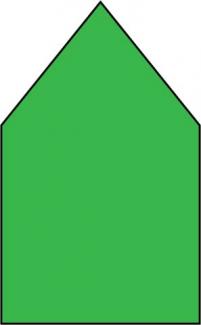
|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 4 |
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 4TopicGeometry Concepts DescriptionThis image depicts a composite figure consisting of a regular pentagon and a square. This combination of shapes demonstrates how different polygons can be combined to create more complex geometric figures. To calculate the total area of this composite figure, we would add the area of the pentagon (given by the formula A = ¼√(25+10√5)s², where s is the side length) to the area of the square (s²). The perimeter would involve summing the lengths of all outer sides of both shapes that are not shared. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 4 |
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 4TopicGeometry Concepts DescriptionThis image depicts a composite figure consisting of a regular pentagon and a square. This combination of shapes demonstrates how different polygons can be combined to create more complex geometric figures. To calculate the total area of this composite figure, we would add the area of the pentagon (given by the formula A = ¼√(25+10√5)s², where s is the side length) to the area of the square (s²). The perimeter would involve summing the lengths of all outer sides of both shapes that are not shared. |
Applications of Polygons |
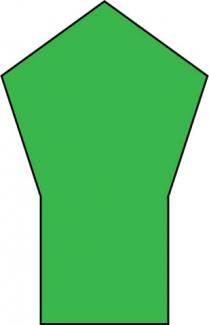
|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 5 |
Math Clip Art--Composite Figures--Composite Figure 5TopicGeometry Concepts DescriptionThis image illustrates a composite figure composed of two rectangles that form an L-shape. This configuration is commonly seen in floor plans, furniture design, and architectural layouts. To calculate the total area of this L-shaped figure, we would add the areas of the two rectangles (l * w for each). The perimeter would involve summing the lengths of all outer sides, being careful not to double-count the shared edge. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 5 |
Math Clip Art--Composite Figures--Composite Figure 5TopicGeometry Concepts DescriptionThis image illustrates a composite figure composed of two rectangles that form an L-shape. This configuration is commonly seen in floor plans, furniture design, and architectural layouts. To calculate the total area of this L-shaped figure, we would add the areas of the two rectangles (l * w for each). The perimeter would involve summing the lengths of all outer sides, being careful not to double-count the shared edge. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 6 |
Math Clip Art--Composite Figures--Composite Figure 6TopicGeometry Concepts DescriptionThis image shows a composite figure made up of two overlapping rectangles that form a cross shape. This type of configuration is often seen in architectural designs, religious symbols, and logo designs. To find the total area of this cross-shaped figure, we would add the areas of the two rectangles (l * w for each) and then subtract the area of the overlapping region to avoid double-counting. The perimeter calculation would involve summing the lengths of all outer edges of the cross shape. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 6 |
Math Clip Art--Composite Figures--Composite Figure 6TopicGeometry Concepts DescriptionThis image shows a composite figure made up of two overlapping rectangles that form a cross shape. This type of configuration is often seen in architectural designs, religious symbols, and logo designs. To find the total area of this cross-shaped figure, we would add the areas of the two rectangles (l * w for each) and then subtract the area of the overlapping region to avoid double-counting. The perimeter calculation would involve summing the lengths of all outer edges of the cross shape. |
Applications of Polygons |
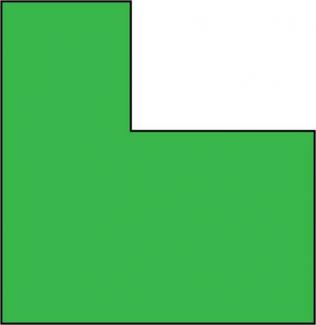
|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 7 |
Math Clip Art--Composite Figures--Composite Figure 7TopicGeometry Concepts DescriptionThis image depicts a composite figure consisting of two adjacent regular hexagons. This configuration is often found in nature, such as in honeycomb structures, and is used in various design and engineering applications. To calculate the total area of this figure, we would add the areas of the two hexagons (given by the formula A = (3√3 * s²) / 2, where s is the side length). The perimeter would involve summing the lengths of all outer sides, excluding the shared edge between the hexagons. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 7 |
Math Clip Art--Composite Figures--Composite Figure 7TopicGeometry Concepts DescriptionThis image depicts a composite figure consisting of two adjacent regular hexagons. This configuration is often found in nature, such as in honeycomb structures, and is used in various design and engineering applications. To calculate the total area of this figure, we would add the areas of the two hexagons (given by the formula A = (3√3 * s²) / 2, where s is the side length). The perimeter would involve summing the lengths of all outer sides, excluding the shared edge between the hexagons. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 8 |
Math Clip Art--Composite Figures--Composite Figure 8TopicGeometry Concepts DescriptionThis image illustrates a composite figure composed of two regular hexagons connected by a square. This unique configuration combines the properties of hexagons and squares in an interesting geometric arrangement. To calculate the total area of this figure, we would add the areas of the two hexagons (A = (3√3 * s²) / 2 for each, where s is the side length) and the area of the connecting square (s²). The perimeter would involve summing the lengths of all outer sides of the figure. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 8 |
Math Clip Art--Composite Figures--Composite Figure 8TopicGeometry Concepts DescriptionThis image illustrates a composite figure composed of two regular hexagons connected by a square. This unique configuration combines the properties of hexagons and squares in an interesting geometric arrangement. To calculate the total area of this figure, we would add the areas of the two hexagons (A = (3√3 * s²) / 2 for each, where s is the side length) and the area of the connecting square (s²). The perimeter would involve summing the lengths of all outer sides of the figure. |
Applications of Polygons |
|
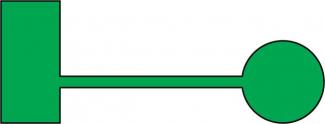
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 9 |
Math Clip Art--Composite Figures--Composite Figure 9TopicGeometry Concepts DescriptionThis image shows a composite figure made up of a rectangle and a circle connected by a long, thin rectangle. This configuration might represent various real-world objects, such as a lollipop, a simplified key, or certain types of tools or instruments. To find the total area of this figure, we would add the areas of the rectangle (l * w), the circle (πr²), and the connecting thin rectangle. The perimeter calculation would involve the circumference of the circle and the outer edges of the rectangles, excluding any parts where they connect. |
Applications of Polygons |

|
Math Clip Art--Geometry Concepts--Composite Figures--Composite Figure 9 |
Math Clip Art--Composite Figures--Composite Figure 9TopicGeometry Concepts DescriptionThis image shows a composite figure made up of a rectangle and a circle connected by a long, thin rectangle. This configuration might represent various real-world objects, such as a lollipop, a simplified key, or certain types of tools or instruments. To find the total area of this figure, we would add the areas of the rectangle (l * w), the circle (πr²), and the connecting thin rectangle. The perimeter calculation would involve the circumference of the circle and the outer edges of the rectangles, excluding any parts where they connect. |
Applications of Polygons |
|
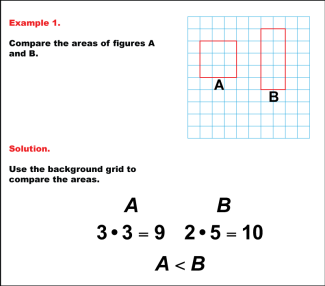
Math Example: Comparing Area: Example 1 |
Math Example: Comparing Area: Example 1TopicGeometry DescriptionThis example compares the areas of two rectangles labeled A and B on a grid. Rectangle A measures 3 units by 3 units, while Rectangle B is 2 units by 5 units. The area of Rectangle A is calculated as 3 * 3 = 9 square units, and the area of Rectangle B is 2 * 5 = 10 square units. By comparing these areas, we can conclude that A < B, meaning Rectangle B has a larger area than Rectangle A. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |
|
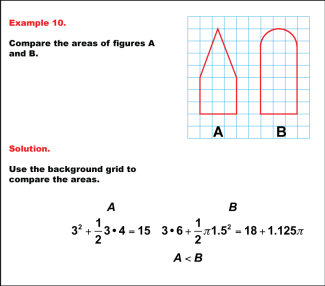
Math Example: Comparing Area: Example 10 |
Math Example: Comparing Area: Example 10TopicGeometry DescriptionThis example compares the areas of two complex shapes (Figures A and B) drawn on a grid. Figure A is a composite of a square with a triangular top, while Figure B is a semicircle on top of a rectangle. Calculating their areas, we find that Figure A has an area of 32 + (1/2) * 3 * 4 = 15 square units, and Figure B has an area of 3 * 6 + (1/2) * π * 1.52 = 18 + 1.125π square units. Comparing these results, we can conclude that A < B, meaning Figure B has a larger area than Figure A. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |
|
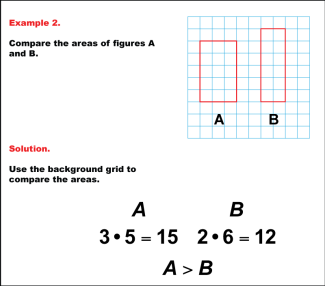
Math Example: Comparing Area: Example 2 |
Math Example: Comparing Area: Example 2TopicGeometry DescriptionIn this example, we compare the areas of two rectangles, A and B, drawn on a grid. Rectangle A has dimensions of 3 units by 5 units, while Rectangle B measures 2 units by 6 units. Calculating their areas, we find that Rectangle A has an area of 3 * 5 = 15 square units, and Rectangle B has an area of 2 * 6 = 12 square units. Comparing these results, we can conclude that A > B, meaning Rectangle A has a larger area than Rectangle B. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |

|
Math Example: Comparing Area: Example 3 |
Math Example: Comparing Area: Example 3TopicGeometry DescriptionThis example presents two rectangles, A and B, drawn on a grid for area comparison. Rectangle A measures 4 units by 5 units, while Rectangle B is 3 units by 6 units. Calculating their areas, we find that Rectangle A has an area of 4 * 5 = 20 square units, and Rectangle B has an area of 3 * 6 = 18 square units. Comparing these results, we can conclude that A > B, meaning Rectangle A has a larger area than Rectangle B. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |
|
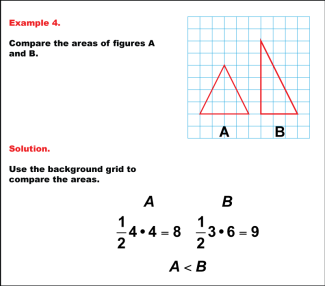
Math Example: Comparing Area: Example 4 |
Math Example: Comparing Area: Example 4TopicGeometry DescriptionThis example compares the areas of two triangles, A and B, drawn on a grid. Triangle A has a base of 4 units and a height of 4 units, while Triangle B has a base of 3 units and a height of 6 units. Using the formula for the area of a triangle (1/2 * base * height), we calculate that Triangle A has an area of (1/2) * 4 * 4 = 8 square units, and Triangle B has an area of (1/2) * 3 * 6 = 9 square units. Comparing these results, we can conclude that A < B, meaning Triangle B has a larger area than Triangle A. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |
|
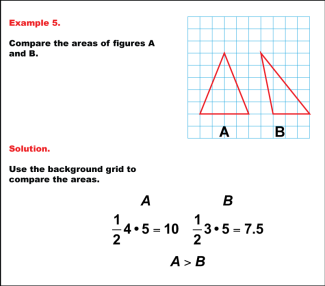
Math Example: Comparing Area: Example 5 |
Math Example: Comparing Area: Example 5TopicGeometry DescriptionThis example compares the areas of two triangles, A and B, nested within a grid. Triangle A has a base of 4 units and a height of 5 units, while Triangle B has a base of 3 units and a height of 5 units. Using the formula for the area of a triangle (1/2 * base * height), we calculate that Triangle A has an area of 1/2 * 4 * 5 = 10 square units, and Triangle B has an area of 1/2 * 3 * 5 = 7.5 square units. Comparing these results, we can conclude that A > B, meaning Triangle A has a larger area than Triangle B. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |
|
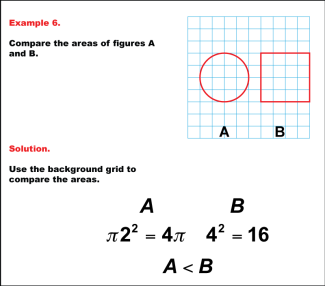
Math Example: Comparing Area: Example 6 |
Math Example: Comparing Area: Example 6TopicGeometry DescriptionThis example compares the areas of a circle (Figure A) and a square (Figure B) drawn on a grid. The circle has a radius of 2 units, while the square has a side length of 4 units. Using the formula for the area of a circle (π r2) and a square (s2), we calculate that the circle has an area of π * 22 = 4π square units, and the square has an area of 42 = 16 square units. Comparing these results, we can conclude that A < B, meaning the square has a larger area than the circle. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |
|
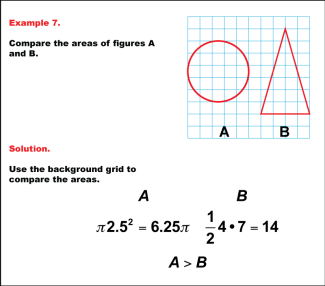
Math Example: Comparing Area: Example 7 |
Math Example: Comparing Area: Example 7TopicGeometry DescriptionThis example compares the areas of a circle (Figure A) and a triangle (Figure B) drawn on a grid. The circle has a radius of 2.5 units, while the triangle has a base of 4 units and a height of 7 units. Using the formula for the area of a circle (π r2) and a triangle (1/2 * base * height), we calculate that the circle has an area of π * 2.52 = 6.25π square units, and the triangle has an area of 1/2 * 4 * 7 = 14 square units. Comparing these results, we can conclude that A > B, meaning the circle has a larger area than the triangle. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |
|
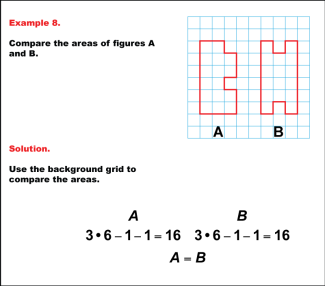
Math Example: Comparing Area: Example 8 |
Math Example: Comparing Area: Example 8TopicGeometry DescriptionThis example compares the areas of two complex shapes (Figures A and B) made up of rectangles, displayed on a grid. Both figures have identical areas calculated as 3 * 6 - 1 - 1 = 16 square units. By comparing these results, we can conclude that A = B, meaning both shapes have equal areas despite their different appearances. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |
|
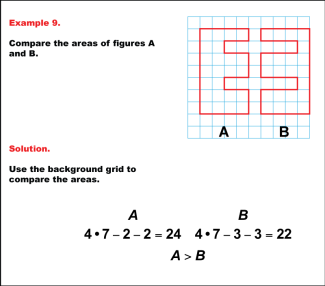
Math Example: Comparing Area: Example 9 |
Math Example: Comparing Area: Example 9TopicGeometry DescriptionThis example compares the areas of two complex shapes (Figures A and B) drawn on a grid. Figure A is a shape with multiple right angles, while Figure B is similar but slightly smaller. Calculating their areas, we find that Figure A has an area of 4 * 7 - 2 - 2 = 24 square units, and Figure B has an area of 4 * 7 - 3 - 3 = 22 square units. Comparing these results, we can conclude that A > B, meaning Figure A has a larger area than Figure B. |
Area and Perimeter of Quadrilaterals, Area and Perimeter of Triangles and Area and Circumference |

|
Video Transcript: Geometry Applications: Polygons, Segment 3: Composite Figures |
Video Transcript: Geometry Applications: Polygons, Segment 3: Composite Figures This is the transcript for the video of same title. Video contents: The Petronas Towers in Indonesia provide an opportunity to explore the composite shapes used in the design of the towers. This is part of a collection of video transcript from the Geometry Applications video series. To see the complete collection of transcripts, click on this link.Note: The download is a PDF file.Video Transcript LibraryTo see the complete collection of video transcriptsy, click on this link. |
Applications of Polygons |